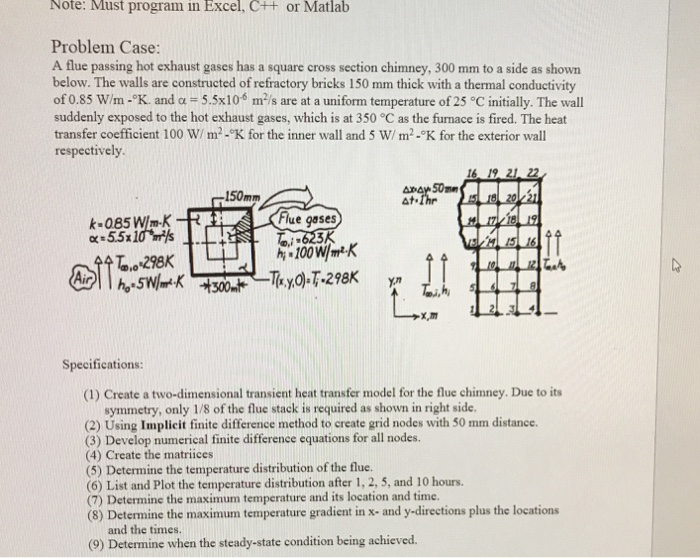
Note: Must program in Excel, C++ or Matlab Problem Case: A flue passing hot exhaust gases has a square cross section chimney, 300 mm to a side as shown below. The walls are constructed of refractory bricks 150 mm thick with a thermal conductivity of 0.85 wm-oK. and -55x106 mrs are at a uniform temperature of 25C nitially. The wall suddenly exposed to the hot exhaust gases, which is at 350 C as the funace is fired. The heat transfer coefficient 100 W/ m2-K for the inner wall and 5 W/m2-K for the exterior wall respectively. 50mm Flue gases T y.coote98K r llE h -100W/mK eIthe529 KK X,r Specifications: (1) Create a two-dimensional transient heat transfer model for the flue chimney. Due to its symmetry, only 1/8 of the flue stack is required as shown in right side. (2) Using Implicit finite difference method to create grid nodes with 50 mm distance. (3) Develop numerical finite difference equations for all nodes (4) Create the matriices (5) Determine the temperature distribution of the flue. (6) List and Plot the temperature distribution after 1, 2, 5, and 10 hours. (7) Determine the maximum temperature and its location and time (8) Determine the maximum temperature gradient in x- and y direcetions plus the locations and the times. (9) Determine when the steady-state condition being achieved. Note: Must program in Excel, C++ or Matlab Problem Case: A flue passing hot exhaust gases has a square cross section chimney, 300 mm to a side as shown below. The walls are constructed of refractory bricks 150 mm thick with a thermal conductivity of 0.85 wm-oK. and -55x106 mrs are at a uniform temperature of 25C nitially. The wall suddenly exposed to the hot exhaust gases, which is at 350 C as the funace is fired. The heat transfer coefficient 100 W/ m2-K for the inner wall and 5 W/m2-K for the exterior wall respectively. 50mm Flue gases T y.coote98K r llE h -100W/mK eIthe529 KK X,r Specifications: (1) Create a two-dimensional transient heat transfer model for the flue chimney. Due to its symmetry, only 1/8 of the flue stack is required as shown in right side. (2) Using Implicit finite difference method to create grid nodes with 50 mm distance. (3) Develop numerical finite difference equations for all nodes (4) Create the matriices (5) Determine the temperature distribution of the flue. (6) List and Plot the temperature distribution after 1, 2, 5, and 10 hours. (7) Determine the maximum temperature and its location and time (8) Determine the maximum temperature gradient in x- and y direcetions plus the locations and the times. (9) Determine when the steady-state condition being achieved