NOTE: Please, include all the calculations, m$-$files and Simulink models, plots and graphics that you produced on your own. In addition, provide detailed analyses for each plot t in your report.Thanks

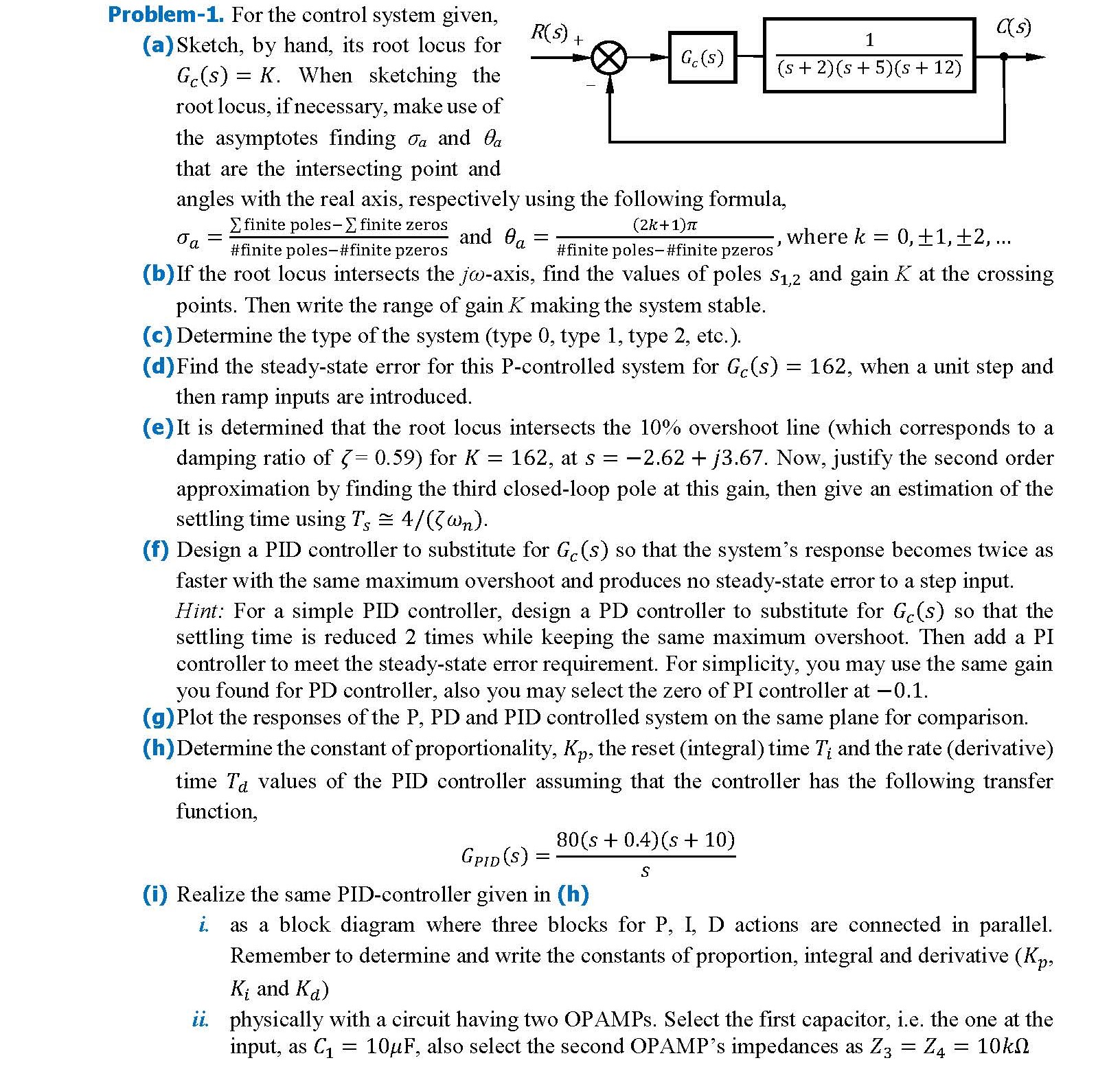
Problem$-1.$ For the control system given,

$($a$)$ Sketch, by hand, its root locus for

$G_c(s)=K.$ When sketching the

root locus, if necessary, make use of

the asymptotes finding ${}_a$ and ${}_a$

that are the intersecting point and

angles with the real axis, respectively using the following formula,

${}_a=\frac{{\displaystyle \underset{?}{\overset{?}{}}}\text{f}\text{i}\text{n}\text{i}\text{t}\text{e}\text{p}\text{o}\text{l}\text{e}\text{s}-{\displaystyle \underset{?}{\overset{?}{}}}\text{f}\text{i}\text{n}\text{i}\text{t}\text{e}\text{z}\text{e}\text{r}\text{o}\text{s}}{\text{\#}\text{f}\text{i}\text{n}\text{i}\text{t}\text{e}\text{p}\text{o}\text{l}\text{e}\text{s}\frac{-}{\text{\#}}\text{f}\text{i}\text{n}\text{i}\text{t}\text{e}\text{p}\text{z}\text{e}\text{r}\text{o}\text{s}}$ and ${}_a=\frac{(2k+1)}{\text{\#}\text{f}\text{i}\text{n}\text{i}\text{t}\text{e}\text{p}\text{o}\text{l}\text{e}\text{s}\frac{-}{\text{\#}}\text{f}\text{i}\text{n}\text{i}\text{t}\text{e}\text{p}\text{z}\text{e}\text{r}\text{o}\text{s}},$ where $k=0,+-1,+-2,$dots

$($b$)$ If the root locus intersects the $j-$axis, find the values of poles $s_{1,2}$ and gain $K$ at the crossing

points. Then write the range of gain $K$ making the system stable.

$($c$)$ Determine the type of the system $($type $0,$ type $1,$ type $2,$ etc.$).$

$($d$)$Find the steady$-$state error for this P$-$controlled system for $G_C(s)=162,$ when a unit step and

then ramp inputs are introduced.

$($e$)$ It is determined that the root locus intersects the $10\%$ overshoot line $($which corresponds to a

damping ratio of $=0\mathrm{.}59)$ for $K=162,$ at $s=-2\mathrm{.}62+j3\mathrm{.}67.$ Now, justify the second order

approximation by finding the third closed$-$loop pole at this gain, then give an estimation of the

settling time using $T_s$~$=\frac{4}{{}_n}.$

$($f$)$ Design a PID controller to substitute for $G_C(s)$ so that the system's response becomes twice as

faster with the same maximum overshoot and produces no steady$-$state error to a step input.

Hint: For a simple PID controller, design a PD controller to substitute for $G_c(s)$ so that the

settling time is reduced $2$ times while keeping the same maximum overshoot. Then add a PI

controller to meet the steady$-$state error requirement. For simplicity, you may use the same gain

you found for PD controller, also you may select the zero of PI controller at $-0\mathrm{.}1.$

$($g$)$Plot the responses of the P$,$ PD and PID controlled system on the same plane for comparison.

$($h$)$ Determine the constant of proportionality, $K_p,$ the reset $($integral$)$ time $T_i$ and the rate $($derivative$)$

time $T_d$ values of the PID controller assuming that the controller has the following transfer

function,

$G_{\frac{?}{PI}D}(s)=\frac{80(s+0\mathrm{.}4)(s+10)}{s}$

$($i$)$ Realize the same PID$-$controller given in $($h$)$

i$.$ as a block diagram where three blocks for P$,$ I, D actions are connected in parallel.

Remember to determine and write the constants of proportion, integral and derivative $,$

$K_i$ and $K_d)$

ii$.$ physically with a circuit having two OPAMPs. Select the first capacitor, i$.$e$.$ the one at the

input, as $C_1=10F,$ also select the second OPAMP's impedances as $Z_3=Z_4=10k$