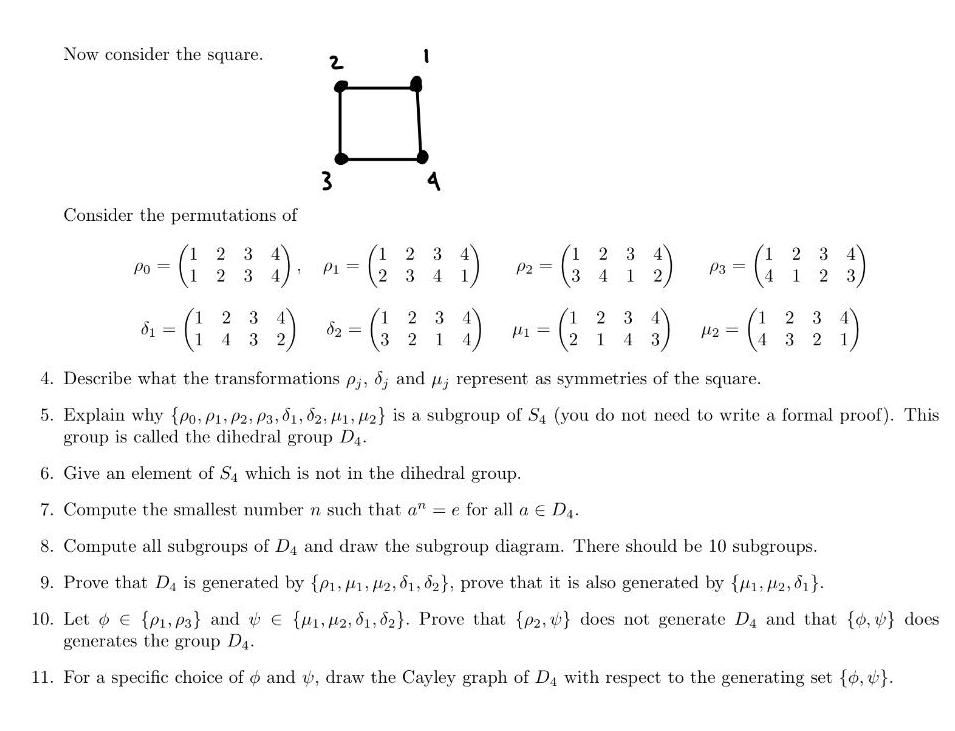
Now consider the square. Consider the permutations of 1 2 3 4 34 Po = 8 = ( 1 2 23 G 2) 4 3 2 3 P1 82 = = 4 1 2 3 2 3 4 (3 1) 4) 2 3 4 21 P2 fl1 = 1 2 3 4 3 4 12 1 2 2 3 4 143 P3 142 = 14 4 2 3 23 32 1 3 3 2 3 1) 4. Describe what the transformations pj, 6, and , represent as symmetries of the square. 5. Explain why {po, P1, P2, P3, 81, 82, M1, 2} is a subgroup of S4 (you do not need to write a formal proof). This group is called the dihedral group D4- 6. Give an element of S4 which is not in the dihedral group. 7. Compute the smallest number n such that an = e for all a D. 8. Compute all subgroups of D4 and draw the subgroup diagram. There should be 10 subgroups. 9. Prove that D4 is generated by {P1, 1, 2, 81, 82}, prove that it is also generated by {, , 8}. 10. Let {P1, P3} and {M1, M2, 81, 82). Prove that {p2, } does not generate D4 and that {o, } does enerates the group D4. 11. For a specific choice of and , draw the Cayley graph of D with respect to the generating set {o, }.