Question: Obtain the temperature distribution as a function of (x, y, t) T 1 T + ax dy a t Where at x = 0

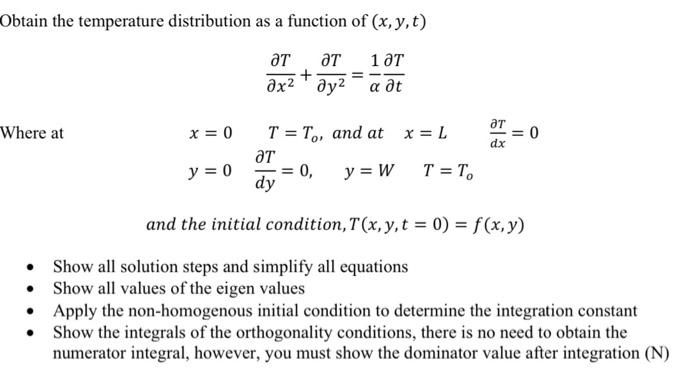
Obtain the temperature distribution as a function of (x, y, t) T 1 T + ax dy a t Where at x = 0 y = 0 T = To, and at x = L T dy = 0, y = W T = T Show all solution steps and simplify all equations Show all values of the eigen values T dx and the initial condition, T(x, y, t = 0) = f(x,y) 0 Apply the non-homogenous initial condition to determine the integration constant Show the integrals of the orthogonality conditions, there is no need to obtain the numerator integral, however, you must show the dominator value after integration (N)

Step by Step Solution

★★★★★

3.39 Rating (158 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

Solution Step1 The temperature distribution as a function of xyt is given by Tx2 Ty2 1 Tt where is a ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

The rope supports a lantern that weighs 50 N. Is the tension in the rope less than, equal to, or more than 50 N? Use the parallelogram rule to defend your answer.

When mixing two types of atoms, say A and B, will atoms always diffuse from regions of higher concentrations to regions of lower concentrations? Explain either in words or with a graph.

In double-slit experiment using light of wavelength 600 nm, the angular width of a fringe formed on a distant screen is 0.1. What is the spacing between the two slits?

After you graduate, you get a job in a small city where you have taken your sisters offer of living in her apartment. Your job pays you $20 per hour and you have up to 60 hours per week available....

-8 -6 9 4 6 4 2 (0, -2) =4 6 y 2 4 6 8 -8 equation of the given graph will be of the form f(x) = al or stretch. Graph the parent function y = log(x), and col vertical shifts? How does the general...

Obtain the temperature distribution as a function of (x, y, t) in rectangular coordinates for unsteady state operating conditions and without heat generation. The domain is two- dimensional (0

Please write a matlab code. If possible avoid using syms in code as it slows down the code. Thank you in advance. In heat transfer one of the important concepts is "Heat Transfer from Extended...

4). Consider the rectangular slab (semi-infinite in the x-direction) depicted below. The surface at x=0 and y=b are maintained at a temperature T. There is a source of energy/volume S in the slab...

Math\t107-6381\t-\tQuiz\t#4\t-\tSchultz\t-\tDue\tFebruary\t21,\t2016\t-\tpage\t1\tof 3 Follow\tthese\tdirections\tcarefully. This\tquiz\tis\tdue\tby\t11:59\tEastern\ttime\ton\tFebruary\t21,\t2016. o...

3. (40 pts +5 pts Bonus) A plastic sheet is made by extruding heated plastic through a die and then allowing the sheet to cool in air prior to being wound on a roller as shown in the schematic...

Euler's Method and Modified Euler's Method: Find a numerical solution to the initial value problem (dy / dx) = 2x + y, y(0) = 1 (1) using the Euler method. Use Euler's method to find a numerical...

If sin(x) = -1/3 and Pi x 3Pi/2, then cot(2x) =

In this exercise we estimate the proportions of listeners familiar with the song who would give the song each rating. Using the definition of conditional probability, we estimate that Note here that...

Find the derivative o f the function. f ( x ) = 3 2 x 2 - 8 2 f ' ( x ) =

For each of the following scenarios, indicate whether an asset has been impaired (Y for yes and N for no) and, if so, the amount of loss that should berecorded. Book Value Fair Value Is Asset...

The partners of Harris and Whelan, attorneys-at-law, decide to implement an activity-based costing system for their firm. They identify the following three cost pools and budgeted amounts for each...

Beasley Company makes three types of exercise machines. Data have been accumulated for four possible overhead drivers. Data for these four possible drivers are shown in rows 3 to 7 of the following...

Presented below are two independent situations: 1. Potomac Inc. acquired 10% of the 500,000 shares of common stock of Maryland Corporation at a total cost of $11 per share on June 17, 2010. On...

Josh Borke recorded the following transactions during the month of April. Post these entries to the Cash T-account of the general ledger to determine the ending balance in cash. The beginning balance...

True/False: Vertical Integration involves a company acquiring or merging with competitors in the same industry to consolidate market power, while Horizontal Integration entails a company controlling...

Show that cos(2x) -1/cosx - 1 = 4 . Do this by first creating a vector x that has the elements 1.0, 0.5, 0.1, 0.01, 0.001, and 0.0001. Then, create a new vector y in which each element is determined...

An aluminum fuel tank has a cylindrical middle section and a semi-spherical ends. The outside diameter is 10 in., and the length of the cylindrical section is 24 in. The wall-thickness of the...

Create a column vector in which the first element is 26, the elements decrease with increments of -3.6, and the last element is -10. (A column vector can be created by the transpose of a row vector.)

24. Suppose that a marketing manager is interested in p, the proportion of con sumers that will buy a particular new product. He considers the following two hypotheses: H0:p = .10 Hi'.p = .20. His...

=+1. A random sample, without replacement, of three items is to be selected from a population of five items (labeled a, b, c, d, and e). a. List all possible different samples. b. List the samples...

=+2. An engineering firm is constructing power plants at three different sites. Define the events E1, E2, and E3 as follows: Section 5.1 Exercises E15 the plant at site 1 is completed by the contract...