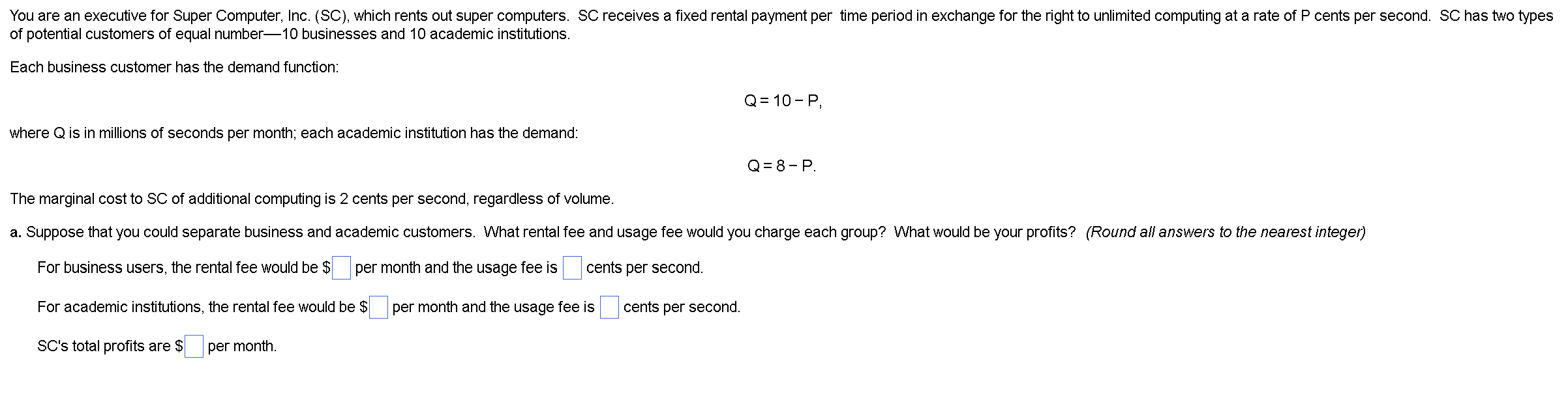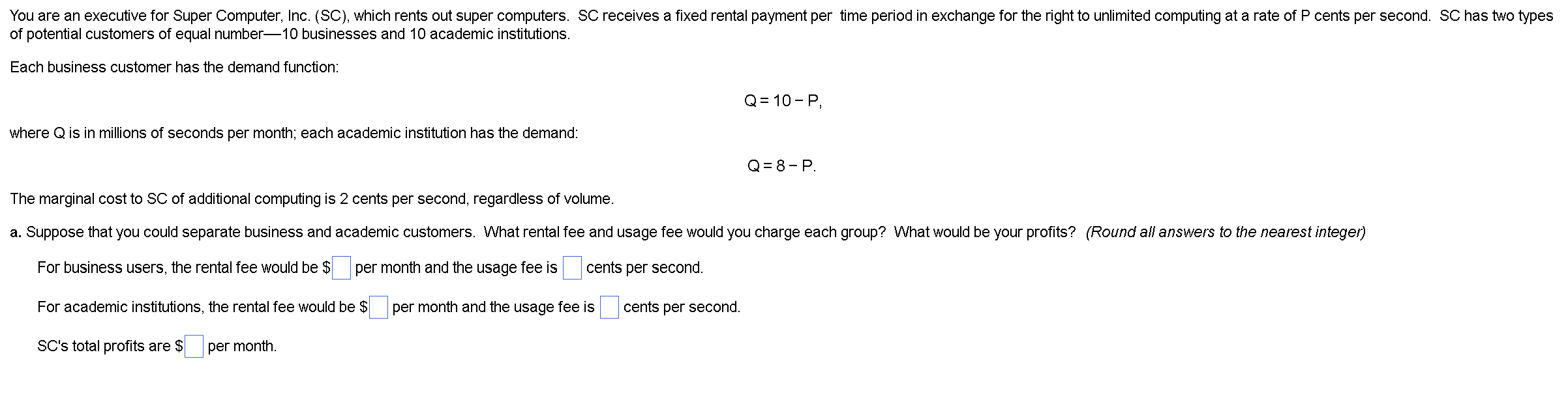

of potential customers of equal number-10 businesses and 10 academic institutions. Each business customer has the demand function: Q=10P where Q is in millions of seconds per month; each academic institution has the demand: Q=8P. The marginal cost to SC of additional computing is 2 cents per second, regardless of volume. For business users, the rental fee would be $ per month and the usage fee is cents per second. For academic institutions, the rental fee would be $ per month and the usage fee is cents per second. SC's total profits are: ] per month. b. Suppose you were unable to keep the two types of customers separate and charged a zero rental fee. What usage fee would maximize your profits? What would be your profits? The profit maximizing usage fee is cents per second. (round your answer to two decimal places) SC's profits are $ per month. (round your answer to the nearest dollar) The profit maximizing rental fee is $ per month (round your answer to the nearest dollar) and the usage fee is cents per second. (round your answer to one decimal place) SC's profits are $ per month. (round your answer to the nearest dollar) of potential customers of equal number-10 businesses and 10 academic institutions. Each business customer has the demand function: Q=10P where Q is in millions of seconds per month; each academic institution has the demand: Q=8P. The marginal cost to SC of additional computing is 2 cents per second, regardless of volume. For business users, the rental fee would be $ per month and the usage fee is cents per second. For academic institutions, the rental fee would be $ per month and the usage fee is cents per second. SC's total profits are: ] per month. b. Suppose you were unable to keep the two types of customers separate and charged a zero rental fee. What usage fee would maximize your profits? What would be your profits? The profit maximizing usage fee is cents per second. (round your answer to two decimal places) SC's profits are $ per month. (round your answer to the nearest dollar) The profit maximizing rental fee is $ per month (round your answer to the nearest dollar) and the usage fee is cents per second. (round your answer to one decimal place) SC's profits are $ per month. (round your answer to the nearest dollar)