Question
One project in a junior mechanical engineering course was to design a manually operated aluminum-can compactor. The prototype in Figure 5-1 was developed and built
One project in a junior mechanical engineering course was to design a manually operated aluminum-can compactor. The prototype in Figure 5-1 was developed and built by a team of threeJoyce, Ted, and Will. Having been convinced by their classmates that it would sell well, they are now trying to convince Teds father to manufacture it in his machine shop. This machine shop employs 15 people doing a large variety of small batch fabrications for about 40 local manufacturing plants. Recently, one of their two largest customers announced plans to move their plant to another state, thus the shop is facing a 20% drop in business. Teds father, Mr. Dick Strong, has identified three options for dealing with this loss of business. Through a combination of price cuts and vigorous marketing, he can attract business away from his principal competitors. He expects that this strategy would lead to financial ruin, as he has historically worked hard at marketing and competitive pressures have kept his profit margins relatively low. He finds the option of layoffs nearly as unattractive. Skilled machinists are hard to find, so any he lays off are unlikely to be available for rehire later. Also the damage to shop morale will be high, making it likely that additional employees might leave, thus making it more difficult to hire replacements. Layoffs would also damage his self-respect and reputation, as he has never laid someone off and he has stressed that Strong Metalworks is a family. The only other option he can envision is adding a new kind of activity to his business. This could represent (1) a new process such as plating, which he now subcontracts; (2) a new market, such as sending his salespeople into the next city; or (3) developing products for wholesale or retail sale rather than simply fabricating components to be incorporated into the products of others. Not surprisingly, Mr. Strong is most interested in this last possibility, and he is considering the suggested product from the student team. Although he has other possibilities, he would like to encourage his son and the other students. Whichever product is selected for development, if any, Mr. Strong recognizes that this initial venture into a new mode of operations is unlikely to replace the entire 20% drop in business. It may not even be profitable. Rather, he views this as an experiment whose primary value may be marketing information and experience. Whereas coping with the unanticipated decline in demand is the primary motivation, fabrication of complete products for direct sale will also allow the demand for labor to be smoothed through production to inventory. Figure 5-1 shows the concept and operation of the students can compactor. Figure 5-2 provides a visual guide to product configuration and assembly. Both drawings illustrate that the compactor operation consists of a sliding mass within a cylinder to compress the aluminum can. The window in the cylinder allows can insertion, while a second smaller window permits safe, automatic removal (it falls out). In Table 5-1, the student time and material cost for product development are shown. The development has included a design effort, prototype testing, and final write-up. After discussing the manufacturing process with Mr. Strong, the students agreed that the product design could be refined. This redesign will emphasize improving the can compactors manufacturability and its effectiveness. Table 5-2 summarizes the expected time and material cost to modify the design and to produce 20 revised prototypes. Once the design is finalized, it is expected that the material cost can be trimmed by 40% and the manufacturing time by 70%. Although new capital equipment may be justifiable, Mr. Strong has ruled it out for now. Student time may be treated as a freebie. (They get learning, experience, and prototypes for themselves and friends.) The employees of Strong Metalworks average $17.50/hour in wages and fringe benefits. The burden rate for calculating the cost of administrative overhead is 55%. Mr. Strong has stated that this can be ignored for the redesign phase since those units will not be sold.
Joyce has also taken a marketing class, so she examined the possibilities for sales of this product. To begin with, local stores offer specialized manual equipment at a cost of $35 to $40. Although exact estimates are not possible, one store whose stock and level of business seemed average admitted to annual sales of 20 compactors. The yellow pages yielded a count of 25 such stores in the local area. Joyce suggested that from the number of stores selling compactors, the level of sales would increase by 150 per year for the next five years. Later expansion to two nearby metropolitan communities should support similar increases in demand. Mr. Strong asked the team to estimate when the product will break even (ignoring the time value of money). Options 1. Calculate the equivalent annual worth over a 15 year life considering no inflation and a discount rate of 5%. 2. Instead of assuming that sales increase by 150 per year, reevaluate the project assuming a 20% rate for growth in demand. Suggestion to the Student 1. The alternative in this case is easy to identify, but there have been at least three objectives identified. What are the two non-economic objectives? 2. You must somehow derive a wholesale price for the can cruncher. Typical markups at the manufacturing and retail levels might be 30% to 40%. 3. What is the fixed cost for continued development? 4. What is the variable cost for production, and how much contribution or profit is there per unit? 5. Calculate the cumulative production, the total cost, and the total revenue over time. What is the breakeven point? 6. Given the economic results and the non-economic criteria, what do you recommend to Mr. Strong? For The Options: 7. If the growth in sales is projected at a constant amount per year, then an arithmetic gradient can be used for a simple equivalence equation. If the growth is at a constant rate, then a geometric gradient must be used. This is best analyzed with a spreadsheet or an equivalent discount rate. Table 5-3 is one way to structure the analysis for comparison with manual calculated values.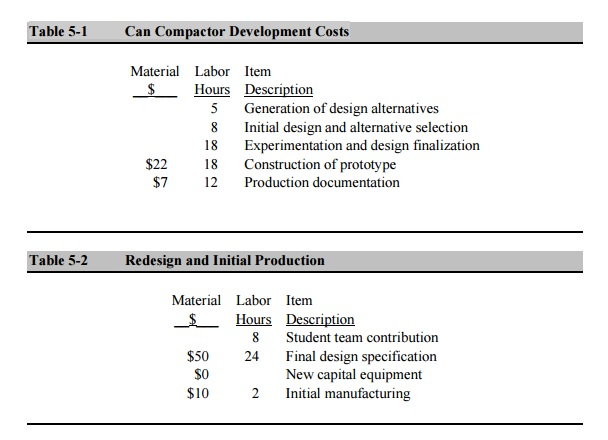
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


