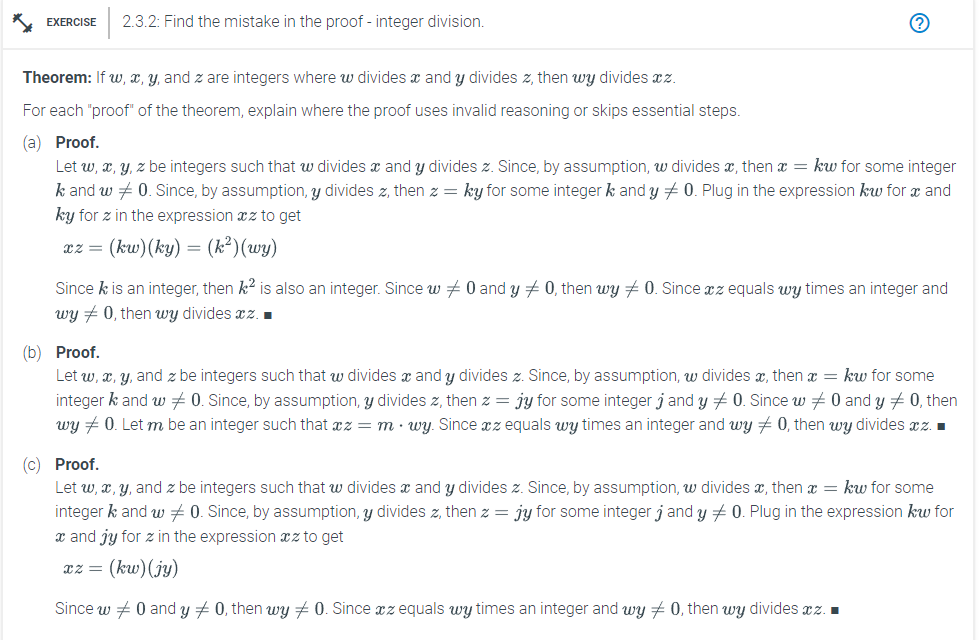
Only question C

$2\mathrm{.}3\mathrm{.}2$: Find the mistake in the proof $-$ integer division.

Theorem: If $w,x,y,$ and $z$ are integers where $w$ divides $x$ and $y$ divides $z,$ then $wy$ divides $xz.$

For each "proof" of the theorem, explain where the proof uses invalid reasoning or skips essential steps.

$($a$)$ Proof.

Let $w,x,y,z$ be integers such that $w$ divides $x$ and $y$ divides $z.$ Since, by assumption, $w$ divides $x,$ then $x=kw$ for some integer

$k$ and $w0.$ Since, by assumption, $y$ divides $z,$ then $z=ky$ for some integer $k$ and $y0.$ Plug in the expression $kw$ for $x$ and

$ky$ for $z$ in the expression $xz$ to get

$xz=(kw)(ky)=(k^2)(wy)$

Since $k$ is an integer, then $k^2$ is also an integer. Since $w0$ and $y0,$ then $wy0.$ Since $xz$ equals $wy$ times an integer and

$wy0,$ then $wy$ divides $xz.$

$($b$)$ Proof.

Let $w,x,y,$ and $z$ be integers such that $w$ divides $x$ and $y$ divides $z.$ Since, by assumption, $w$ divides $x,$ then $x=kw$ for some

integer $k$ and $w0.$ Since, by assumption, $y$ divides $z,$ then $z=jy$ for some integer $j$ and $y0.$ Since $w0$ and $y0,$ then

$wy0.$ Let $m$ be an integer such that $xz=m*wy.$ Since $xz$ equals $wy$ times an integer and $wy0,$ then $wy$ divides $xz.$

$($c$)$ Proof.

Let $w,x,y,$ and $z$ be integers such that $w$ divides $x$ and $y$ divides $z.$ Since, by assumption, $w$ divides $x,$ then $x=kw$ for some

integer $k$ and $w0.$ Since, by assumption, $y$ divides $z,$ then $z=jy$ for some integer $j$ and $y0.$ Plug in the expression $kw$ for

$x$ and $jy$ for $z$ in the expression $xz$ to get

$xz=(kw)(jy)$

Since $w0$ and $y0,$ then $wy0.$ Since $xz$ equals $wy$ times an integer and $wy0,$ then $wy$ divides $xz.$