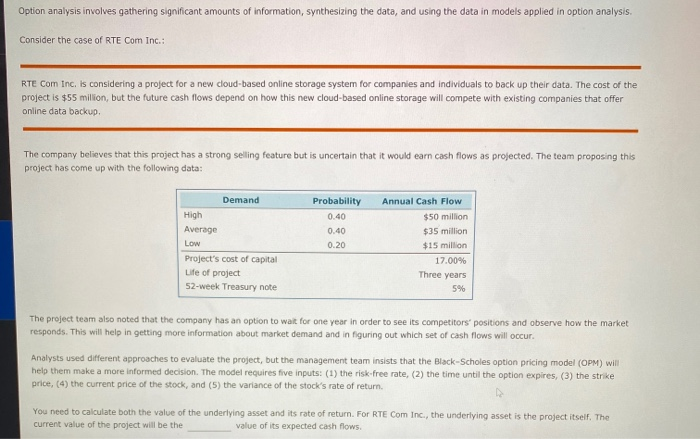
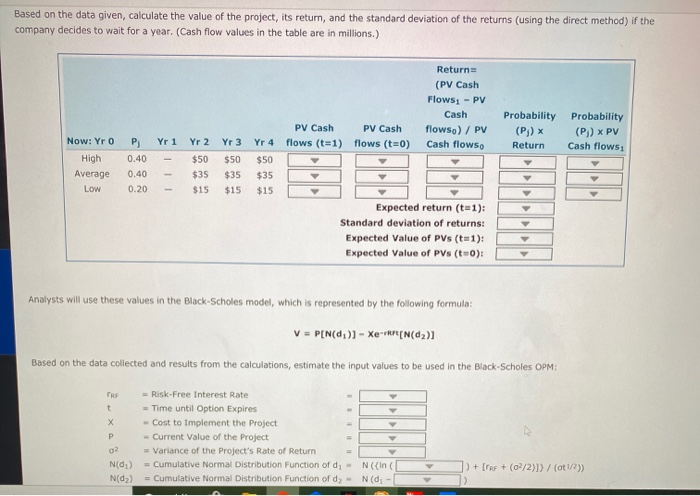
Option analysis involves gathering significant amounts of information, synthesizing the data, and using the data in models applied in option analysis, Consider the case of RTE Com Inc.: RTE Com Inc. is considering a project for a new cloud-based online storage system for companies and individuals to back up their data. The cost of the project is $55 million, but the future cash flows depend on how this new cloud-based online storage will compete with existing companies that offer online data backup The company believes that this project has a strong selling feature but is uncertain that it would earn cash flows as projected. The team proposing this project has come up with the following data: Demand High Average Low Project's cost of capital Life of project 52-week Treasury note Probability 0.40 0.40 0.20 Annual Cash Flow $50 million $35 million $15 millon 17.00% Three years 5% The project team also noted that the company has an option to wait for one year in order to see its competitors' positions and observe how the market responds. This will help in getting more information about market demand and in figuring out which set of cash flows will occur. Analysts used different approaches to evaluate the project, but the management team insists that the Black-Scholes option pricing model (OPM) will help them make a more informed decision. The model requires five inputs: (1) the risk-free rate, (2) the time until the option expires, (3) the strike price, (4) the current price of the stock, and (5) the variance of the stock's rate of return You need to calculate both the value of the underlying asset and its rate of return. For RTE Com Inc., the underlying asset is the project itself. The current value of the project will be the value of its expected cash flows. Based on the data given, calculate the value of the project, its return, and the standard deviation of the returns (using the direct method) if the company decides to wait for a year. (Cash flow values in the table are in millions.) Return (PV Cash Flows - PV Cash flowso) / PV Cash flows. Probability (P) Return Probability (P) x PV Cash flows Now: Yr O High Average Low P 0.40 0.40 0.20 Y 1 Yr 2 - $50 - $35 - $15 PV Cash PV Cash flows (t=1) flows (t=0) [ Yr3 $50 $35 $15 Yr 4 $50 $35 $15 Expected return (t-1): Standard deviation of returns: Expected Value of PVs (t=1): Expected Value of PVs ( 0) Analysts will use these values in the Black-Scholes model, which is represented by the following formula: V = P[N(d1)] - Xe" (N(d2)] Based on the data collected and results from the calculations, estimate the input values to be used in the Black-Scholes OPM: T t X P = Risk-Free Interest Rate = Time until Option Expires - Cost to implement the Project = Current Value of the Project = Variance of the Project's Rate of Return - Cumulative Normal Distribution Function of d; = Cumulative Normal Distribution Function of d) - N(d) N(d) N(in ([ N(d, - ) + the + (0/2)]>/(at1/2)) Option analysis involves gathering significant amounts of information, synthesizing the data, and using the data in models applied in option analysis, Consider the case of RTE Com Inc.: RTE Com Inc. is considering a project for a new cloud-based online storage system for companies and individuals to back up their data. The cost of the project is $55 million, but the future cash flows depend on how this new cloud-based online storage will compete with existing companies that offer online data backup The company believes that this project has a strong selling feature but is uncertain that it would earn cash flows as projected. The team proposing this project has come up with the following data: Demand High Average Low Project's cost of capital Life of project 52-week Treasury note Probability 0.40 0.40 0.20 Annual Cash Flow $50 million $35 million $15 millon 17.00% Three years 5% The project team also noted that the company has an option to wait for one year in order to see its competitors' positions and observe how the market responds. This will help in getting more information about market demand and in figuring out which set of cash flows will occur. Analysts used different approaches to evaluate the project, but the management team insists that the Black-Scholes option pricing model (OPM) will help them make a more informed decision. The model requires five inputs: (1) the risk-free rate, (2) the time until the option expires, (3) the strike price, (4) the current price of the stock, and (5) the variance of the stock's rate of return You need to calculate both the value of the underlying asset and its rate of return. For RTE Com Inc., the underlying asset is the project itself. The current value of the project will be the value of its expected cash flows. Based on the data given, calculate the value of the project, its return, and the standard deviation of the returns (using the direct method) if the company decides to wait for a year. (Cash flow values in the table are in millions.) Return (PV Cash Flows - PV Cash flowso) / PV Cash flows. Probability (P) Return Probability (P) x PV Cash flows Now: Yr O High Average Low P 0.40 0.40 0.20 Y 1 Yr 2 - $50 - $35 - $15 PV Cash PV Cash flows (t=1) flows (t=0) [ Yr3 $50 $35 $15 Yr 4 $50 $35 $15 Expected return (t-1): Standard deviation of returns: Expected Value of PVs (t=1): Expected Value of PVs ( 0) Analysts will use these values in the Black-Scholes model, which is represented by the following formula: V = P[N(d1)] - Xe" (N(d2)] Based on the data collected and results from the calculations, estimate the input values to be used in the Black-Scholes OPM: T t X P = Risk-Free Interest Rate = Time until Option Expires - Cost to implement the Project = Current Value of the Project = Variance of the Project's Rate of Return - Cumulative Normal Distribution Function of d; = Cumulative Normal Distribution Function of d) - N(d) N(d) N(in ([ N(d, - ) + the + (0/2)]>/(at1/2))