Oxygen delivery and consumption in muscle tissues The Krogh Cylinder and the Krogh$-$Erlang Equation Almost all living beings need oxygen to survive. In all vertebrates, a respiratory system enables the oxygen to enter the organism. Oxygen has very low solubility in water $($and blood$),$ therefore it is mostly carried

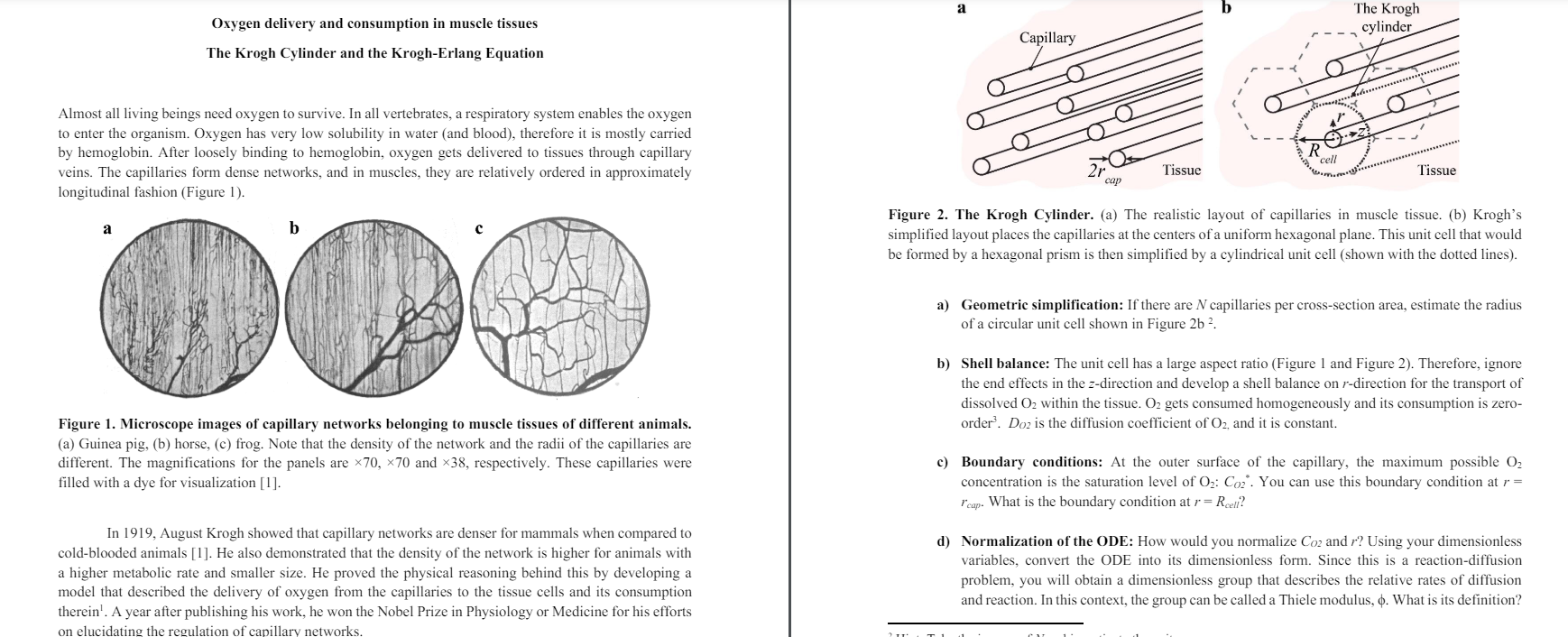
by hemoglobin. After loosely binding to hemoglobin, oxygen gets delivered to tissues through capillary veins. The capillari In $1919,$ August Krogh showed that capillary networks are denser for mammals when compared to cold$-$blooded animals $.$ He also demonstrated that the density of the network is higher for animals with a higher metabolic rate and smaller size. He proved the physical reasoning behind this by developing a model that described the delivery of oxygen from the capillaries to the tissue cells and its consumption therein. A year after publishing his work, he won the Nobel Prize in Physiology or Medicine for his efforts on elucidating the regulation of capillary networks. In this active session, more than $100$ years later, we will analyze Krogh$$s model on oxygen diffusion and consumption in tissues, which is a classic reaction$-$diffusion problem. Our goal to is to obtain an estimate of the concentration profile of dissolved oxygen within muscle tissue and analyze its consequences. Krogh had a brilliant perspective for modelling the capillary network and the tissue encapsulating it$.$ He visualized the network as a regular array of capillaries, each placed at the center of a close packed $($hexagonal$)$ unit cell $($Figure $2$a and $2$b$).$ Then, he approximated the unit cell with a cylinder, so that the problem was amenable to analytical analysis.. This unit cell that would be formed by a hexagonal prism is then simplified by a cylindrical unit cell. a$)$ Geometric simplification: If there are N capillaries per cross$-$section area, estimate the radius of a circular unit cell shown in Figure $2$b $2.$ b$)$ Shell balance: The unit cell has a large aspect ratio $($Figure $1$ and Figure $2).$ Therefore, ignore the end effects in the z$-$direction and develop a shell balance on r$-$direction for the transport of dissolved O$2$ within the tissue. O$2$ gets consumed homogeneously and its consumption is zeroorder. DO$2$ is the diffusion coefficient of O$2,$ and it is constant.

c$)$ Boundary conditions: At the outer surface of the capillary, the maximum possible O$2$ concentration is the saturation level of O$2$: CO$2*.$ You can use this boundary condition at r $=$rcap. What is the boundary condition at r $=$ Rcell?

d$)$ Normalization of the ODE: How would you normalize CO$2$ and r$?$ Using your dimensionless variables, convert the ODE into its dimensionless form. Since this is a reaction$-$diffusion problem, you will obtain a dimensionless group that describes the relative rates of diffusion and reaction. In this context, the group can be called a Thiele modulus, $.$ What is its definition? e$)$ Normalization of the BCs: What are the boundary conditions for the dimensionless variables? Use $\backslash$kappa $=$ rcap$/$Rcell$.$

f$)$ The solution: Solve the differential equation.

g$)$ Critical value of $$: $$crit$.$ Note that if $$ is larger than a certain value, O$2$ will not reach to the boundary of the unit cell. In that case, an oxygen$-$starved, a$.$k$.$a$.$ anoxic, region will develop. In a few minutes, the anoxic cells will die. This is called necrosis. Develop an expression for $$crit$.$

h$)$ The meaning of $\backslash$kappa $.$ Go back to the definition of Rcell and show that $\backslash$kappa is equal to $\backslash$alpha cap, which is the areal density of capillaries. That is$,\backslash$alpha cap $=($total cross$-$section area of all capillaries$)/($total cross$-$section area of the tissue$).$ Write $$crit in terms of $\backslash$alpha cap instead of $\backslash$kappa $.$

i$)$ Critical rate constant, k$0,$crit. The zero$-$order rate constant is representative of the metabolic rate. In conjunction with the result of part $($h$)$ for $$crit$,$ use the definition of $$ that you obtained after normalization and obtain an expression for the maximum O$2$ consumption rate. This value is related to the maximum metabolic rate allowable before an anoxic region develops.

j$)$ Trends. Go back to the definition of $$ that you obtained after normalization and replace Rcell with N$.$ Near $=$crit levels, necrosis occurs if any of the following occurs.

$-$ Presence of tumor cells

$-$ Faulty capillaries

$-$ Low levels of O$2$ saturation in blood$5.$

Identify which parameter in the definition of $$ changes for the cases given above. Does the parameter increase or decrease?

k$)$ Calculating $$crit$.$ For the muscle tissue of a horse, N $=3000$ mm$-2$

and $2$rcap $=7\mathrm{.}2\backslash$mu m$.$ Calculate Rcell and $$crit$.$ Do the same for a frog tissue: N $=400$ mm$-2$ and $2$rcap $=15\backslash$mu m$.$

l$)$ The Krogh length, $\backslash$Lambda $.$ Note that for the different tissues in part $($k$),$crit ~ $2.$ Using the definition of $,$ derive a length scale that approximately characterizes the maximum distance between the capillaries. For similar CO$2*$

and DO$2,$ note that the distance $\backslash$Lambda is determined by the metabolic

rate only, as Krogh showed experimentally.