Answered step by step
Verified Expert Solution
Question
1 Approved Answer
P1) Prove the Mean Value Property in 2 dimensions: If u is harmonic in C R and D(xo, a) CN, then 1 u(xo) u(x)
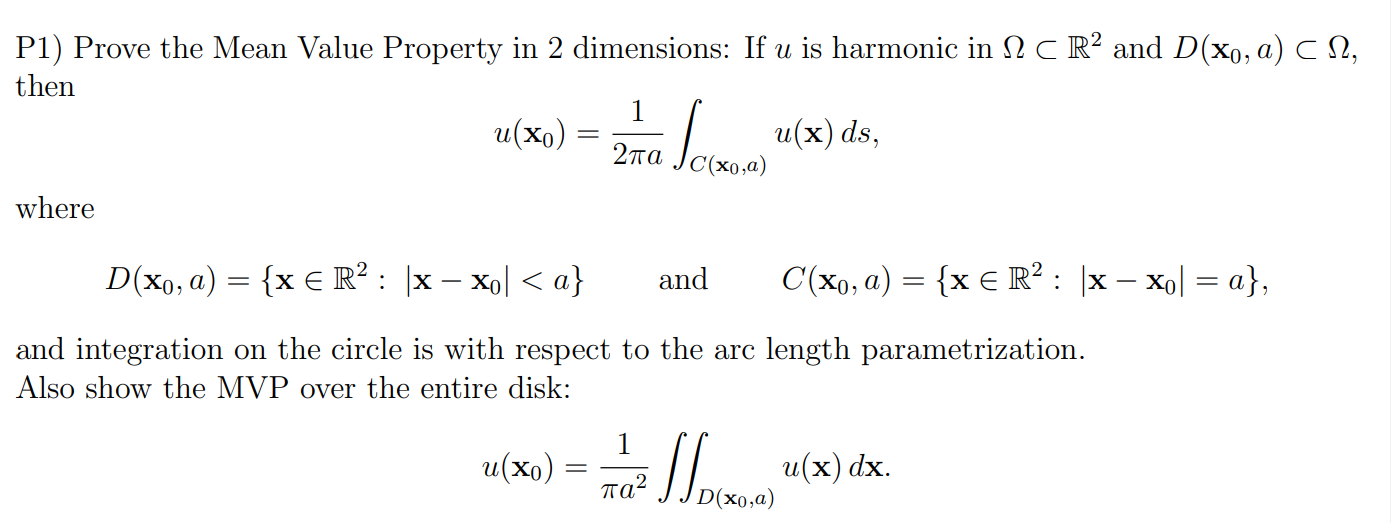
P1) Prove the Mean Value Property in 2 dimensions: If u is harmonic in C R and D(xo, a) CN, then 1 u(xo) u(x) ds, 2 C(xo,a) where D(xo, a) = {x = R : |x xo| < a} and C(xo, a) = {x = R : |x x0| = a}, and integration on the circle is with respect to the arc length parametrization. Also show the MVP over the entire disk: u(xo) = 1 ) u(x) dx.
Step by Step Solution
There are 3 Steps involved in it
Step: 1
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Calculus Early Transcendentals
Authors: James Stewart
7th edition
538497904, 978-0538497909
