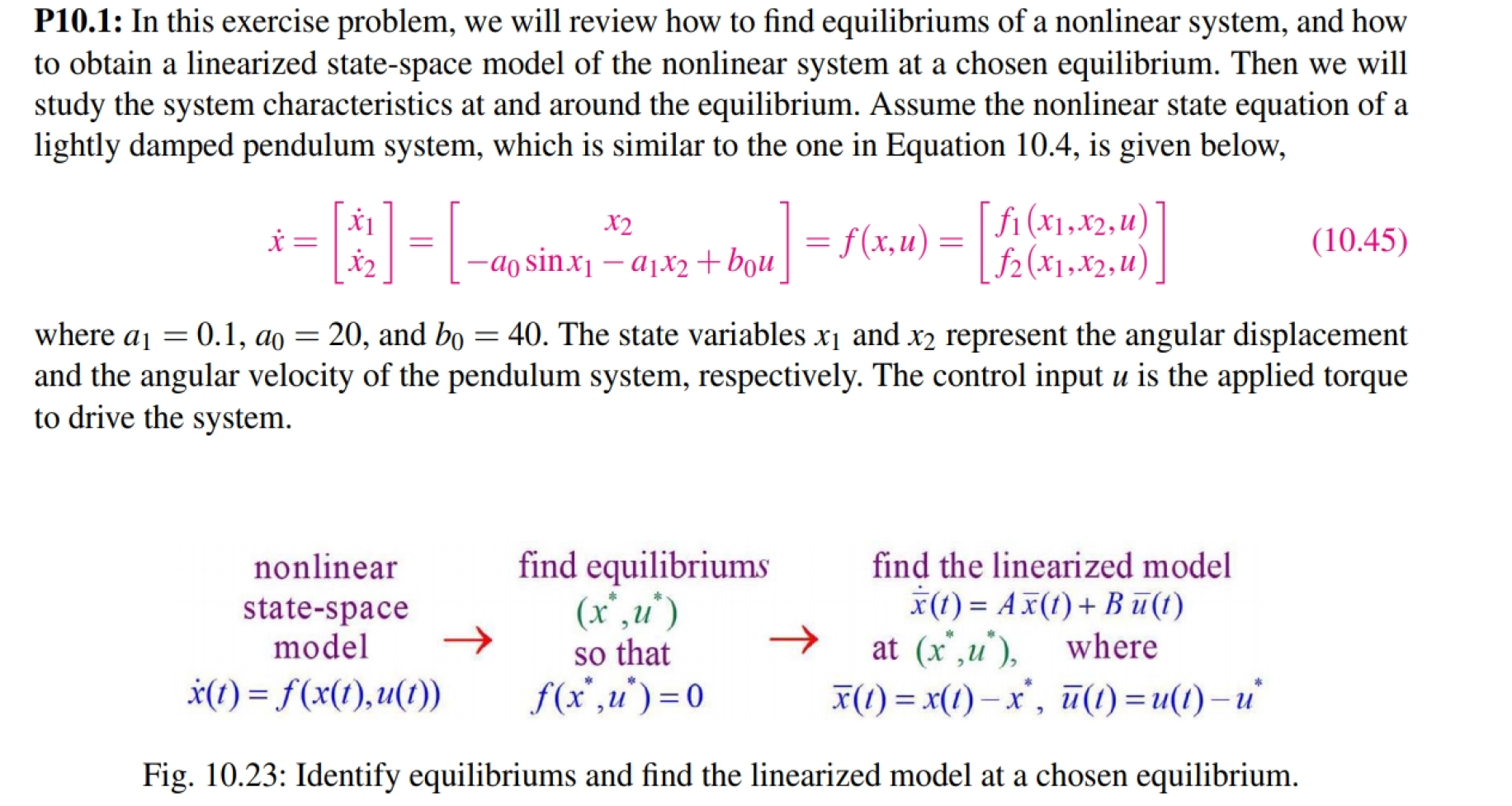
P10.1: In this exercise problem, we will review how to find equilibriums of a nonlinear system, and how to obtain a linearized state-space model of the nonlinear system at a chosen equilibrium. Then we will study the system characteristics at and around the equilibrium. Assume the nonlinear state equation of a lightly damped pendulum system, which is similar to the one in Equation 10.4, is given below, i= -=[!] = [-coins , in town (10.45) fi(x1,x2,u) -a, sinx 21x2+bou = f(x,u) = ) | f2 (x1,x2,u) where aj = 0.1, 0o = 20, and bo = 40. The state variables xi and x2 represent the angular displacement and the angular velocity of the pendulum system, respectively. The control input u is the applied torque to drive the system. nonlinear state-space model (t) = f(x(t),u(t)) find equilibriums (x",u") find the linearized model X(t) = A x(1) + B(0 at (x",u), where F(t) = x(t) x", (t)=u(t) u* so that f(x*,u") = 0 Fig. 10.23: Identify equilibriums and find the linearized model at a chosen equilibrium. P10.1c: Find the characteristic equation of the linearized model and the poles of the system, and com- ment on the system characteristics based on the damping ratio, natural frequency, and the location of the poles on the complex plane. P10.1: In this exercise problem, we will review how to find equilibriums of a nonlinear system, and how to obtain a linearized state-space model of the nonlinear system at a chosen equilibrium. Then we will study the system characteristics at and around the equilibrium. Assume the nonlinear state equation of a lightly damped pendulum system, which is similar to the one in Equation 10.4, is given below, i= -=[!] = [-coins , in town (10.45) fi(x1,x2,u) -a, sinx 21x2+bou = f(x,u) = ) | f2 (x1,x2,u) where aj = 0.1, 0o = 20, and bo = 40. The state variables xi and x2 represent the angular displacement and the angular velocity of the pendulum system, respectively. The control input u is the applied torque to drive the system. nonlinear state-space model (t) = f(x(t),u(t)) find equilibriums (x",u") find the linearized model X(t) = A x(1) + B(0 at (x",u), where F(t) = x(t) x", (t)=u(t) u* so that f(x*,u") = 0 Fig. 10.23: Identify equilibriums and find the linearized model at a chosen equilibrium. P10.1c: Find the characteristic equation of the linearized model and the poles of the system, and com- ment on the system characteristics based on the damping ratio, natural frequency, and the location of the poles on the complex plane