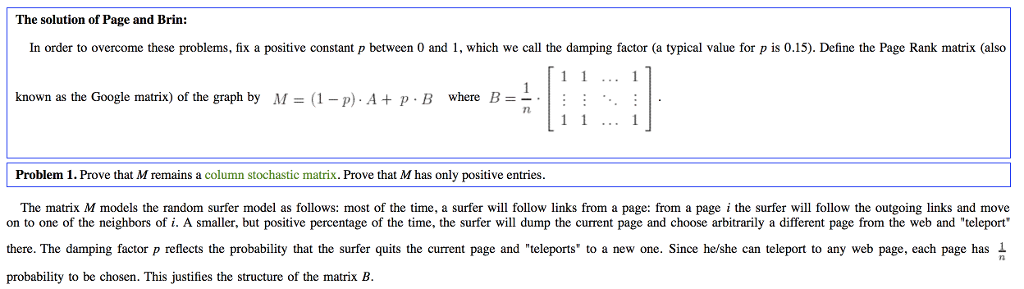
Page Rank

The solution of Page and Brin: In order to overcome these problems, fix a positive constant p between 0 and 1, which we call the damping factor (a typical value for p is 0.15).Define the Page Rank matrix (also known as the Google matrix) of the graph by M= (1-P)-A+p.B where B=- n. Problem 1. Prove that M remains a column stochastic matrix. Prove that M has only positive entries. The matrix M models the random surfer model as follows: most of the time, a surfer will follow links from a page: from a page i the surfer will follow the outgoing links and move on to one of the neighbors of i. A smaller, but positive percentage of the time, the surfer will dump the current page and choose arbitrarily a different page from the web and "teleport' there. The damping factor p reflects the probability that the surfer quits the current page and "teleports" to a new one. Since he/she can teleport to any web page, each page has probability to be chosen. This justifies the structure of the matrix B. The solution of Page and Brin: In order to overcome these problems, fix a positive constant p between 0 and 1, which we call the damping factor (a typical value for p is 0.15).Define the Page Rank matrix (also known as the Google matrix) of the graph by M= (1-P)-A+p.B where B=- n. Problem 1. Prove that M remains a column stochastic matrix. Prove that M has only positive entries. The matrix M models the random surfer model as follows: most of the time, a surfer will follow links from a page: from a page i the surfer will follow the outgoing links and move on to one of the neighbors of i. A smaller, but positive percentage of the time, the surfer will dump the current page and choose arbitrarily a different page from the web and "teleport' there. The damping factor p reflects the probability that the surfer quits the current page and "teleports" to a new one. Since he/she can teleport to any web page, each page has probability to be chosen. This justifies the structure of the matrix B