Question
PART 1 Continental tire is used for F-35 fighter jets in USA. Before every take off, the tire pressure and quality of the tire is
PART 1
Continental tire is used for F-35 fighter jets in USA. Before every take off, the tire pressure and quality of
the tire is being measured. After allocating all the data, it's been found that Continental tires have an
average life span of 8000 hours in normal weather condition. However, in desert the average life span of
the tire becomes as low as 5000 hours. Suppose, the continental tire's average life span is what it gets
during normal weather condition and has a standard deviation of 400 hours. Find the probability that the
mean life of a random sample of 25 such tires is -
a) less than 7890 hours
b) between 7850 and 7910 hours
c) within 130 hours of the population mean
d) less than the population mean by 150 hours or more
Question 2:
Two thousand randomly selected adults were asked if they are in favor of or against cloning. The
following table gives the responses.
In Favor Against No Option
Male 395 405 100
Female 300 680 120
If one person is selected at random from these 2000 adults, find the probability that this person is-
a) in favor of cloning
b) against cloning
c) in favor of cloning given the person is a female
d) a male given the person has no opinion
e) Are the events "female" and "no opinion" independent? Why or why not?
Question 3:
A random sample of 8 observations taken from a population that is normally distributed produced a
sample mean of 44.98 and a standard deviation of 6.77. Find the critical and observed values of t and the
ranges for the p-value for each of the following tests of hypotheses, using = .05.
a. H0: = 50 versus H1: 50
b. H0: = 50 versus H1:
Question 4: 12 Marks
Heinz, a manufacturer of ketchup, uses a particular machine to dispense 16 ounces of its ketchup into
containers. From many years of experience with the particular dispensing machine, Heinz knows the
amount of product in each container follows a normal distribution with a mean of 16 ounces and a
standard deviation of 0.15 ounce. A sample of 50 containers filled last hour revealed the mean amount
per container was 16.017 ounces. Does this evidence suggest that the mean amount dispensed is different
from 16 ounces? Use the .05 significance level.
(a) State the null hypothesis and the alternate hypothesis.
(b) What is the probability of a Type I error?
(c) Give the formula for the test statistic.
(d) State the decision rule.
(e) Determine the value of the test statistic.
(f) What is your decision regarding the null hypothesis?
(g) Interpret, in a single sentence, the result of the statistical test.
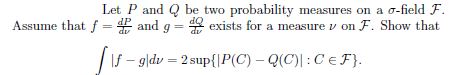


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


