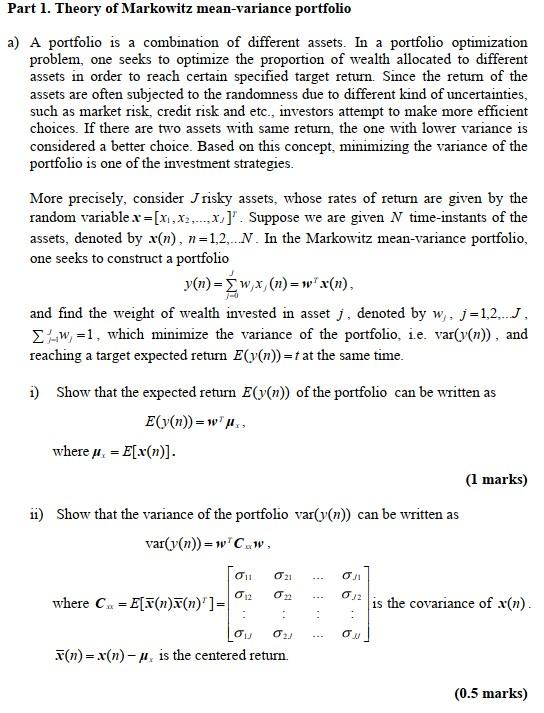
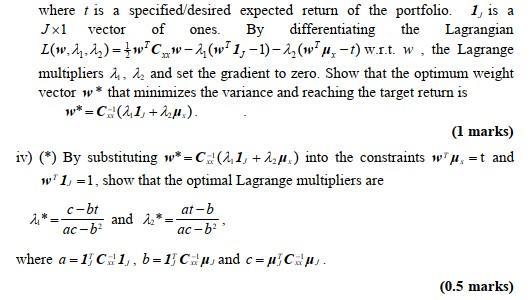
Part 1. Theory of Markowitz mean-variance portfolio a) A portfolio is a combination of different assets. In a portfolio optimization problem, one seeks to optimize the proportion of wealth allocated to different assets in order to reach certain specified target return. Since the return of the assets are often subjected to the randomness due to different kind of uncertainties, such as market risk, credit risk and etc., investors attempt to make more efficient choices. If there are two assets with same return the one with lower variance is considered a better choice. Based on this concept, minimizing the variance of the portfolio is one of the investment strategies. More precisely, consider risky assets, whose rates of return are given by the random variable x = [x1,x2,...,x.]". Suppose we are given N time-instants of the assets, denoted by x(n), n=1,2, N. In the Markowitz mean-variance portfolio, one seeks to construct a portfolio y(n)= w;x (n)=w'x(n), and find the weight of wealth invested in asset j, denoted by w. j=1,2,J, {}-w;=1, which minimize the variance of the portfolio, i.e. var(v(n)), and reaching a target expected return E(v(n))=t at the same time. 1-0 1) Show that the expected return Ev(n)) of the portfolio can be written as Ev(n))=w". where . - E[x(n)]. (1 marks) 11) Show that the variance of the portfolio var(y(n)) can be written as var(v(n)) =w'CW 011 021 012 02 is the covariance of x(n). . : where C. = E[7(n)(n)']= Lou F(n)= x(n)-#, is the centered return. 02. Oy = (0.5 marks) 111) The mean-variance portfolio optimization problem can be written as: min *w'CW subject to wat w 1,=1 9 where t is a specified/desired expected return of the portfolio. 1, is a Jx1 vector of ones. By differentiating the Lagrangian L(w, m, 1) = 4w'CW-24(w1,-1)- 12(w?, -t)w.l.t. w, the Lagrange multipliers , iz and set the gradient to zero. Show that the optimum weight vector w* that minimizes the variance and reaching the target return is w*=C: (11,+). (1 marks) iv) (*) By substituting w* =C(1,1, +AsJl.) into the constraints w'yland w'1, =1, show that the optimal Lagrange multipliers are c-bt ac-b2 and le at-b ac-b2 where a=1; C. 1,, b=1 Cuand c='. a= (0.5 marks) Part 1. Theory of Markowitz mean-variance portfolio a) A portfolio is a combination of different assets. In a portfolio optimization problem, one seeks to optimize the proportion of wealth allocated to different assets in order to reach certain specified target return. Since the return of the assets are often subjected to the randomness due to different kind of uncertainties, such as market risk, credit risk and etc., investors attempt to make more efficient choices. If there are two assets with same return the one with lower variance is considered a better choice. Based on this concept, minimizing the variance of the portfolio is one of the investment strategies. More precisely, consider risky assets, whose rates of return are given by the random variable x = [x1,x2,...,x.]". Suppose we are given N time-instants of the assets, denoted by x(n), n=1,2, N. In the Markowitz mean-variance portfolio, one seeks to construct a portfolio y(n)= w;x (n)=w'x(n), and find the weight of wealth invested in asset j, denoted by w. j=1,2,J, {}-w;=1, which minimize the variance of the portfolio, i.e. var(v(n)), and reaching a target expected return E(v(n))=t at the same time. 1-0 1) Show that the expected return Ev(n)) of the portfolio can be written as Ev(n))=w". where . - E[x(n)]. (1 marks) 11) Show that the variance of the portfolio var(y(n)) can be written as var(v(n)) =w'CW 011 021 012 02 is the covariance of x(n). . : where C. = E[7(n)(n)']= Lou F(n)= x(n)-#, is the centered return. 02. Oy = (0.5 marks) 111) The mean-variance portfolio optimization problem can be written as: min *w'CW subject to wat w 1,=1 9 where t is a specified/desired expected return of the portfolio. 1, is a Jx1 vector of ones. By differentiating the Lagrangian L(w, m, 1) = 4w'CW-24(w1,-1)- 12(w?, -t)w.l.t. w, the Lagrange multipliers , iz and set the gradient to zero. Show that the optimum weight vector w* that minimizes the variance and reaching the target return is w*=C: (11,+). (1 marks) iv) (*) By substituting w* =C(1,1, +AsJl.) into the constraints w'yland w'1, =1, show that the optimal Lagrange multipliers are c-bt ac-b2 and le at-b ac-b2 where a=1; C. 1,, b=1 Cuand c='. a= (0.5 marks)