Part 1:

True-False questions: Answer all questions. Whenever the question concerns a consumption set, assume that C = R2 +.

1. If the price of either good falls, assuming income if xed, the budget set at the old higher price is contained completely in the budget constraint at the new lower price.

2. A consumer has preferences that are strictly monotone (i.e., "more is better"). Say her income doubles, and the price of good two goes up, but just not by that much. These changes must make the consumer better o.

3. If preferences are not convex, then for any commodity bundle x, the set of commodity bundles that are worse than x can never be a convex set.

4. If preferences are convex and strictly monotonic, the set of consumption bundles that form an indierence curve itself is a convex set.

5. If someone has a utility function U(x,y)= 1000 + 16 min {x1 +x2}, then goods x1 and x2 are perfect complements for that person.

6. If a consumer has convex preferences, then a point of tangency between her indierence curve and her budget line does not necessarily characterize all demand choices.

7. A consumer with preferences u(x1; x2) = x1 + x 3 2 has "convex preferences" (meaning, the weakly preference sets for any consumption bundle are convex sets).

8. A consumer with preferences u(x1; x2) = 20 (ax1 + bx2) 2 +10 has strictly convex preferences (meaning the weakly preferenced sets strictly convex sets).

9. The utility function u(x1; x2) = ln c1 + ln c2 represents the same preferences as some quasilinear utility function.

10. For any optimizing consumer demand choice for xed positive prices and income but does not have strictly convex preferences can never have a unique optimal choice for demand.

11. When preferences are convex, and not strictly convex, many solutions for demand are always possible at every price.

12. When preferences are nonconvex, the set of demands for the consumer will always be at least 2 choices at every price.

13. When preferences are Leontief, the consumer always ends up eating all of one good.

14. Let the utility function be u(c) = c 2 1 + c2: These preferences are convex, but not strictly convex.

15. The preferences u(c1; c2) = c a 1 c b 2 for a > 0; b > 0 are strictly convex.

Question 2.

Directions. That all the problems. Homework is due Monday, February 2,2021 at 5pm.1.Budget sets.Say we have 2 goods, and that the absolute price of good 1 is10, and of good 2 is 20 (so the absolute price vector isP= (P1;P2) = (10;20),and incomemis 100).a. Dene the consumption set, and then plot the budget set at thisP:b. In class, I discussed the "set inclusion" ordering on the subsets of the con-sumption setC=Rn+:Show in the above setting the budget sets get "smaller"under set inclusion assuming either component ofPincreases, ormdecreases.c. Show that the imposition of positive sale tax of good 1 (not good 2) hasthe same impact as a rise in theP1:d. Say the price of good 1 increases from 10 to 20 whenever more than 1unit of good 1 is purchases. Draw the new budget set, and show its convex. Isthe new budge set strictly convex? Explain.2.Preferences. Letdenote the consumers preference relation onC=R2+:Answer the following:a. Sayis reexive, complete, but not transitive. Show that the consumerspreferences could "cycle" (i.e., if forj= 1;2;3;:::;n;and consumption bundlesxnwe could havexjxj1andx0xn:b. Sayis reexive, complete, and transitive.(i) Can indierence curves "cross"?(ii) If so, what additional assumption on preferences rules this out. Also,provide a detailed argument as to why this assumption indeed does rule outcrossing indierence curves.(iii) Show the consumer cannot "cycle" (i.a., part (a) cannot happen in thiscase).(iv) Show that under "strictly monotonic" preferences, indierence curvescannot be "thick".3.Convex Preferences and optimal solutions. Letdenote the consumerspreference relation onC=R2+:We say a preference relationis convex (re-spectively, strictly convex) if for any two bundlesxandysuch thatx~y(i.e.,xandyindierent), then for any2[0;1](respectively,2(0;1)), andz=x+ (1)y; zx~y(respectively,zx~y):We say a preference relationis continuous if the two sets: weakly less preferred:WLP(x) =fy2Cjx1

y;x2Cgand weakly preferred:WP(x) =fy2Cjyx; x2Cgare "closed"(i.e., contain their boundaries. See discussion in class.Answer the following questions. Let the consumption set beC=R2+:(a) Show ifis convex,WP(x)convex.(b) Show ifis strictly convex,WP(x)is strictly convex.(c) IsWLP(x)convex?Consider a consumer facing a budget setB(p;m) =fx2Cjpxmgforp >>0:Dene the best choice setX(p:m) =fx2Cjxxfor allx2B(p;m)g(d) Show ifis convex,X(p;m)might have many elements (i.e., manyoptimal demand choices).(e) Show ifis strictly convex,X(p;m)is a unique for each price-incomepair.4. Say we have a utility functionu(x) =x1x12for2(0;1):(a) Construct the Marginal rate of substitution.(b) Discuss how the Marginal rate of substitution is related to the slope ofan indierence curve at a pointx >>0(i.e., each component ofxis strictlypositive).5. Answer the following:(i) Why is a utility function considered to be an "ordinal" concept?(ii) Show that ifu(x)represents a consumers preference relation, any^u(x) =10u(x)represents that same utility function.(iii) In question 4, of show that ifu(x1;x2)=x1x(1)2for2(0;1);theMRS between the two goods for^u(x)is not impacted by this strictly increasingtransformation.(iv) Actually, show ifh(y) :R!Ris a strictly increasing continuouslydierentiable transformation,^u(x) =h(u(x))represents that same preferencesasu(x).(v) Show in part (iv) that the MRS at the same for both^u(x)andu(x)assumingx >>0(all components ofxare strictly positive). Can you showactually for allx0;the preferences are the same for^u(x)andu(x)? (hint:answer is yes, but do not use the MRS).(vi) Leth(y) = lny:Forx >>0;show the MRS is the same for both^u(x)andu(x)ifu(x1;x2)=x1x(1)2for2(0;1):

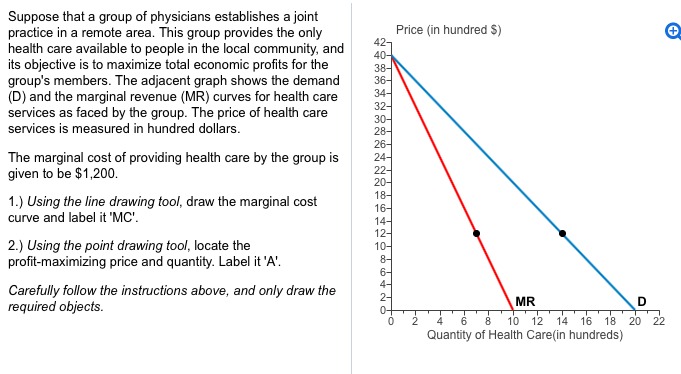
Question 3.

Suppose that a group of physicians establishes a joint practice in a remote area. This group provides the only Price (in hundred $) + health care available to people in the local community, and 42- 40- its objective is to maximize total economic profits for the 38- group's members. The adjacent graph shows the demand 36-1 (D) and the marginal revenue (MR) curves for health care 34- services as faced by the group. The price of health care 32- 30- services is measured in hundred dollars. 28- 26- The marginal cost of providing health care by the group is 24- given to be $1,200. 22- 20- 1.) Using the line drawing tool, draw the marginal cost 18- 16- curve and label it 'MC' 14- 12- 2.) Using the point drawing tool, locate the 10- profit-maximizing price and quantity. Label it 'A'. 8- Carefully follow the instructions above, and only draw the required objects. MR D 2 4 6 8 10 12 14 16 18 20 22 Quantity of Health Care(in hundreds)(55 points) The inverse demand function a monopoly faces is P = 100 -Q. The firm's cost curve is TC(Q) = 10 +5Q (a) (4 points) What is the monopolist's marginal revenue curve? (b) (3 points) What is the monopolist's marginal cost curve? (c) (4 points) What level of output maximizes the monopolist's profits? (d) (4 points) What is the price charged by a profit maximizing monopolist? (e) (4 points) How would your answers to parts (a)- (d) change if TC(Q) = 100 + 5Q? (f) (4 points) For what value of fixed costs, does the monopolist break even? (g) (4 points) For what value of fixed costs, would be monopolist find it optimal to shut down in the short-run? (h) (4 points) For what value of fixed costs, would be monopolist find it optimal to shut down in the long-run? (i) (4 points) What is the value of Lerner Index at the profit maximizing level of output