Parts 3 & 4 only!!

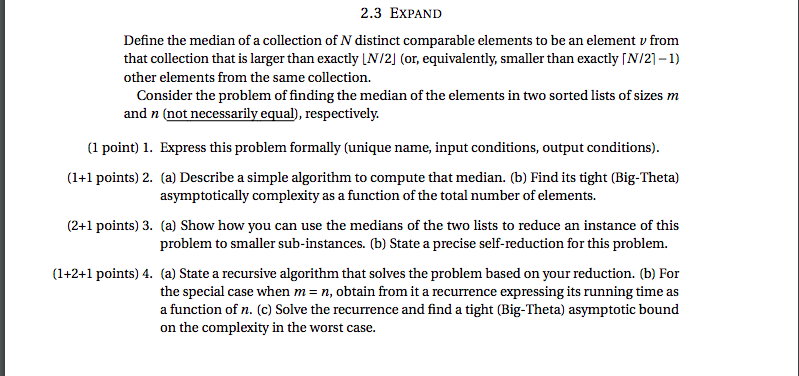
2.3 EXPAND Define the median of a collection of N distinct comparable elements to be an element v from that collection that is larger than exactly [N/2] (or, equivalently, smaller than exactly [N/21 - 1) other elements from the same collection. Consider the problem of finding the median of the elements in two sorted lists of sizes m and n (not necessarily equal), respectively. (1 point) 1. Express this problem formally (unique name, input conditions, output conditions). (1+1 points) 2. (a) Describe a simple algorithm to compute that median. (b) Find its tight (Big-Theta) asymptotically complexity as a function of the total number of elements. (2+1 points) 3. (a) Show how you can use the medians of the two lists to reduce an instance of this problem to smaller sub-instances. (b) State a precise self-reduction for this problem. (1+2+1 points) 4. (a) State a recursive algorithm that solves the problem based on your reduction. (b) For the special case when m= n, obtain from it a recurrence expressing its running time as a function of n. (c) Solve the recurrence and find a tight (Big-Theta) asymptotic bound on the complexity in the worst case. 2.3 EXPAND Define the median of a collection of N distinct comparable elements to be an element v from that collection that is larger than exactly [N/2] (or, equivalently, smaller than exactly [N/21 - 1) other elements from the same collection. Consider the problem of finding the median of the elements in two sorted lists of sizes m and n (not necessarily equal), respectively. (1 point) 1. Express this problem formally (unique name, input conditions, output conditions). (1+1 points) 2. (a) Describe a simple algorithm to compute that median. (b) Find its tight (Big-Theta) asymptotically complexity as a function of the total number of elements. (2+1 points) 3. (a) Show how you can use the medians of the two lists to reduce an instance of this problem to smaller sub-instances. (b) State a precise self-reduction for this problem. (1+2+1 points) 4. (a) State a recursive algorithm that solves the problem based on your reduction. (b) For the special case when m= n, obtain from it a recurrence expressing its running time as a function of n. (c) Solve the recurrence and find a tight (Big-Theta) asymptotic bound on the complexity in the worst case