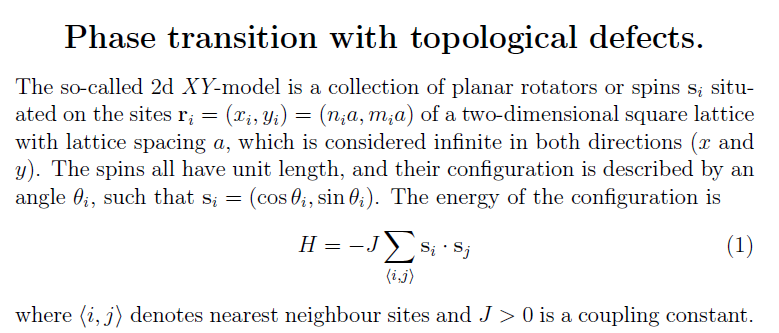
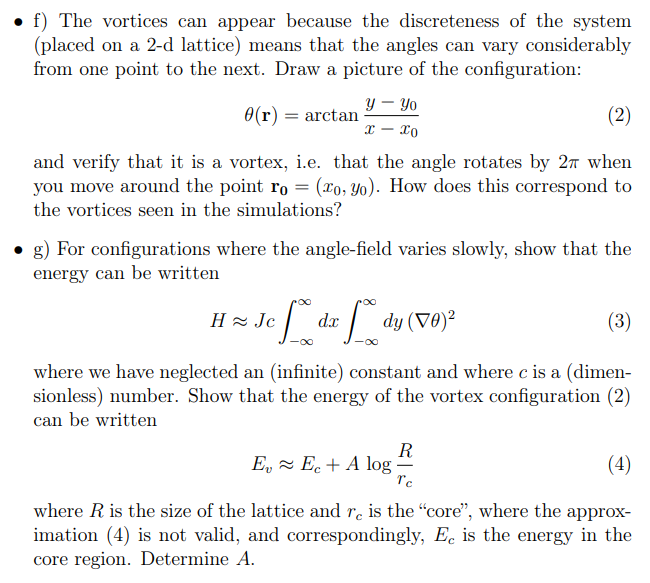
Phase transition with topological defects. The so-called 2d XY-model is a collection of planar rotators or spins si situ- ated on the sites r; = (Li, Yi) = (nja, m;a) of a two-dimensional square lattice with lattice spacing a, which is considered infinite in both directions (x and y). The spins all have unit length, and their configuration is described by an angle i, such that si = (cos6i, sin i). The energy of the configuration is ; H=-1s; s; (1) (ij) where (i, j) denotes nearest neighbour sites and J > 0 is a coupling constant. siis = f) The vortices can appear because the discreteness of the system (placed on a 2-d lattice) means that the angles can vary considerably from one point to the next. Draw a picture of the configuration: y - Yo 0(r) = arctan (2) 2- LO and verify that it is a vortex, i.e. that the angle rotates by 21 when you move around the point ro = (10, yo). How does this correspond to the vortices seen in the simulations? g) For configurations where the angle-field varies slowly, show that the energy can be written HJC dx Ldy (50) (10 (3) where we have neglected an (infinite) constant and where c is a (dimen- sionless) number. Show that the energy of the vortex configuration (2) can be written R E, Ec + A log where R is the size of the lattice and re is the "core", where the approx- imation (4) is not valid, and correspondingly, Ec is the energy in the core region. Determine A. Phase transition with topological defects. The so-called 2d XY-model is a collection of planar rotators or spins si situ- ated on the sites r; = (Li, Yi) = (nja, m;a) of a two-dimensional square lattice with lattice spacing a, which is considered infinite in both directions (x and y). The spins all have unit length, and their configuration is described by an angle i, such that si = (cos6i, sin i). The energy of the configuration is ; H=-1s; s; (1) (ij) where (i, j) denotes nearest neighbour sites and J > 0 is a coupling constant. siis = f) The vortices can appear because the discreteness of the system (placed on a 2-d lattice) means that the angles can vary considerably from one point to the next. Draw a picture of the configuration: y - Yo 0(r) = arctan (2) 2- LO and verify that it is a vortex, i.e. that the angle rotates by 21 when you move around the point ro = (10, yo). How does this correspond to the vortices seen in the simulations? g) For configurations where the angle-field varies slowly, show that the energy can be written HJC dx Ldy (50) (10 (3) where we have neglected an (infinite) constant and where c is a (dimen- sionless) number. Show that the energy of the vortex configuration (2) can be written R E, Ec + A log where R is the size of the lattice and re is the "core", where the approx- imation (4) is not valid, and correspondingly, Ec is the energy in the core region. Determine A








