please address these questions effectively

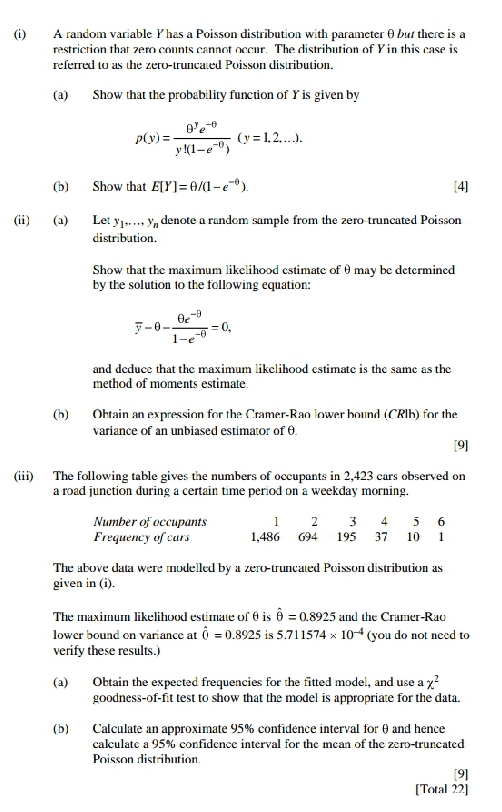
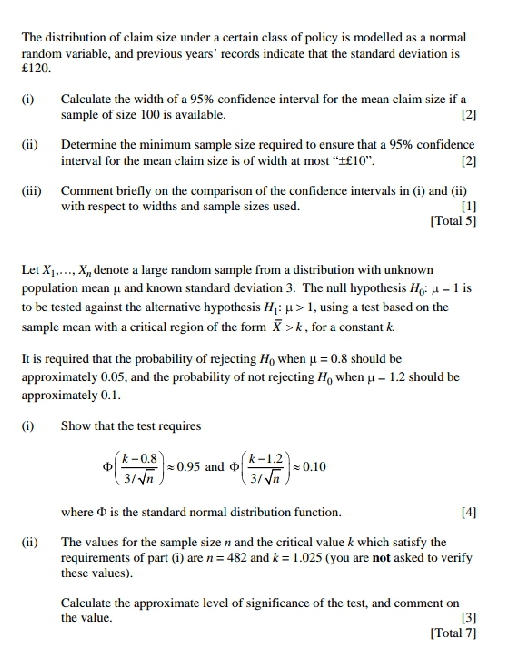
A random variable I has a Poisson distribution with parameter 0 bur there is a restriction that zero counts cannot occur. The distribution of Y in this case is referred to as the zero-truncated Poisson distribution. (a) Show that the probability function of Y' is given by PO)=- ( )=1.2....). y!(1-e-0) (b) Show that E[Y]= 0/(1-e-"). [4] (Hi) (a) Let y1..... ya denote a random sample from the zero-truncated Poisson distribution. Show that the maximum likelihood estimate of 9 may be determined by the solution to the following equation: 1-e-0 and deduce that the maximum likelihood estimate is the same as the method of moments estimate. (h) Obtain an expression for the Cramer-Ran lower hound (CRIb) for the variance of an unbiased estimator of 0 [9] (iii) The following table gives the numbers of occupants in 2,423 cars observed on a road junction during a certain time period on a weekday morning. Number of occupants 2 3 4 5 6 Frequency of cars 1,486 195 37 10 The above data were modelled by a zero-truncated Poisson distribution as given in (i). The maximum likelihood estimate of 6 is 0 = 0.8925 and the Cramer-Rao lower bound on variance at () = 0.8925 is 5.711574 x 10 4 (you do not need to verify these results.) (a) Obtain the expected frequencies for the fitted model, and use a y goodness-of-fit test to show that the model is appropriate for the data. (b) Calculate an approximate 95% confidence interval for ( and hence calculate a 95% confidence interval for the mean of the zero-truncated Poisson distribution. [9] [Total 22]The distribution of claim size under a certain class of policy is modelled as a normal random variable, and previous years' records indicate that the standard deviation is (120. (1) Calculate the width of a 95 confidence interval for the mean claim size if a sample of size 100 is available. 121 (ii) Determine the minimum sample size required to ensure that a 95% confidence interval for the mean claim size is of width at most "If10". [2] (iii) Comment briefly on the comparison of the confidence intervals in (i) and (ii) with respect to widths and sample sizes used. [1] [Total 5] Let X1...; Xx denote a large random sample from a distribution with unknown population mean p and known standard deviation 3. The null hypothesis Ho: 4 - 1 is to be tested against the alternative hypothesis /1: > 1, using a test based on the sample mean with a critical region of the form X > k, for a constant A. It is required that the probability of rejecting Ho when u = 0.8 should be approximately 0.05, and the probability of not rejecting Ho when u - 1.2 should be approximately 0.1. (1) Show that the test requires A -0.8 =0.95 and