.. please address this

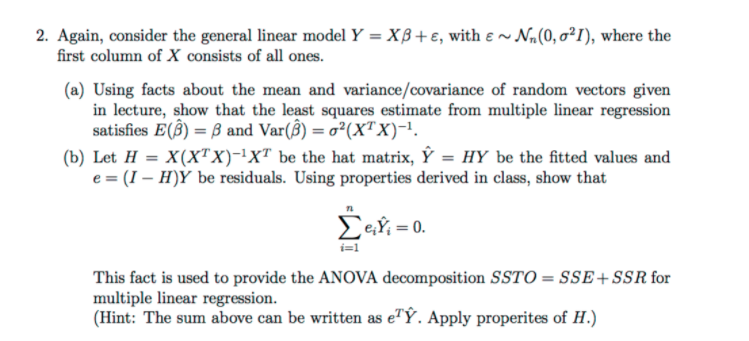
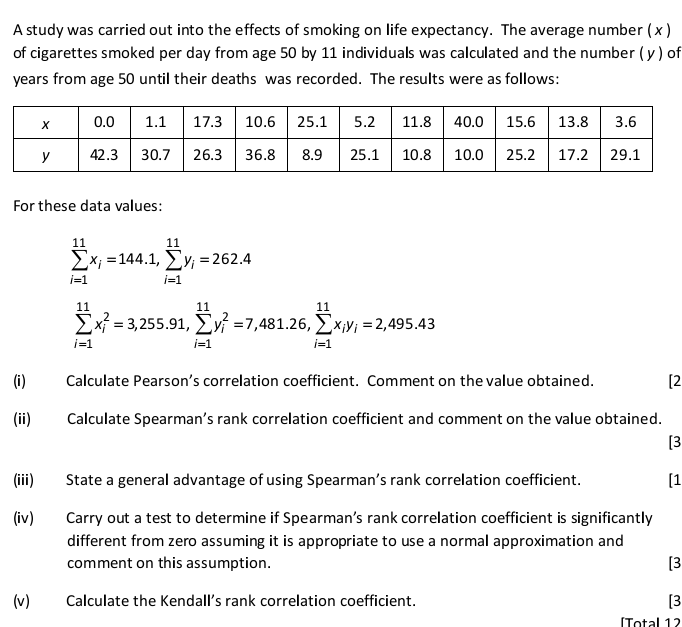
1. Consider the simple regression model: V/i = Po+ Piri +ui, for i = 1, ..., n, with E(ur,) 7 0 and let z be a dummy instrumental variable for a, such that we can write: with E(uilz;) = 0 and E(vilzi) = 0. (c) Denote by no, the number of observations for which z =0 and by n, the number of observations for which z, = 1. Show that: (a - 2) = =(n-m). 1=1 and that: [(8 -=)(3: - 9) = 7 "(n - ni) (31 - 90) . where to and g are the sample means of y for z equal to 0 and 1 respectively. (Hint: Use the fact that n = nj + no, and that = = m). (d) Now we regress y on i to obtain an estimator of 81. From the standard formula of the slope estimator for an OLS regression and using the result in (c), show that: By1 - 90 I1 - To This estimator is called the Wald estimator.2. Again, consider the general linear model Y = XB + , with & ~ Nn(0, o?/), where the first column of X consists of all ones. (a) Using facts about the mean and variance/covariance of random vectors given in lecture, show that the least squares estimate from multiple linear regression satisfies E(B) = B and Var(B) = 03(XTX)-1. (b) Let H = X(X X)-1XT be the hat matrix, Y = HY be the fitted values and e = (I - H)Y be residuals. Using properties derived in class, show that n Ex = 0. i=1 This fact is used to provide the ANOVA decomposition SSTO = SSE + SSR for multiple linear regression. (Hint: The sum above can be written as e Y. Apply properites of H.)5. There are three individuals in an economy. The demand functions for these individuals are given as follows: X1 = 22-2P. X2 = 8-P, X3 = 28-2P. What is the market demand when P = 10? (a) X = 30 - 3P (b) X = 50 - 4P (c) X = 28 - 2P (d) X = 36 - 3P (e) X - 58 - 5PA study was carried out into the effects of smoking on life expectancy. The average number ( x ) of cigarettes smoked per day from age 50 by 11 individuals was calculated and the number ( y ) of years from age 50 until their deaths was recorded. The results were as follows: X 0.0 1.1 17.3 10.6 25.1 5.2 11.8 40.0 15.6 13.8 3.6 y 42.3 30.7 26.3 36.8 8.9 25.1 10.8 10.0 25.2 17.2 29.1 For these data values: 11 11 x; =144.1, > y, =262.4 /=1 1=1 11 11 x, = 3,255.91, Ev, =7,481.26, _x,y; =2,495.43 11 /=1 1=1 Calculate Pearson's correlation coefficient. Comment on the value obtained. [2 (ii) Calculate Spearman's rank correlation coefficient and comment on the value obtained. [3 (iii) State a general advantage of using Spearman's rank correlation coefficient. [1 (iv) Carry out a test to determine if Spearman's rank correlation coefficient is significantly different from zero assuming it is appropriate to use a normal approximation and comment on this assumption. [3 (v) Calculate the Kendall's rank correlation coefficient. [3 1 17