Please answer the following two questions. Thanks.

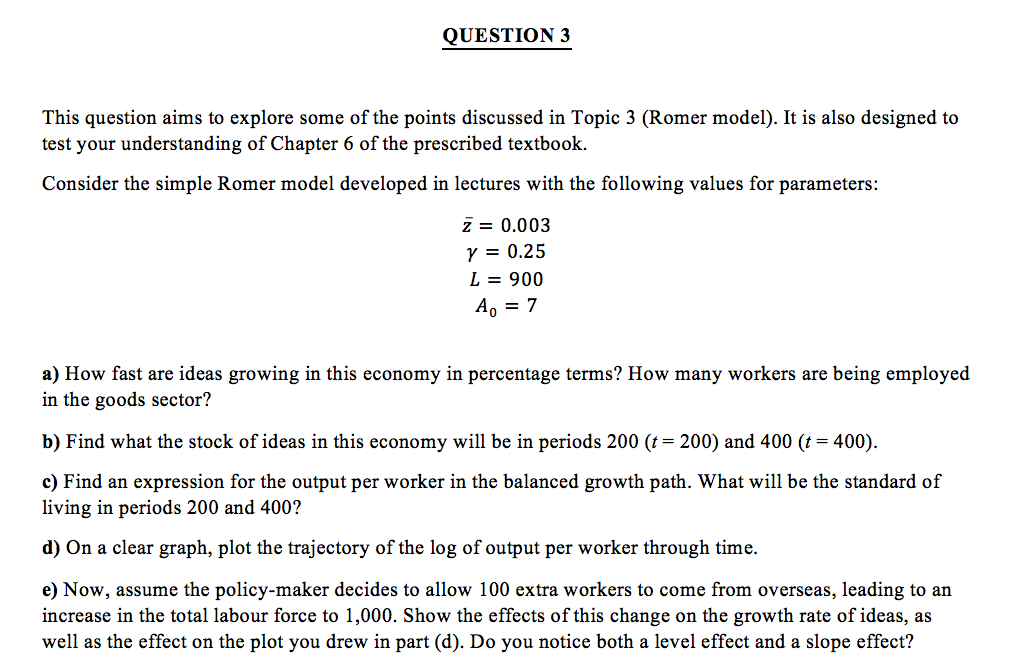
QUESTION 2 This question aims to explore some of the points discussed in Topic 2 (Solow-Swan model). It is also designed to test your understanding of Chapter 5 of the prescribed textbook. Consider an economy with the general Cobb-Douglas production function: Y, = month\". Answer the following questions assuming that labour grows at the rate n = 0 and adopting the assumptions made in lecture. The equation describing capital dynamics is: Km = K, + It th where a' is a constant parameter. a) Obtain the steady state levels of the capital stock (K), output (Y), capital per worker (k), output per worker (y), consumption per worker (C/L), total savings (S), private investment (I), real wages (w) and real interest rates (r). Make sure to show all your work. b) Assuming that s = 0.4, d = 0.1, u = 0.4 and Al = I, calculate the steady state values of the variables in (a). Now, assume the policy-maker successfully implemented a policy that resulted in the increase of the total factor productivity level to 2 (i.e., A = 2), ceteris paribus. c) Using the help of the Solow-Swan diagram developed in lectures and tutorials, explain the effect of this policy on the standard of living of the economy. Make sure to include: i) The economic explanation of why the economy experienced a change in the steady state level of capital per worker; ii) A diagram illustrating what happened to the relevant curves. iii) Another diagram illustrating the dynamics of the stock of capital in the economy (before, at and after the technological shock). iv) What must have happened to the growth rate of output per worker in the transition between the initial equilibrium and the nal one? c) Is the current savings rate (3 = 0.4) the golden rule of savings? Hint: you don't need to calculate the actual golden rule, just to show that the current one is not the golden rule. QUESTION 3 This question aims to explore some of the points discussed in Topic 3 (Romer model). It is also designed to test your understanding of Chapter 6 of the prescribed textbook. Consider the simple Romer model developed in lectures with the following values for parameters: E = 0.003 r = 0.25 L = 900 AI, = 7 a) How fast are ideas growing in this economy in percentage terms? How many workers are being employed in the goods sector? b) Find what the stock of ideas in this economy will be in periods 200 (r = 200) and 400 (t = 400). c) Find an expression for the output per worker in the balanced growth path. What will be the standard of living in periods 200 and 400? d) On a clear graph, plot the trajectory of the log of output per worker through time. e) Now, assume the policy-maker decides to allow 100 extra workers to come from overseas, leading to an increase in the total labour force to 1,000. Show the effects of this change on the growth rate of ideas, as well as the effect on the plot you drew in part (d). Do you notice both a level effect and a slope effect