please anwser all 7 questions! Thank you!
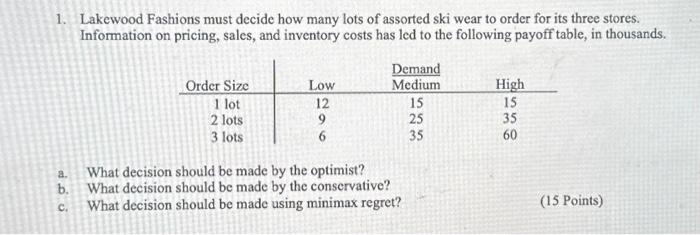
1. Lakewood Fashions must decide how many lots of assorted ski wear to order for its three stores. Information on pricing, sales, and inventory costs has led to the following payoff table, in thousands. a. What decision should be made by the optimist? b. What decision should be made by the conservative? c. What decision should be made using minimax regret? (15 Points) 2. A Pacific Northwest lumber company is considering the expansion of one of its mills. The question is whether to do it now, or wait for one year and re-consider. If they expand now, the major factors of importance are the state of the economy and the level of interest rates. The combination of these two factors results in five possible situations. If they do not expand now, only the state of the economy is important and three conditions characterize the possibilities. The following table summarizes the situation: (15 Points) a. Draw the decision tree for this problem. b. What is the expected value for expanding? c. What is the expected value for not expanding? d. Based on expected value, what should the company's decision(s) be? 3. For the payoff table below, the decision maker will use P(s1)=.15,P(s2)=.5, and P(s3)=.35. a. What alternative would be chosen according to expected value? b. For a lottery having a payoff of 40,000 with probability p and 15,000 with probability (1p), the decision maker expressed the following indifference probabilities. Let U(40,000)=10 and U(15,000)=0 and find the utility value for each payoff. c. What alternative would be chosen according to expected utility? (15 Points) 4. Consider the following two-person zero-sum game. Assume the two players have the same two strategy options. The payoff table shows the gains for Player A. Determine the optimal strategy for each player. What is the value of the game? (15 Points) 5. Use a four-period moving average to calculate MFE, MAE, MSE, and MAPE. Historical records show: (15 points) 5346,7812,6513,5783,5982,6519,6283,5577,6712,7345 6. The average SAT verbal score for students from one high school over the last ten exams is 508,490,502,505,493,506,492,490,503,501 Estimate the parameter (b0,b1) of the linear trend equation: T=b0+b1t, where T= trend value of the time series in period t. (15 Points) 7. Quarterly billing for water usage is shown below. The forecast equation is: Ft=64.8756.1375Qtr1t+32.325Qtr2t+86.0375Qtr3t+1.2875(t). Forecast the summer of year 5 and spring of year 6. (10 Points)













