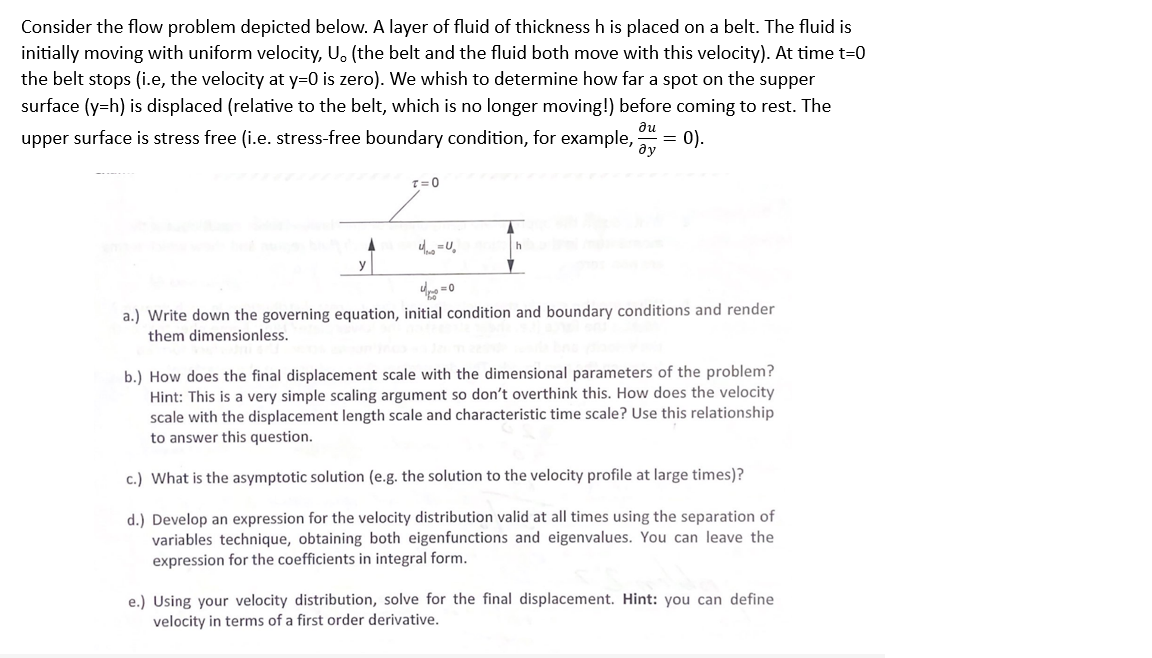
Please don't use ChatGPT to solve this problem. I prefer a handwriting style.

Consider the flow problem depicted below. A layer of fluid of thickness h is placed on a belt. The fluid is initially moving with uniform velocity, Uo (the belt and the fluid both move with this velocity). At time t=0 the belt stops (i.e, the velocity at y=0 is zero). We whish to determine how far a spot on the supper surface (y=h) is displaced (relative to the belt, which is no longer moving!) before coming to rest. The upper surface is stress free (i.e. stress-free boundary condition, for example, yu=0 ). a.) Write down the governing equation, initial condition and boundary conditions and render them dimensionless. b.) How does the final displacement scale with the dimensional parameters of the problem? Hint: This is a very simple scaling argument so don't overthink this. How does the velocity scale with the displacement length scale and characteristic time scale? Use this relationship to answer this question. c.) What is the asymptotic solution (e.g. the solution to the velocity profile at large times)? d.) Develop an expression for the velocity distribution valid at all times using the separation of variables technique, obtaining both eigenfunctions and eigenvalues. You can leave the expression for the coefficients in integral form. e.) Using your velocity distribution, solve for the final displacement. Hint: you can define velocity in terms of a first order derivative