please graph all the data. thank you!
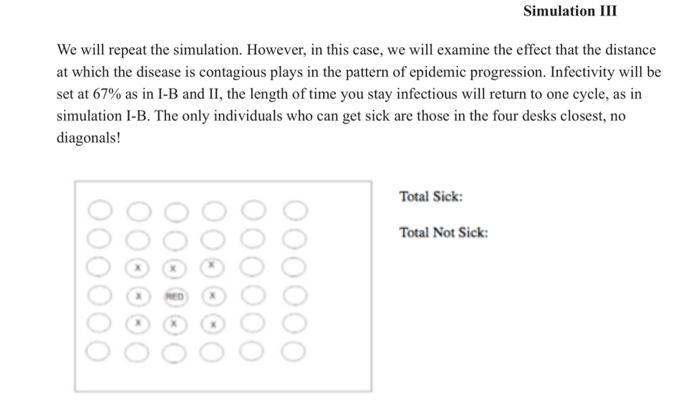
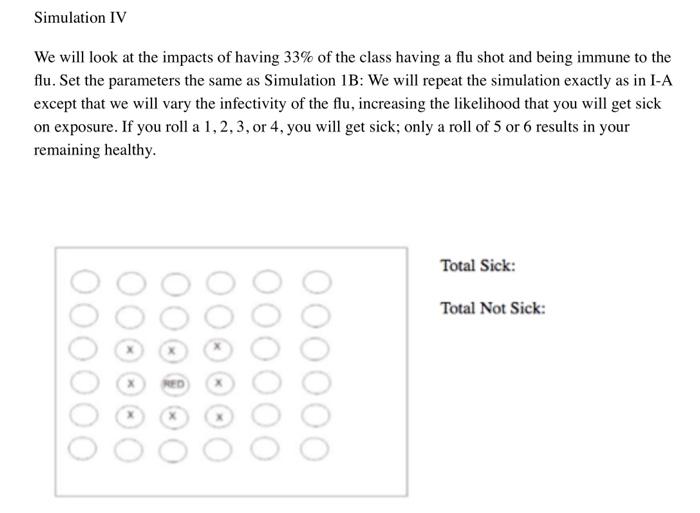
Simulation 1 The infectious time frame will be one cycle (1 roll). If you roll a l or a 2, sorryyou get the flu and should stack your cup so that red is on the outside. If you roll a 3, 4, 5, or 6, you were lucky enough not to get the flu on this exposure. Keep track of sick and not sick Total Sick: Total Not Sick: RED Simulation I-B We will repeat the simulation exactly as in I-A except that we will vary the infectivity of the flu, increasing the likelihood that you will get sick on exposure. If you roll a 1, 2, 3, or 4, you will get sick; only a roll of 5 or 6 results in your remaining healthy. Total Sick: Total Not Sick: X RED X Simulation II We will repeat the simulation. However, in this case, we will examine the effect that length of contagion has on the pattern of epidemic progression. The infectivity will again be set at 67% (i.e., a roll of 1, 2, 3, or 4 means you get the flu, a 5 or 6 means you stay healthy). Once you get the flu, you stay infectious for three cycles (You need to roll the dice 3 times and get a 5 or 6, 3 times in a row. Total Sick: Total Not Sick: Simulation III We will repeat the simulation. However, in this case, we will examine the effect that the distance at which the disease is contagious plays in the pattern of epidemic progression. Infectivity will be set at 67% as in 1-B and II, the length of time you stay infectious will return to one cycle, as in simulation I-B. The only individuals who can get sick are those in the four desks closest, no diagonals! Total Sick: Total Not Sick: Simulation IV We will look at the impacts of having 33% of the class having a flu shot and being immune to the flu. Set the parameters the same as Simulation 1B: We will repeat the simulation exactly as in I-A except that we will vary the infectivity of the flu, increasing the likelihood that you will get sick on exposure. If you roll a 1, 2, 3, or 4, you will get sick; only a roll of 5 or 6 results in your remaining healthy. Total Sick: Total Not Sick: Simulation 1 The infectious time frame will be one cycle (1 roll). If you roll a l or a 2, sorryyou get the flu and should stack your cup so that red is on the outside. If you roll a 3, 4, 5, or 6, you were lucky enough not to get the flu on this exposure. Keep track of sick and not sick Total Sick: Total Not Sick: RED Simulation I-B We will repeat the simulation exactly as in I-A except that we will vary the infectivity of the flu, increasing the likelihood that you will get sick on exposure. If you roll a 1, 2, 3, or 4, you will get sick; only a roll of 5 or 6 results in your remaining healthy. Total Sick: Total Not Sick: X RED X Simulation II We will repeat the simulation. However, in this case, we will examine the effect that length of contagion has on the pattern of epidemic progression. The infectivity will again be set at 67% (i.e., a roll of 1, 2, 3, or 4 means you get the flu, a 5 or 6 means you stay healthy). Once you get the flu, you stay infectious for three cycles (You need to roll the dice 3 times and get a 5 or 6, 3 times in a row. Total Sick: Total Not Sick: Simulation III We will repeat the simulation. However, in this case, we will examine the effect that the distance at which the disease is contagious plays in the pattern of epidemic progression. Infectivity will be set at 67% as in 1-B and II, the length of time you stay infectious will return to one cycle, as in simulation I-B. The only individuals who can get sick are those in the four desks closest, no diagonals! Total Sick: Total Not Sick: Simulation IV We will look at the impacts of having 33% of the class having a flu shot and being immune to the flu. Set the parameters the same as Simulation 1B: We will repeat the simulation exactly as in I-A except that we will vary the infectivity of the flu, increasing the likelihood that you will get sick on exposure. If you roll a 1, 2, 3, or 4, you will get sick; only a roll of 5 or 6 results in your remaining healthy. Total Sick: Total Not Sick