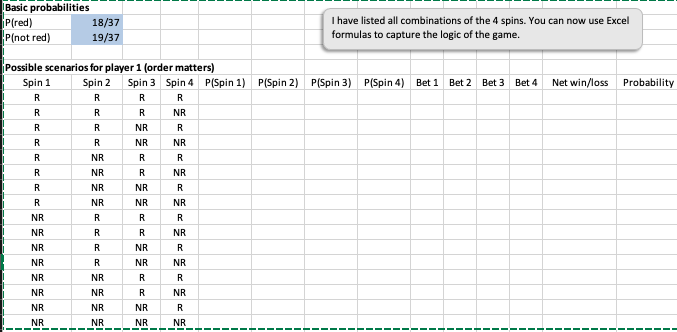
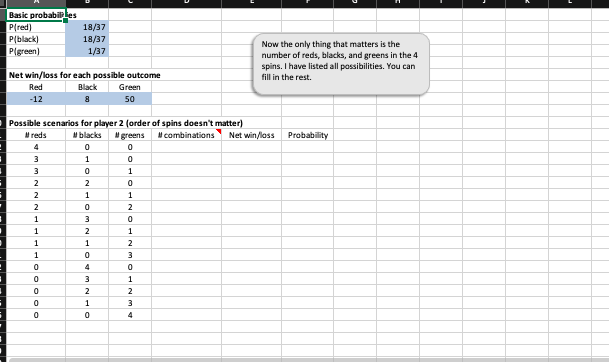
please help. d o not understand

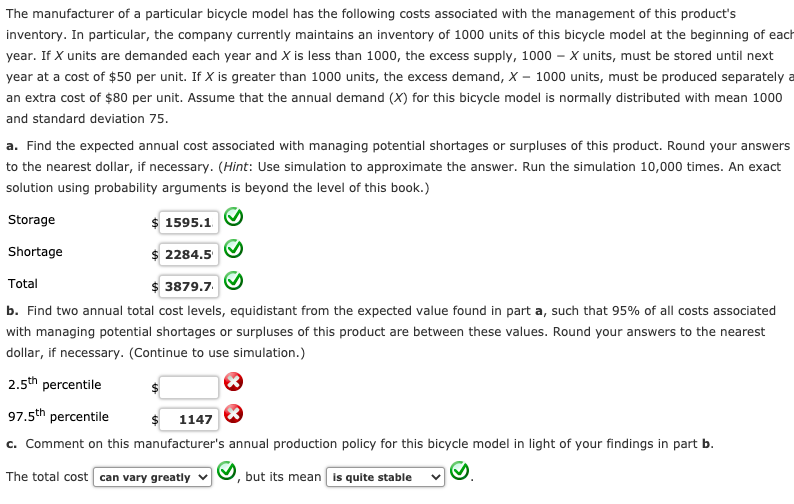
The manufacturer of a particular bicycle model has the following costs associated with the management of this product's inventory. In particular, the company currently maintains an inventory of 1000 units of this bicycle model at the beginning of each year. If X units are demanded each year and X is less than 1000, the excess supply, 1000 - X units, must be stored until next year at a cost of $50 per unit. If X is greater than 1000 units, the excess demand, X - 1000 units, must be produced separately an extra cost of $80 per unit. Assume that the annual demand (X) for this bicycle model is normally distributed with mean 1000 and standard deviation 75. a. Find the expected annual cost associated with managing potential shortages or surpluses of this product. Round your answers to the nearest dollar, if necessary. (Hint: Use simulation to approximate the answer. Run the simulation 10,000 times. An exact solution using probability arguments is beyond the level of this book.) Storage 1595.1 Shortage $ 2284.5 Total $ 3879.7 b. Find two annual total cost levels, equidistant from the expected value found in part a, such that 95% of all costs associated with managing potential shortages or surpluses of this product are between these values. Round your answers to the nearest dollar, if necessary. (Continue to use simulation.) 2.5" percentile 97.5th percentile 1147 c. Comment on this manufacturer's annual production policy for this bicycle model in light of your findings in part b. The total cost can vary greatly v but its mean is quite stableBasic probabilities iP (red) 18/37 I have listed all combinations of the 4 spins. You can now use Excel P(not red) 19/37 formulas to capture the logic of the game. Possible scenarios for player 1 (order matters) Spin 1 Spin 2 Spin 3 Spin 4 P(Spin 1) P(Spin 2) P(Spin 3) P(Spin 4) Bet 1 Bet 2 Bet 3 Bet 4 R Net win/loss R Probability R R R R R NR R R NR R R R NR NR R NR R R R NR R NR R NR NR R R NR NR NR NR R R R NR R R NR NR R NR R NR R NR NR NR NR R R NR NR R NR NR NR NR R NR NR NR NRBasic probabil les P/red] 18/37 P(black] 18/37 Now the only thing that matters is the P(green] 1/37 number of reds, blacks, and greens in the 4 spins. I have listed all possibilities. You can Net win/loss for each possible outcome fill in the rest. Red Black Green -12 50 Possible scenarios for player 2 (order of spins doesn't matter) U reds # blacks greens # combinations * Net win/loss Probability 4 0 1