PLEASE HELP ME ANSWER THIS QUESTION. THANK YOU SO MUCH !!

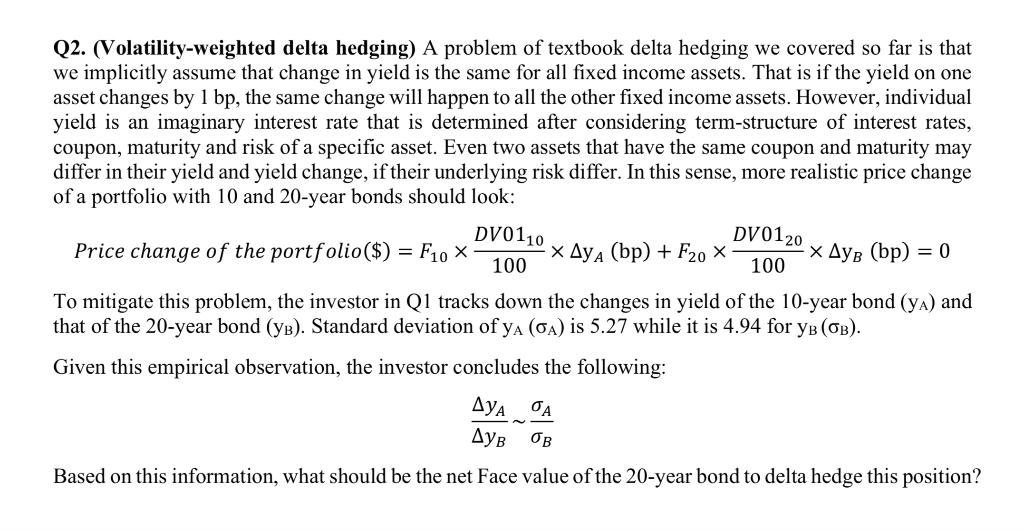
Q2. (Volatility-weighted delta hedging) A problem of textbook delta hedging we covered so far is that we implicitly assume that change in yield is the same for all fixed income assets. That is if the yield on one asset changes by 1 bp, the same change will happen to all the other fixed income assets. However, individual yield is an imaginary interest rate that is determined after considering term-structure of interest rates, coupon, maturity and risk of a specific asset. Even two assets that have the same coupon and maturity may differ in their yield and yield change, if their underlying risk differ. In this sense, more realistic price change of a portfolio with 10 and 20-year bonds should look: DV0110 Price change of the portfolio($) = F10 x x Aya (bp) + F20 x -x Ays (bp) = 0 DV0120 v AvR (bp) 100 100 To mitigate this problem, the investor in Q1 tracks down the changes in yield of the 10-year bond (YA) and that of the 20-year bond (YB). Standard deviation of yA (GA) is 5.27 while it is 4.94 for YB (6B). Given this empirical observation, the investor concludes the following: Based on this information, what should be the net Face value of the 20-year bond to delta hedge this position? Q2. (Volatility-weighted delta hedging) A problem of textbook delta hedging we covered so far is that we implicitly assume that change in yield is the same for all fixed income assets. That is if the yield on one asset changes by 1 bp, the same change will happen to all the other fixed income assets. However, individual yield is an imaginary interest rate that is determined after considering term-structure of interest rates, coupon, maturity and risk of a specific asset. Even two assets that have the same coupon and maturity may differ in their yield and yield change, if their underlying risk differ. In this sense, more realistic price change of a portfolio with 10 and 20-year bonds should look: DV0110 Price change of the portfolio($) = F10 x x Aya (bp) + F20 x -x Ays (bp) = 0 DV0120 v AvR (bp) 100 100 To mitigate this problem, the investor in Q1 tracks down the changes in yield of the 10-year bond (YA) and that of the 20-year bond (YB). Standard deviation of yA (GA) is 5.27 while it is 4.94 for YB (6B). Given this empirical observation, the investor concludes the following: Based on this information, what should be the net Face value of the 20-year bond to delta hedge this position