Please help me do question (i),(ii),(iii), Thank you a lot!!!

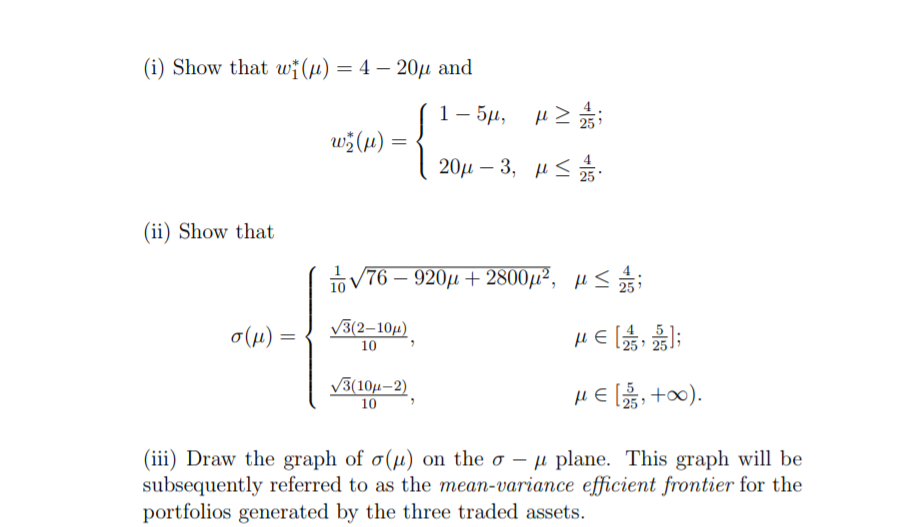
1. Consider a two-period economy, where two risky assets and one riskless asset are traded at date 0 and they generate cash flows at date 1. The rate of return on risky asset j is denoted by r return on the riskless asset is denoted by rf. The date-0 markets for the two risky assets are perfect. However, short sale is prohibited for the riskless asset at date 0 y, j 1.2 The rate of Suppose that rf -0.2, and you are given the following data regarding the two risky assets E0.15 var Ti cov T2,T1 coV T1, T2 var T2 0.01-0.01 0.01 0.04 Let wi, w2, and 1- Wi - W2 be the portfolio weights that you assign to, respectively, the two risky assets, and the riskless asset. Suppose that you wish to have a portfolio of the three traded assets that generates an expected rate of return equal to E R, and you would like to keep the variance of the rate of return on that portfolio as small as possible. Formally, you are asked to solve the following minimization program min. O.01 uf + 2tvMz . (-0.01) + 0.04u subject to Given , let (wi (), w ()) denote the solution to the above minimiza- tion program. Correspondingly, let "() denote the minimized value of the objective function; that is, (A) 0.01 lu' (A)]2 + 2[tv ()][u$(A)] . (-0.01) + 0.04(u$(A)]2 (i) Show that wi (-4-20 and (ii) Show that V32-10 10 25 25J V3(1011-2) 10 25 (iii) Draw the graph of (a) on the - plane. This graph will be subsequently referred to as the mean-variance efficient frontier for the portfolios generated by the three traded assets. 1. Consider a two-period economy, where two risky assets and one riskless asset are traded at date 0 and they generate cash flows at date 1. The rate of return on risky asset j is denoted by r return on the riskless asset is denoted by rf. The date-0 markets for the two risky assets are perfect. However, short sale is prohibited for the riskless asset at date 0 y, j 1.2 The rate of Suppose that rf -0.2, and you are given the following data regarding the two risky assets E0.15 var Ti cov T2,T1 coV T1, T2 var T2 0.01-0.01 0.01 0.04 Let wi, w2, and 1- Wi - W2 be the portfolio weights that you assign to, respectively, the two risky assets, and the riskless asset. Suppose that you wish to have a portfolio of the three traded assets that generates an expected rate of return equal to E R, and you would like to keep the variance of the rate of return on that portfolio as small as possible. Formally, you are asked to solve the following minimization program min. O.01 uf + 2tvMz . (-0.01) + 0.04u subject to Given , let (wi (), w ()) denote the solution to the above minimiza- tion program. Correspondingly, let "() denote the minimized value of the objective function; that is, (A) 0.01 lu' (A)]2 + 2[tv ()][u$(A)] . (-0.01) + 0.04(u$(A)]2 (i) Show that wi (-4-20 and (ii) Show that V32-10 10 25 25J V3(1011-2) 10 25 (iii) Draw the graph of (a) on the - plane. This graph will be subsequently referred to as the mean-variance efficient frontier for the portfolios generated by the three traded assets








