please help me solve and explain along the way! Thank you!!
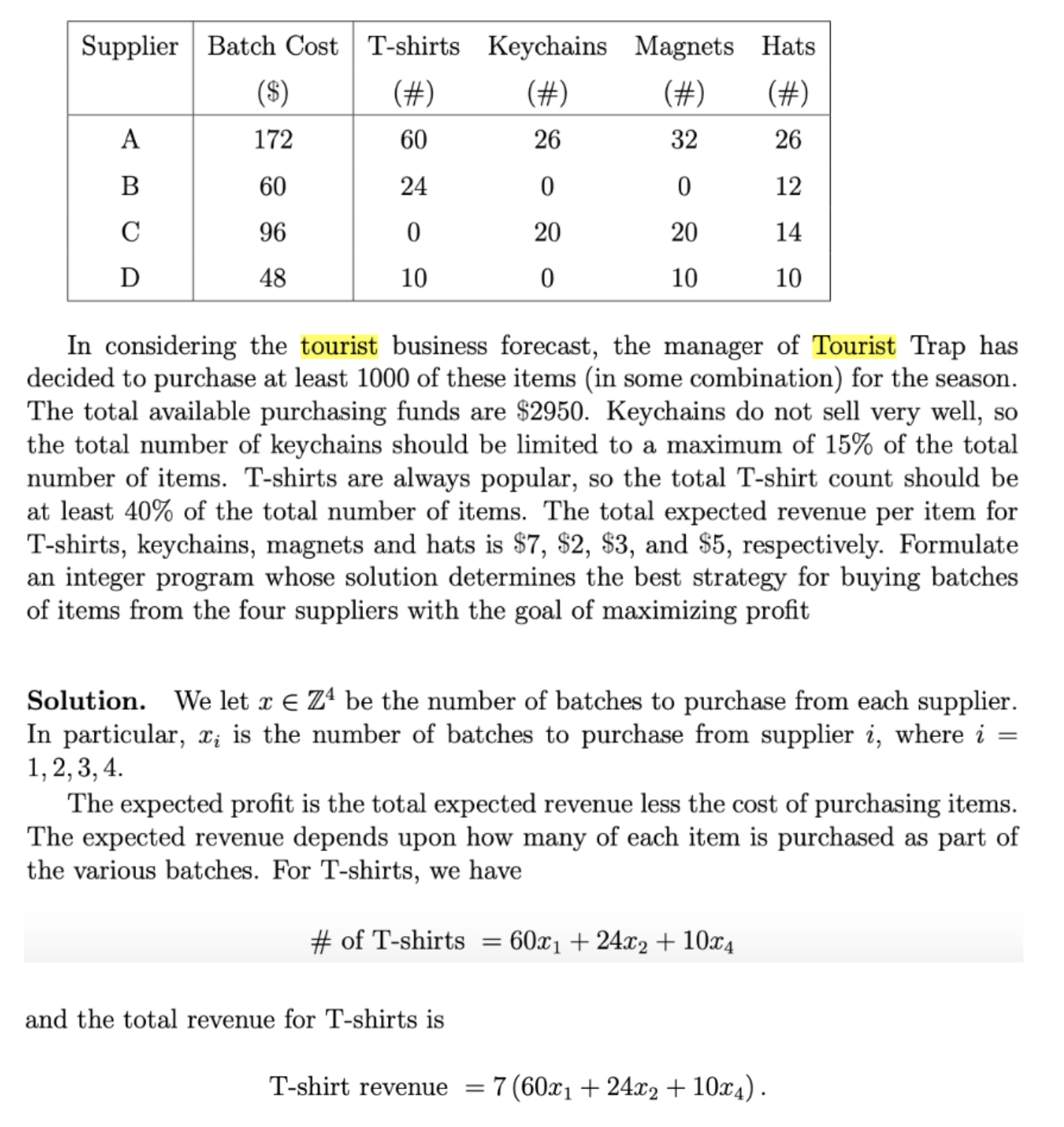
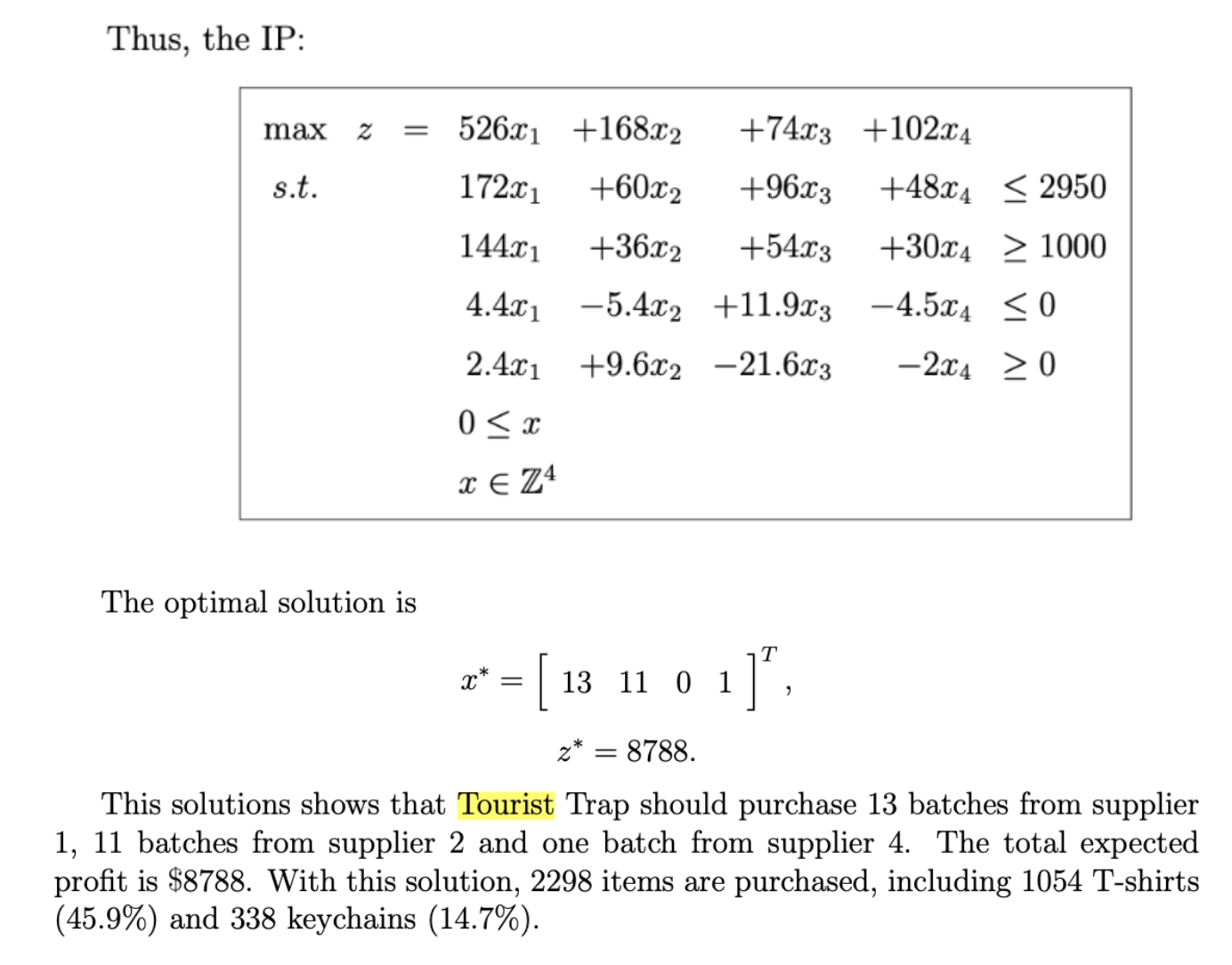
3. Reformulate the 'Iburist Trap problem to include the following additional vari- ables m5 = total number of items purchased 235 = total number of Tshirts purchased x7 = total number of keychains purchased Use these variables to simplify some of the existing constraints, at the expense of adding new constraints. Show that the solution is the same as the original formulation. Why might this modied formulation be preferable? 11.3 Tourist Trap Shop The Tourist Trap Shep survives on the tourist trade selling a variety of trinkets. Among other items, they sell T-shirts, keychains, refrigerator magnets, and hats. They can obtain these items from four different wholesalers which supply these items in xed quantities. The table below shows the quantity of each item found in each supplier's standard shipment. It also shows the wholesale cost of each shipment. Supplier T-shirts Keychains M:gn)ets Hats ($)2 In considering the tourist business forecast, the manager of Tourist Trap has decided to purchase at least 1000 of these items (in some combination) for the season. The total available purchasing funds are $2950. Keychains do not sell very well, so the total number of keychains should be limited to a maximum of 15% of the total number of items. Tshirts are always popular, so the total Tshirt count should be at least 40% of the total number of items. The total expected revenue per item for T-shirts, keychains, magnets and hats is $7, $2, $3, and $5, respectively. Formulate an integer program whose solution determines the best strategy for buying batches of items from the four suppliers with the goal of maximizing prot Solution. We let a: E 2\" be the number of batches to purchase from each supplier. In particular, 2:; is the number of batches to purchase from supplier z', where i = 1, 2, 3, 4. The expected prot is the total expected revenue less the cost of purchasing items. The expected revenue depends upon how many of each item is purchased as part of the various batches. For T-shirts, we have # of T-shirts = 60:51 + 243:2 + 10% and the total revenue for Tshirts is Tshjrt revenue = 7 (602:1 + 249:2 + 102:4) . Similarly, we have Keychajn revenue = 2 (26:31 + 201113) a Magnet revenue = 3 (32x1 + 2011:; + 101:4), Hat revenue = 5 (263:1 + 121:; + 14533 + 103:4) . The total prot, z, is the sum of these individual prots less the cost of batches: Z = 698151 + 228.752 + 170563 + 1501134 - 1721111 60272 - 963:3 - 481171, 2: = 526321 + 1681:; + 743:3 + 102214. Alternatively, one could compute the expected prot per batch from each supplier, arriving at the same prot function. Next, consider the various constraints. First, have purchasing funds limited to $2950. This leads to the constraint: 172331 + 60132 + 9633 -I- 48224 S 2950. Next, we must purchase at least 1000 total items. Each batch contains differing numbers of items. We have 14431 + 36:32 + 543:3 + 302:4 2 1000. The number of keychains should be limited to a maximum of 15% of the total number of items. We have 2ch1 + 2m:3 g (045)0442:1 + 362:2 + 54:1:3 + 30334). 0r, equivalently, 4.43:1 5.4132 + 11.9213 4.534 S 0. Notice, in particular that the total number of purchases items is not necessarily to 1000. Similarly, we have that the total number of T-shirts should be at leas of the total number of items. This leads to the constraint: 2.4151 + 9.65132 21.6333 2234 Z 0. Thus, the IP: The optimal solution is m*=[13 11 01]T, z" = 8788. This solutions shows that Tourist Trap should purchase 13 batches from supplier 1, 11 batches from supplier 2 and one batch from supplier 4. The total expected prot is $8788. With this solution, 2298 items are purchased, including 1054 T-shirts (45.9%) and 338 keychains (14.7%)