Question
Please help me understand this concept. I need explanations on how do these. thank you Q3. Proportions (percentages) in a Z Distribution [10 points] A
Please help me understand this concept. I need explanations on how do these. thank you
Q3. Proportions (percentages) in a Z Distribution [10 points]
A standardized visual working memory test has a population mean (?) of 54 and a standard deviation (?) of 4. Because the scores are normally distributed, the whole distribution of scores can be converted into a Z distribution. Each raw score in the original distribution has a corresponding Z score in the Z distribution. The Z distribution has a symmetrical bell shape with known properties, so it's possible to mathematically figure out the percentage of scores within any specified area in the distribution.
Deduct 0.5 if the calculation process is correct but the result is wrong
| Helpful tips: |
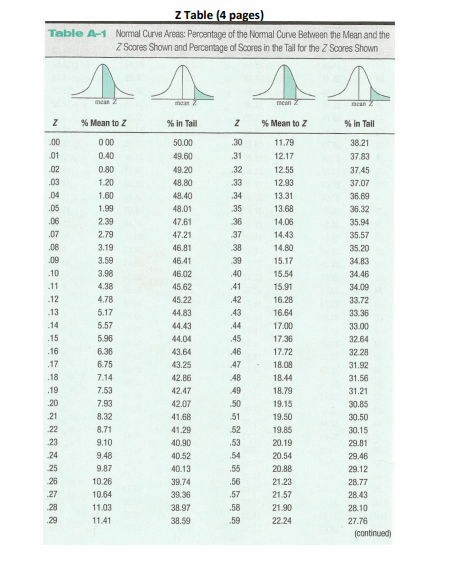
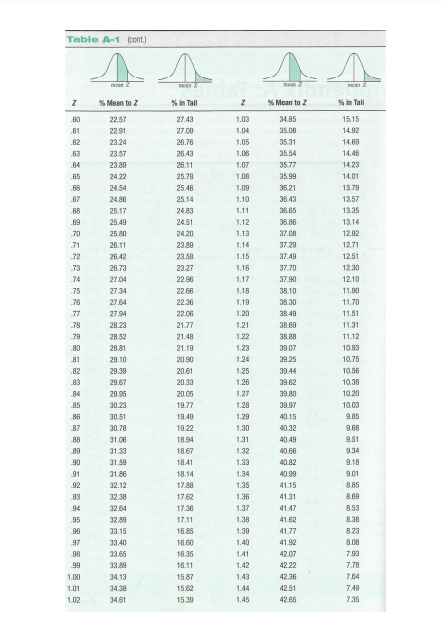
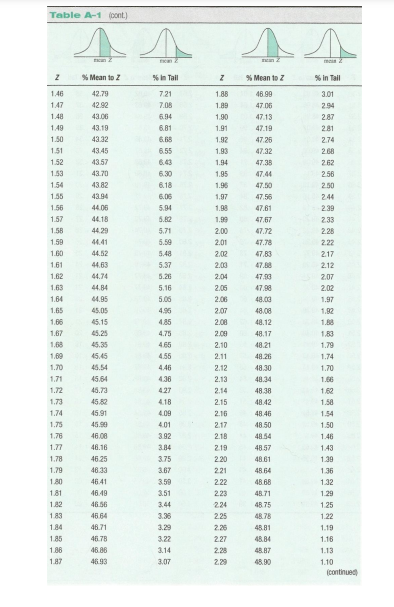
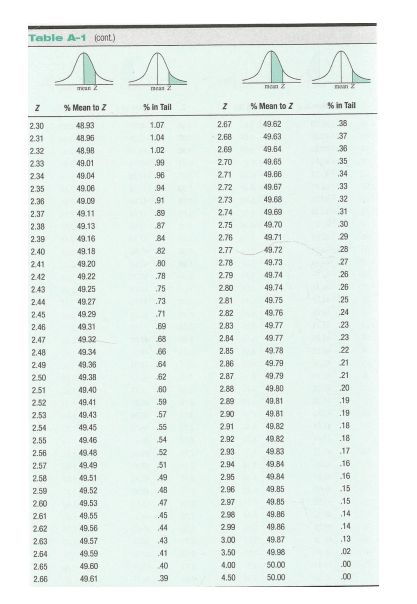
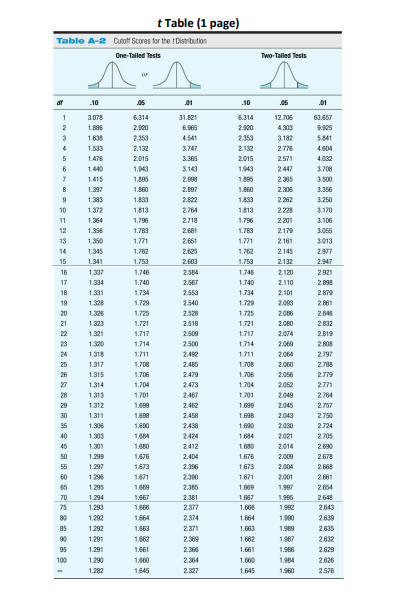
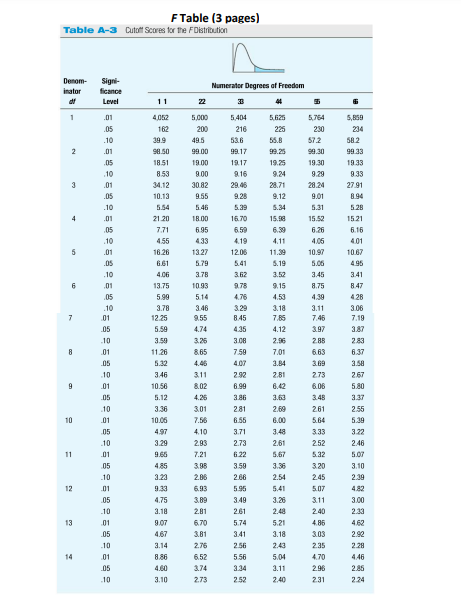
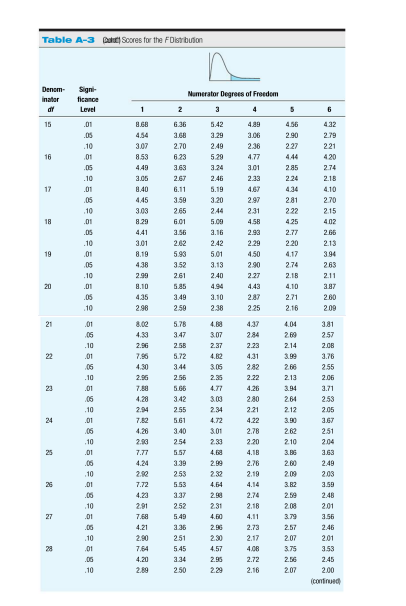
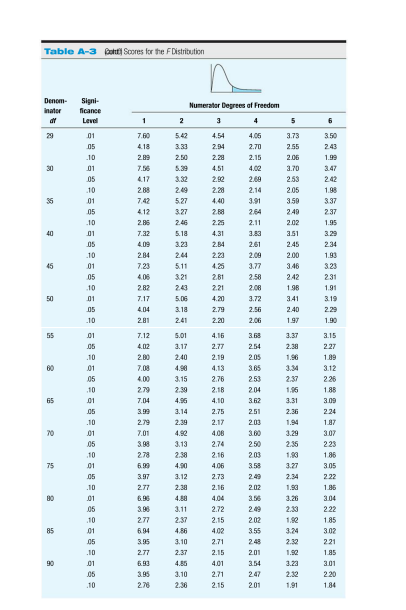
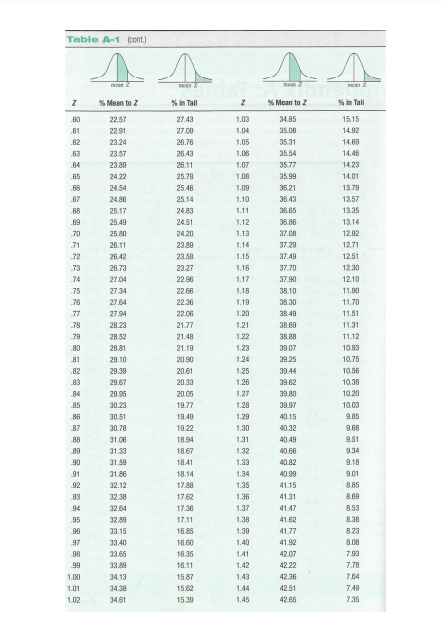
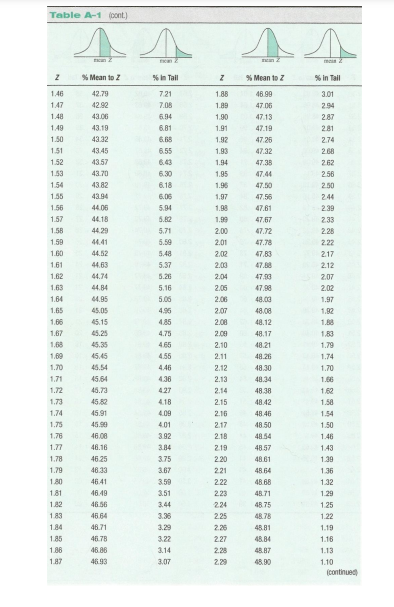
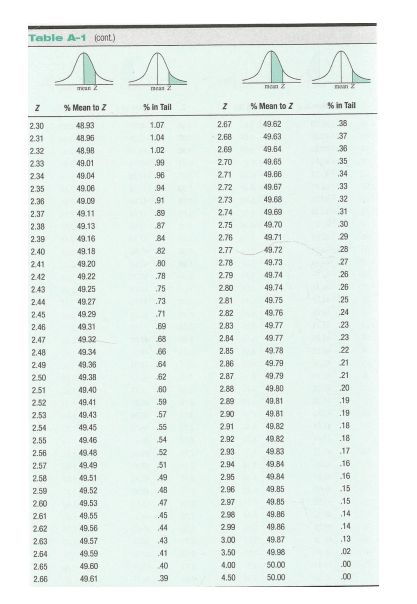
To answer the following questions, it helps to draw a Z distribution (bell curve) and place an individual's Z score on the distribution as a visual aid. Use the Z table for converting between Z score and area (percentage) of the distribution marked by this Z score |
Use this Normal Distribution Table to visualize how your z scores are plotted on a normal distribution: https://www.mathsisfun.com/data/standard-normal-distribution-table.html |
.
Q3 A. Raven has a score of 47. What is Raven's Z score? Round the result to the hundredth (2nd place to the right of the decimal).
[1 point]
Z = (X-m)/s *Population Mean =m *Population Standard Deviation =s
*Raw Score = X *Z score =Z
Z= (X-M)/SD
Z= (47 - 54)/4
Z= -7/4
Z= -1.75
Q3 B. What is the percentage of students that score lower than Raven?
[1 point]
Q3 C. Based on the Z table, if 1000 students take the test, how many of them would likely scoreabove Raven's score? (Round the answer to a whole number)
[1 point]
Q3 D. Erica has a score of 59. What is Erica's Z score?
[1 point]
Z = (X-m)/s *Population Mean =m *Population Standard Deviation =s
*Raw Score = X *Z score =Z
Z= (X-M)/SD
Z= (59 -54)/4
Z= 5/4
Z= 1.25
Q3 E. What is the percentage of students that score lower than Erica?
[1 point]
Using the standard deviation table 0 to 1.25 is 39.44%. Each side of the table is 50%. So 50% add 39.44 and you can see everyone bellow that point.
39.44+ 50=
89.44% of people score lower than Erica
P== 0.894
Q3 F. Based on the Z table, if 1000 students take the test, how many of them would likely scorebelowErica's score? (Round the answer to a whole number)
[1 point]
Q3 G. What is the percentage of students that score between Raven and Erica?
[1 point]
Q3 H. Ryan scores at the 78th percentile on this exam, what is his Z score?
Hint: A score at 78th percentile means 78% of the scores are below this score.
[1 point]
Q3 I. Based on the result of the previous question and using the population mean and SD for Q3, what is Ryan's raw score of working memory?
[1 point]
Q3 J. What would be the median raw score on this exam?
Hint: Review the definition of "median" and then figure out the percentage of scores below (or above) this score.
[1 point]
Q4. Z Distribution: From Probabilities to Proportions (percentages)[7 points]
Answer the following questions with this scenario:
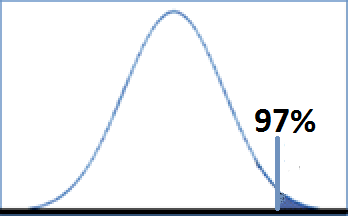
A school district runs a gifted language program in fourth grade with a selection criterion of 97th percentile. That is, students who score at 97th percentile or above on a standardized language test are eligible for the gifted program. Answer the following question based on this scenario.
Helpful tips:
Review the tutorial videos on "how to use the Z table" before answering the following questions.
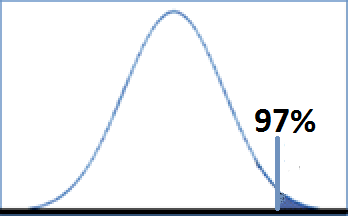
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


