Please help me with this question!
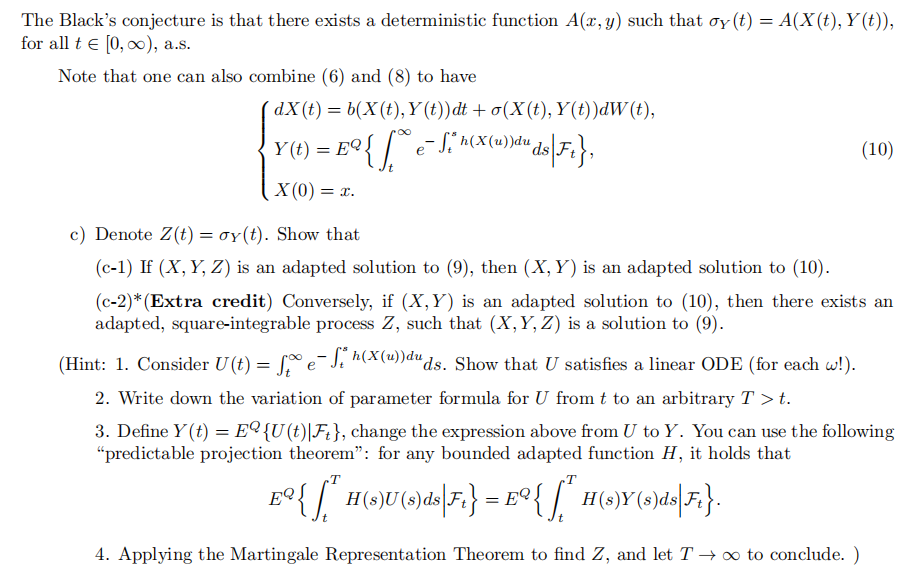
7) This problem concerns the so-called "consol bond" (see Exercise 23.5, 2nd Ed.), which by definition is a perpetual coupon bond that pays, say, $1 every year, forever. 3 a) Denote the price of the T-bond by p(t, T), and assume that the coupon of the consol bound is paid "continuously" (think: continuously compound interest!). Show that, the price of the consol at time t should be Y (t ) = / p(t, s )ds - EX. { J e Sir(w)duds}. (6) where r is the short rate. Check that, if r(t) = r is a constant, then Y (t) = }, for any t 2 0! b) Let Q be the martingale measure, under which p(t, T) follows the dynamics dp (t, T) = p(t, T) r(t ) at + u(t, T)aw(t) }, where W is a Q-Brownian motion. Show that the price Y satisfies the following SDE: dY (t) = (Y(t)r(t) - 1 ) dt + or(t) dW(t), (7) where ov(t) = J. p(t, s)v(t, s)ds. (Hint: You might want to follow the (heuristic) argument in proving the HJM drift condition.) Now assume that the short rate takes the form r(t) = h(X(t)), where h(x) > > 0 is a deterministic function and X is a "factor" process which is assumed to be a diffusion: dX (t) = b(t, X(t)) dt + o(t, X(t))dW(t), X(0) = I. (8) The Black's Conjecture is about a case where the short rate (or X) and the consol rate (or price Y) are correlated in the sense that b(t, X(t)) = b(X(t), Y(t)), o(t, X(t)) = o(X(t), Y(t)). In this case the SDEs (7) and (8) become a Forward-backward SDE over an infinite horizon: dX (t ) = b( X (t ) , Y (t) ) at + o (X(t), Y(t) )dw(t), dY (t) = (Y(t)h(X(t)) - 1)dt + oy (t)dW(t), X(0) = I. (9) Y (t) is bounded for all t e [0, co).The Black's conjecture is that there exists a deterministic function A(x, y) such that oy (t) = A(X(t), Y(t)), for all t E [0, oo), a.s. Note that one can also combine (6) and (8) to have dX (t) = b(X (t), Y (t) ) dt + o(X(t), Y(t) )dW(t), Y(t) = EQ e - J. " (X (u) )duds Ft ; (10) (X(0) = 1. c) Denote Z(t) = oy(t). Show that (c-1) If (X, Y, Z) is an adapted solution to (9), then (X, Y) is an adapted solution to (10). (c-2)*(Extra credit) Conversely, if (X, Y) is an adapted solution to (10), then there exists an adapted, square-integrable process Z, such that (X, Y, Z) is a solution to (9). (Hint: 1. Consider U(t) = [pe J. "(x())duds. Show that U satisfies a linear ODE (for each w!). 2. Write down the variation of parameter formula for U from t to an arbitrary T > t. 3. Define Y(t) = E {U(t)|Ft}, change the expression above from U to Y. You can use the following "predictable projection theorem": for any bounded adapted function H, it holds that H(s)U(s) ds F. = E.{ H (s ) Y (s)ds Fi . 4. Applying the Martingale Representation Theorem to find Z, and let T - co to conclude. )