 Please help!
Please help!
Provided answers in excel spreadsheet

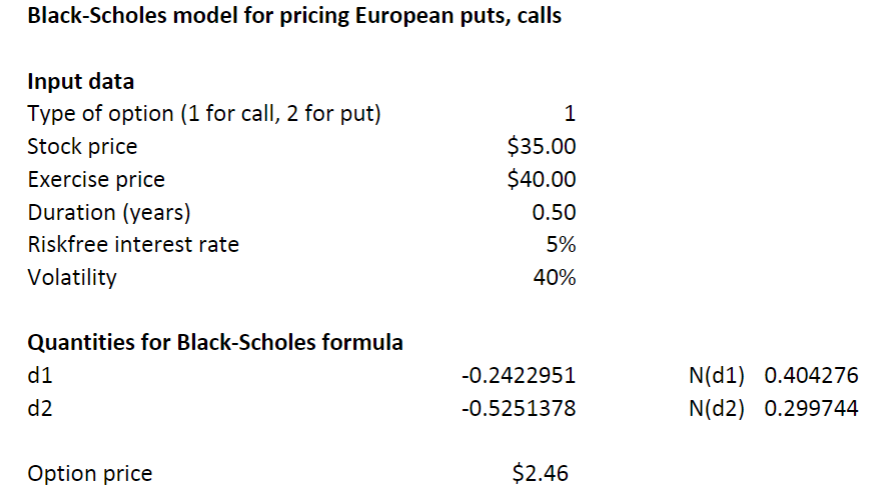
Black-Scholes model for pricing European puts, calls Input data Type of option (1 for call, 2 for put) Stock price Exercise price Duration (years) Riskfree interest rate Volatility 1 $35.00 $40.00 0.50 5% 40% Quantities for Black-Scholes formula d1 d2 -0.2422951 -0.5251378 N(d1) 0.404276 N(d2) 0.299744 Option price $2.46 A European call option on a stock earns the owner an amount equal to the price at expiration minus the exercise price, if the price of the stock on which the call is written exceeds the exercise price. Otherwise, the call pays nothing. A European put option earns the owner an amount equal to the exercise price minus the price at expiration, if the price at expiration is less than the exercise price. Otherwise, the put pays nothing. The file PO2_24.xlsx contains a template that finds (based on the well-known Black-Scholes formula) the price of a European call and put based on the following inputs: today's stock price, the duration of the option (in years), the option's exercise price, the annual risk-free rate of interest, and the annual volatility in stock price. For example, a 40% volatility means approximately that the standard deviation of annual percentage changes in the stock price is 40%. a. Consider a six-month European call option with exercise price $40. Assume a current stock price of $35, a risk-free rate of 5%, and an annual volatility of 40%. Determine the price of the call option. b. Use a data table to show how a change in volatility changes the value of the option. Give an intuitive explanation for your results. c. Use a data table to show how a change in today's stock price changes the option's value. Give an intuitive explanation for your results. d. Use a data table to show how a change in the option's duration changes the option's value. Give an intuitive explanation for your results. Black-Scholes model for pricing European puts, calls Input data Type of option (1 for call, 2 for put) Stock price Exercise price Duration (years) Riskfree interest rate Volatility 1 $35.00 $40.00 0.50 5% 40% Quantities for Black-Scholes formula d1 d2 -0.2422951 -0.5251378 N(d1) 0.404276 N(d2) 0.299744 Option price $2.46 A European call option on a stock earns the owner an amount equal to the price at expiration minus the exercise price, if the price of the stock on which the call is written exceeds the exercise price. Otherwise, the call pays nothing. A European put option earns the owner an amount equal to the exercise price minus the price at expiration, if the price at expiration is less than the exercise price. Otherwise, the put pays nothing. The file PO2_24.xlsx contains a template that finds (based on the well-known Black-Scholes formula) the price of a European call and put based on the following inputs: today's stock price, the duration of the option (in years), the option's exercise price, the annual risk-free rate of interest, and the annual volatility in stock price. For example, a 40% volatility means approximately that the standard deviation of annual percentage changes in the stock price is 40%. a. Consider a six-month European call option with exercise price $40. Assume a current stock price of $35, a risk-free rate of 5%, and an annual volatility of 40%. Determine the price of the call option. b. Use a data table to show how a change in volatility changes the value of the option. Give an intuitive explanation for your results. c. Use a data table to show how a change in today's stock price changes the option's value. Give an intuitive explanation for your results. d. Use a data table to show how a change in the option's duration changes the option's value. Give an intuitive explanation for your results
 Please help!
Please help!






