Please provide detailed update on below questions.

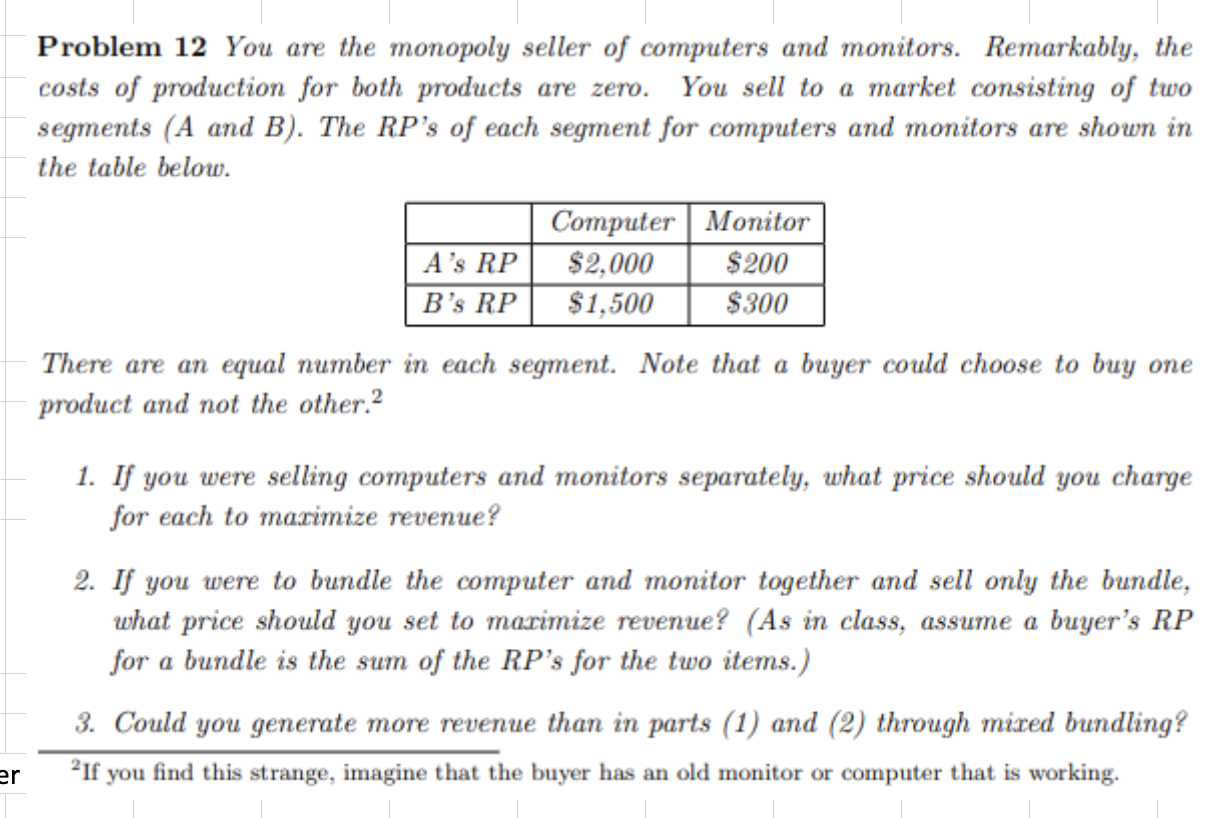
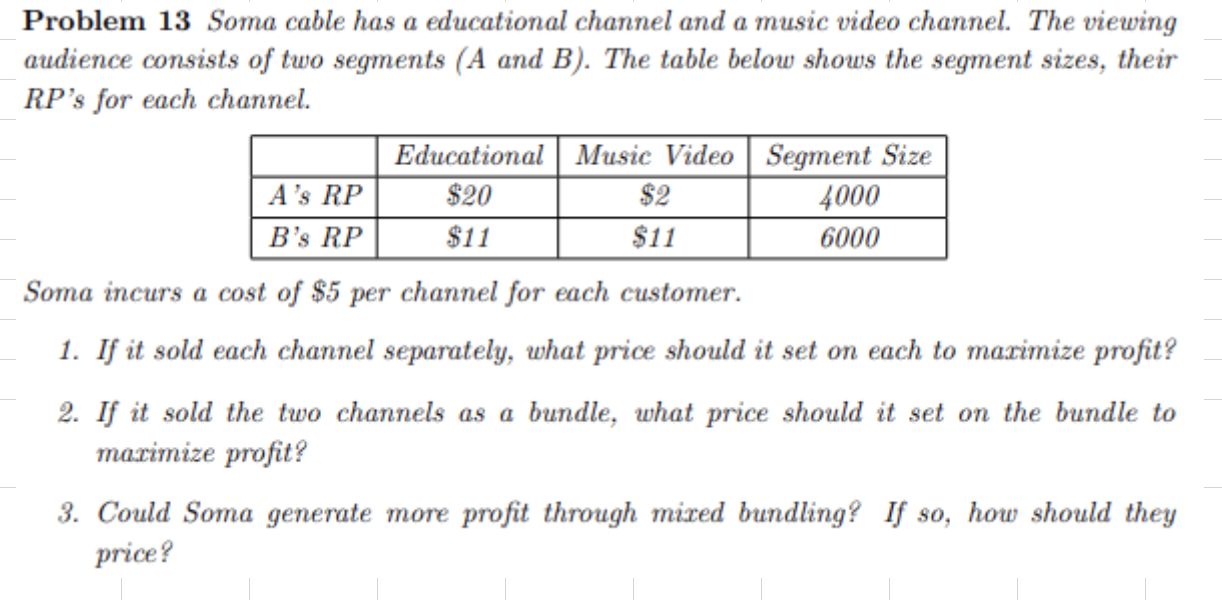
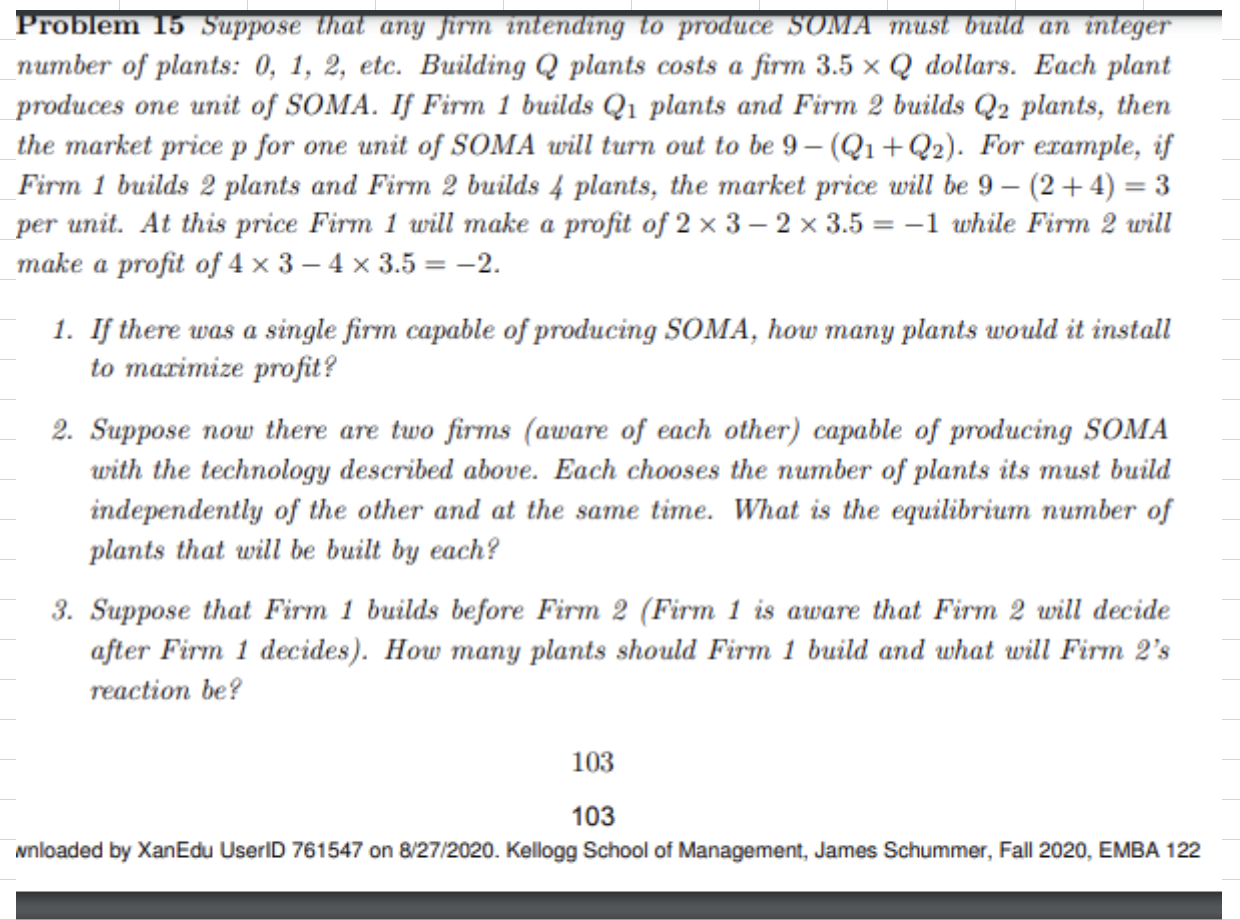
I Problem 16 There are two rms A and B located in different regions that produce an ' identical good at zero cost. Each region is inhabited by a single buyer interested in purchasing _ at most one unit of the good. The RP of each buyer for one unit of the good is $5. If a buyer purchases the good from the seller in the distant location she incurs a transportation _ cost of $2.60. Buyer's will purchase from the location that results in the largest surplus. In case of ties, buyers break them in favor of the closest seller. 1. If the sellers are restricted to announcing a single price per unit for their goods, is pricing at cost (i.e. 0) for both rms an equilibrium? Explain. 2. If the sellers are restricted to announcing a single price (simultaneously) per unit for their goods, what will the equilibrium price be? Explain. . What is the effect on protability, and why, if each rm can also issue a coupon (production and distribution of coupon is costless) that will be directed to buyers in distant regions? So, A will issue a coupon to the buyer who resides in the same region as B. Similarly, B will issue a coupon to the buyer who resides in the same region as A. . What would the equilibrium price and value of a coupon be under the above arrange- ment? Problem 17 In class, bundling has been pitched as a form of price discrimination. Since price discrimination is vulnerable to competition, it would seem that there is no reason to see bundling in competitive markets, unless dictated by transaction costs or economics of manufacturing. Nevertheless, ATFJ' T at one point switched to offering all of its telephone services as a bundle. Further, the market that ATE? T was serving was commonly thought of as being competitive. Is there method to this madness? Consider the following model. Two competing rms make identical products {called widgets) with unlimited capacity. In addition, each rm. can choose to sell a repair contract that goes along with each widget sold. Both rms have the same manufacturing costs: widgets cost $0. 50 per unit to produce, and each repair contract sold costs its seller $0.10. The market consists of 100 buyers each of whom has a reserve price of $3 for one widget. However not all buyers value the repair contract in the same way. The reservation price (RP) of half the buyers for the service contract is $0.05 cents and for the other half it is $0.85 cents. Given prices posted (simultaneously) by the rms, buyers will make the purchase that maximizes their consumer surplus. (If buyers in any one market segment are indi'erent between the two rms, half go to one rm, halfgo to the other.) Each buyer is interested in at most one widget and at most one repair contract. 1. Consider a one-shot game where each rm. sells both widgets and repair contracts separately and buyers can choose to buy none, one or both products. What prices would each rm announce for widgets and repair contracts in equilibrium? 2. Consider a one-shot game where each rm sells widgets and repair contracts as a bundle. Buyers can choose to buy the entire bundle or not. What prices would each rm announce for its bundle in equilibrium? 3. Now suppose Firm 1 prices and sells widgets and repair contracts separately while Firm 2 bundles widgets and repair contracts. In this situation Firm 1 must announce two prices {one for the widgets and the other for the repair contracts) while Firm 2 announces a single price (for its bundle of widgets and contracts). Would prots of BOTH rms in equilibrium be positive? HINT: You do not need to determine the equilibrium prices to answer this question. Consider only whether or not the rms could be stuck making zero prot in equilibrium. 4. 0f the three scenarios above, which is the most protable for the rms? Give an intuitive explanation of why. | | | \\ | | | | | _ Problem 12 You are the monopoly seller of computers and monitons. Remarkably, the '!_ costs of production for both products are zero. You sell to a market consisting of two '!_ segments (A and B). The RP's of each segment for computers and monitors are shown in 'l the table below. _ There are on equal number in each segment. Note that a buyer could choose to buy one " product and not the other.2 V 1. If you were selling computers and monitors separately, what price should you charge V for each to maximize revenue? 2. If you were to bundle the computer and monitor together and sell only the bundle, what price should you set to maximize revenue? (As in class, assume a buyer '3 RP for a bundle is the sum of the RP's for the two items.) _ 3. Could you genemte more revenue than in parts (I) and (2) through mixed bundling? 3r 2If you nd this strange, imagine that the buyer has an old monitor or computer that is working. | | | \\ | | | | | _ Problem 13 Soma cable has a educational channel and a music video channel. The viewing audience consists of two segments (A and B). The table below shows the segment sizes, their :RP's for each channel. ~ - Educational Segment Size m $11 $11 6000 Soma incurs a cost of $5 per channel for each customer. _ 1. If it said each channel separately, what price should it set on each to maximize prot? 2. If it sold the two channels as a bundle, what price should it set on the handle to maximize prot? 3. Could Some generate mom prot through mixed bundling? If so, how should they price? | | | | | | \\ \\ | u s a any rm in ng o u mus a number of plants: 0, 1, 2, etc. Building Q plants costs a rm 3.5 x Q dollars. Each plant produces one unit of SOMA. If Firm 1 builds Q1 plants and F lrm 2 builds Q2 plants, then the market price p for one unit of SOMA will turn out to be 9 (Q1 +Q2). For example, if Firm 1 builds 2 plants and Firm 2 builds 4 plants, the market price will be 9 (2 + 4) = 3 per unit. At this price Firm 1 will make a prot of2 x 3 2 x 3.5 = 1 while Firm 2 will makeapmt of4x34x3.5= 2. I. If there was a single rm capable ofproductng SOMA, hour many plants would it install to maximize pmt? 2. Suppose now there one two rms (aware of each other) capable of producing SOMA with the technology described above. Each chooses the number of plants its must build independently of the other and at the some time. What is the equilibrium number of plants that will he built by each? 3. Suppose that Firm 1 builds before Firm 2 (Firm 1 is awane that Finn 2 will decide after Finn 1 decides). How many plants should Firm 1 build and what will Firm 2's reaction be? 103 103 unloaded by XanEm User") 761547 on mm. Kelogg School of Manageaml. James Schumann Fall 2020, EMBA122