please provide step by step excel

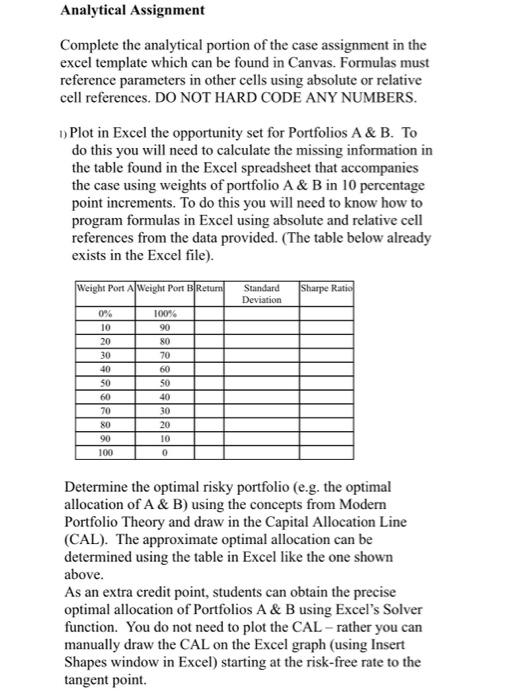
You have been assigned to construct an optimal portfolio comprising two risky assets (Portfolios A \& B) while considering your client's risk tolerance. The attached spread sheet shows historical monthly returns of the two portfolios (A\&B); S\&P 500 index; and 90-day Treasury Bills. Also shown are the annualized returns for each for the period specified. Portfolio A: Active Stock Selection Strategy Portfolio A is an actively managed US equity strategy that uses publicly available fundamental, technical and sentiment factors to assess which stocks are over-priced and which are under-priced. Fundamental factors indicate the magnitude and quality of a company's earnings and the strength of its balance sheet. Examples of such factors include cash flow growth, cash flow return on invested capital, debt to equity ratio, price to cash flow, and accruals which assess earnings quality (low quality earnings indicate that management may be manipulating earnings by adjusting accruals). Companies with favorable fundamental factors tend to outperform those with less favorable factors. Portfolio A uses technical and sentiment factors to identify mispriced stocks by exploiting investor behavioral biases. Examples include: momentum and price reversals where investors tend to over-react to good news by bidding up prices ABOVE fair value and over-react to bad news by bidding down prices BELOW fair value; short interest on a stock which indicates investor sentiment about a company's future prospects; share buybacks and dividend changes which can indicate a positive signal from management's optimism regarding a firm's future prospects; and earnings surprise. Firms with favorable technical and sentiment factors also tend to outperform those stocks having less favorable factors. For example, firms whose earnings and revenue exceed analysts' expectations tend to continue to outperform vs. those firms that experience earnings surprise due to cost cutting. Starting with the market portfolio, the US equity strategy over-weights those stocks having more favorable fundamental, technical and sentiment factors and underweights or avoids those stocks with less favorable or unfavorable factors. The strategy seeks to out-perform the market portfolio as represented by the S\&P 500 . The monthly returns of the US equity strategy are shown in the attached spreadsheet (Portfolio A). Portfolio B: Global Macro Hedge Fund Portfolio B is a global macro hedge fund that seeks to benefit from mis-pricings within and across broad asset classes by taking long and short positions in equity markets, bond markets and currencies. For example, if the manager believes that US equities will out-perform Japanese equities, the portfolio will be long S\&P 500 futures and short TOPIX futures (TOPIX is a Japanese equity index). This long/short trade is not impacted by the overall direction of global equities, but rather the relative return between US and Japanese equities. Similarly for bonds, if the manager believes that interest rates in the United Kingdom (UK) will decline more so than interest rates in Australia, the portfolio will be long UK gilt futures (gilt is the 10-year UK bond) and short Australian 10year bond futures. Again, this trade is not impacted by the overall direction of global interest rates, but rather the relative movement between UK and Australian rates. Recall that bond prices rise as interest rates decline. As a macro hedge fund, Portfolio B is market neutral meaning that the long positions equal short positions thereby dramatically reducing systematic exposures. (e.g. low beta). Combining Portfolios, A \& B Portfolios A \& B are much more volatile than the risk-free rate. You will also find that their correlation is small indicating that there is a diversification benefit to be had from holding both in a portfolio (The correlation is now shown in the spreadsheet. You will need to calculate this using the excel function "=correl(range 1, range2)". You will be meeting with a client that is looking for investment advice from you based on these two portfolios. In preparation for your upcoming meeting with the client, your boss asks that you perform the necessary calculations outlined below and be able to respond to the questions that follow. Hint: You will need to determine the correlations and volatilities for A \& B. Analytical Assignment Complete the analytical portion of the case assignment in the excel template which can be found in Canvas. Formulas must reference parameters in other cells using absolute or relative cell references. DO NOT HARD CODE ANY NUMBERS. 1) Plot in Excel the opportunity set for Portfolios A \& B. To do this you will need to calculate the missing information in the table found in the Excel spreadsheet that accompanies the case using weights of portfolio A \& B in 10 percentage point increments. To do this you will need to know how to program formulas in Excel using absolute and relative cell references from the data provided. (The table below already exists in the Excel file). Determine the optimal risky portfolio (e.g. the optimal allocation of A \& B) using the concepts from Modern Portfolio Theory and draw in the Capital Allocation Line (CAL). The approximate optimal allocation can be determined using the table in Excel like the one shown above. As an extra credit point, students can obtain the precise optimal allocation of Portfolios A \& B using Excel's Solver function. You do not need to plot the CAL - rather you can manually draw the CAL on the Excel graph (using Insert Shapes window in Excel) starting at the risk-free rate to the tangent point. 2) Find the optimal complete portfolio graphically using the client's indifference curve. Plot an indifference curve on the same graph you just created using the utility function formula from Chapter 6 . To make things easier, you can use the same portfolio risk numbers from the table above and then calculate the expected return based on the values of utility (U) and risk aversion coefficient (A) in provided in the Excel spreadsheet. Plot the indifference curve AND the opportunity set of risky assets on the same graph. Next determine the optimal complete portfolio. You did this graphically above, but you need to calculate the precise composition of the optimal risky portfolio (recall ymaxU) and T-Bills (1-ymaxU). 3) Plot the CAPM regressions of Portfolio A and Portfolio B (separate graphs) in the Excel spreadsheet. The market portfolio is represented by the S\&P 500 and the risk-free rate is represented by 90-day T-Bill. Calculate the beta for each portfolio using the following methods: i. The slope function in Excel ii. The beta formula (co-variance divided by the market variance) is explained in the Modules 6&7 Notes; ppt lecture notes; and textbook. Recall the covariance between two assets (A&B) is the volatility of asset A times the volatility of asset B times the correlation between A \& B. Then calculate the alpha for each portfolio A \& B using the intercept function in Excel and the CAPM formula solving for alpha. Note the two CAPM regressions are based on monthly returns so the y-intercept (or alpha) is a MONTHLY alpha. Calculate the annualized alpha using the CAPM formula, beta from above and the annualized returns. After completing, upload the spreadsheet in Canvas Assignments under the Case Study_Analytical Solution. One submission per group. You have been assigned to construct an optimal portfolio comprising two risky assets (Portfolios A \& B) while considering your client's risk tolerance. The attached spread sheet shows historical monthly returns of the two portfolios (A\&B); S\&P 500 index; and 90-day Treasury Bills. Also shown are the annualized returns for each for the period specified. Portfolio A: Active Stock Selection Strategy Portfolio A is an actively managed US equity strategy that uses publicly available fundamental, technical and sentiment factors to assess which stocks are over-priced and which are under-priced. Fundamental factors indicate the magnitude and quality of a company's earnings and the strength of its balance sheet. Examples of such factors include cash flow growth, cash flow return on invested capital, debt to equity ratio, price to cash flow, and accruals which assess earnings quality (low quality earnings indicate that management may be manipulating earnings by adjusting accruals). Companies with favorable fundamental factors tend to outperform those with less favorable factors. Portfolio A uses technical and sentiment factors to identify mispriced stocks by exploiting investor behavioral biases. Examples include: momentum and price reversals where investors tend to over-react to good news by bidding up prices ABOVE fair value and over-react to bad news by bidding down prices BELOW fair value; short interest on a stock which indicates investor sentiment about a company's future prospects; share buybacks and dividend changes which can indicate a positive signal from management's optimism regarding a firm's future prospects; and earnings surprise. Firms with favorable technical and sentiment factors also tend to outperform those stocks having less favorable factors. For example, firms whose earnings and revenue exceed analysts' expectations tend to continue to outperform vs. those firms that experience earnings surprise due to cost cutting. Starting with the market portfolio, the US equity strategy over-weights those stocks having more favorable fundamental, technical and sentiment factors and underweights or avoids those stocks with less favorable or unfavorable factors. The strategy seeks to out-perform the market portfolio as represented by the S\&P 500 . The monthly returns of the US equity strategy are shown in the attached spreadsheet (Portfolio A). Portfolio B: Global Macro Hedge Fund Portfolio B is a global macro hedge fund that seeks to benefit from mis-pricings within and across broad asset classes by taking long and short positions in equity markets, bond markets and currencies. For example, if the manager believes that US equities will out-perform Japanese equities, the portfolio will be long S\&P 500 futures and short TOPIX futures (TOPIX is a Japanese equity index). This long/short trade is not impacted by the overall direction of global equities, but rather the relative return between US and Japanese equities. Similarly for bonds, if the manager believes that interest rates in the United Kingdom (UK) will decline more so than interest rates in Australia, the portfolio will be long UK gilt futures (gilt is the 10-year UK bond) and short Australian 10year bond futures. Again, this trade is not impacted by the overall direction of global interest rates, but rather the relative movement between UK and Australian rates. Recall that bond prices rise as interest rates decline. As a macro hedge fund, Portfolio B is market neutral meaning that the long positions equal short positions thereby dramatically reducing systematic exposures. (e.g. low beta). Combining Portfolios, A \& B Portfolios A \& B are much more volatile than the risk-free rate. You will also find that their correlation is small indicating that there is a diversification benefit to be had from holding both in a portfolio (The correlation is now shown in the spreadsheet. You will need to calculate this using the excel function "=correl(range 1, range2)". You will be meeting with a client that is looking for investment advice from you based on these two portfolios. In preparation for your upcoming meeting with the client, your boss asks that you perform the necessary calculations outlined below and be able to respond to the questions that follow. Hint: You will need to determine the correlations and volatilities for A \& B. Analytical Assignment Complete the analytical portion of the case assignment in the excel template which can be found in Canvas. Formulas must reference parameters in other cells using absolute or relative cell references. DO NOT HARD CODE ANY NUMBERS. 1) Plot in Excel the opportunity set for Portfolios A \& B. To do this you will need to calculate the missing information in the table found in the Excel spreadsheet that accompanies the case using weights of portfolio A \& B in 10 percentage point increments. To do this you will need to know how to program formulas in Excel using absolute and relative cell references from the data provided. (The table below already exists in the Excel file). Determine the optimal risky portfolio (e.g. the optimal allocation of A \& B) using the concepts from Modern Portfolio Theory and draw in the Capital Allocation Line (CAL). The approximate optimal allocation can be determined using the table in Excel like the one shown above. As an extra credit point, students can obtain the precise optimal allocation of Portfolios A \& B using Excel's Solver function. You do not need to plot the CAL - rather you can manually draw the CAL on the Excel graph (using Insert Shapes window in Excel) starting at the risk-free rate to the tangent point. 2) Find the optimal complete portfolio graphically using the client's indifference curve. Plot an indifference curve on the same graph you just created using the utility function formula from Chapter 6 . To make things easier, you can use the same portfolio risk numbers from the table above and then calculate the expected return based on the values of utility (U) and risk aversion coefficient (A) in provided in the Excel spreadsheet. Plot the indifference curve AND the opportunity set of risky assets on the same graph. Next determine the optimal complete portfolio. You did this graphically above, but you need to calculate the precise composition of the optimal risky portfolio (recall ymaxU) and T-Bills (1-ymaxU). 3) Plot the CAPM regressions of Portfolio A and Portfolio B (separate graphs) in the Excel spreadsheet. The market portfolio is represented by the S\&P 500 and the risk-free rate is represented by 90-day T-Bill. Calculate the beta for each portfolio using the following methods: i. The slope function in Excel ii. The beta formula (co-variance divided by the market variance) is explained in the Modules 6&7 Notes; ppt lecture notes; and textbook. Recall the covariance between two assets (A&B) is the volatility of asset A times the volatility of asset B times the correlation between A \& B. Then calculate the alpha for each portfolio A \& B using the intercept function in Excel and the CAPM formula solving for alpha. Note the two CAPM regressions are based on monthly returns so the y-intercept (or alpha) is a MONTHLY alpha. Calculate the annualized alpha using the CAPM formula, beta from above and the annualized returns. After completing, upload the spreadsheet in Canvas Assignments under the Case Study_Analytical Solution. One submission per group