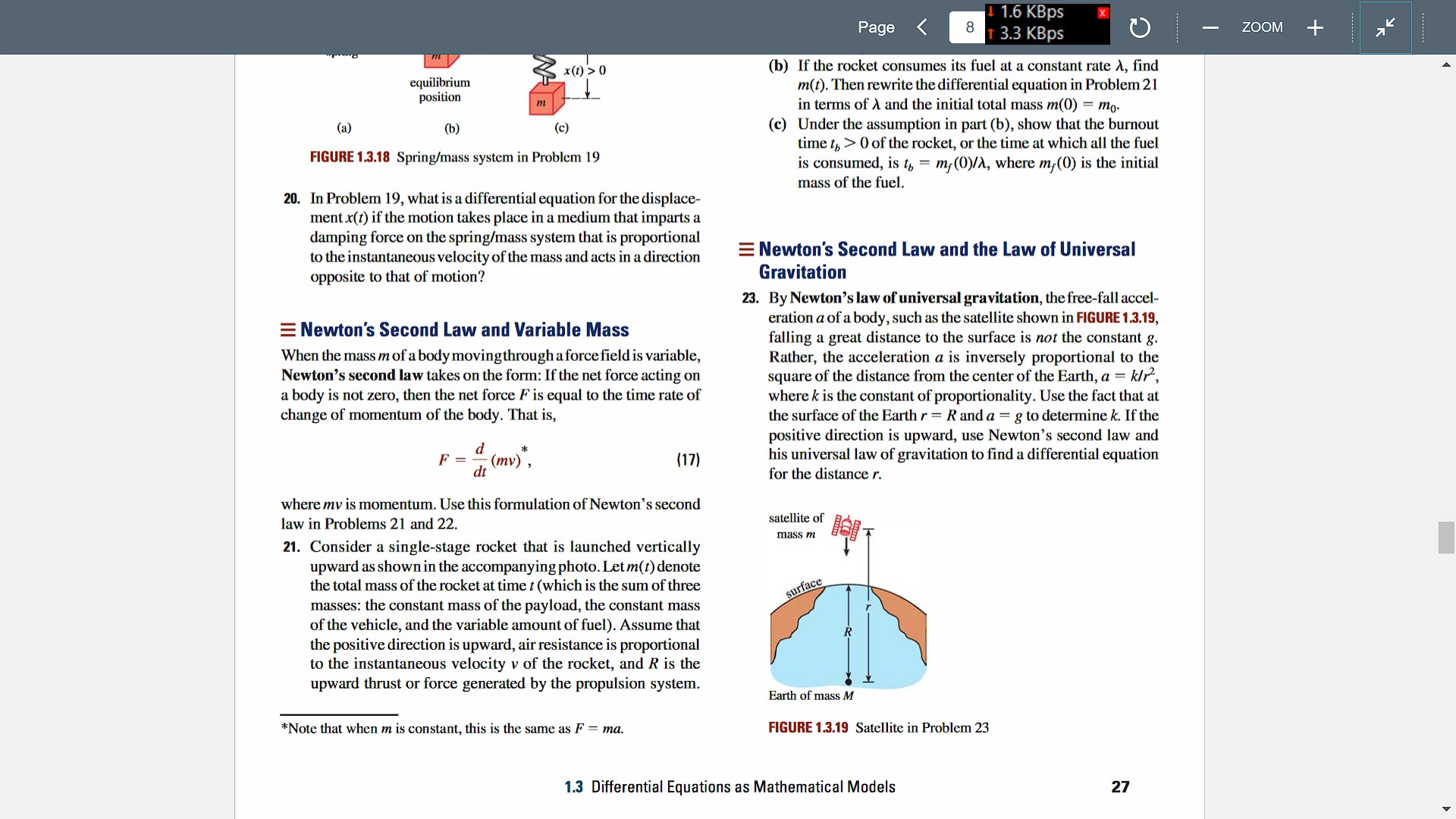
Please refer to the que and Figure in the Image

Zill and Wright, Advanced Engineering Mathematics, 5th edition

1.3 - Problem 23

4 1.6 KBps X Page 8 3.3 KBps ZOOM + x (1) >0 (b) If the rocket consumes its fuel at a constant rate 1, find equilibrium m(t). Then rewrite the differential equation in Problem 21 position m in terms of A and the initial total mass m(0) = mo- (a) (b) (c) (c) Under the assumption in part (b), show that the burnout time to > 0 of the rocket, or the time at which all the fuel FIGURE 1.3.18 Spring/mass system in Problem 19 is consumed, is to = my (0)/), where my(0) is the initial mass of the fuel. 20. In Problem 19, what is a differential equation for the displace- ment x(t) if the motion takes place in a medium that imparts a damping force on the spring/mass system that is proportional to the instantaneous velocity of the mass and acts in a direction Newton's Second Law and the Law of Universal opposite to that of motion? Gravitation 23. By Newton's law of universal gravitation, the free-fall accel- Newton's Second Law and Variable Mass eration a of a body, such as the satellite shown in FIGURE 1.3.19, falling a great distance to the surface is not the constant g. When the mass m of a body moving through a force field is variable, Rather, the acceleration a is inversely proportional to the Newton's second law takes on the form: If the net force acting on square of the distance from the center of the Earth, a = kir, a body is not zero, then the net force F is equal to the time rate of where k is the constant of proportionality. Use the fact that at change of momentum of the body. That is, the surface of the Earth r = R and a = g to determine k. If the positive direction is upward, use Newton's second law and F = d ( mv ) , (17) his universal law of gravitation to find a differential equation dt for the distance r. where my is momentum. Use this formulation of Newton's second law in Problems 21 and 22. satellite of mass m 21. Consider a single-stage rocket that is launched vertically upward as shown in the accompanying photo. Let m(t) denote the total mass of the rocket at time t (which is the sum of three surface masses: the constant mass of the payload, the constant mass of the vehicle, and the variable amount of fuel). Assume that the positive direction is upward, air resistance is proportional to the instantaneous velocity v of the rocket, and R is the upward thrust or force generated by the propulsion system. Earth of mass M *Note that when m is constant, this is the same as F = ma. FIGURE 1.3.19 Satellite in Problem 23 1.3 Differential Equations as Mathematical Models 27