PLEASE SHOW ALL WORK. Thank you.
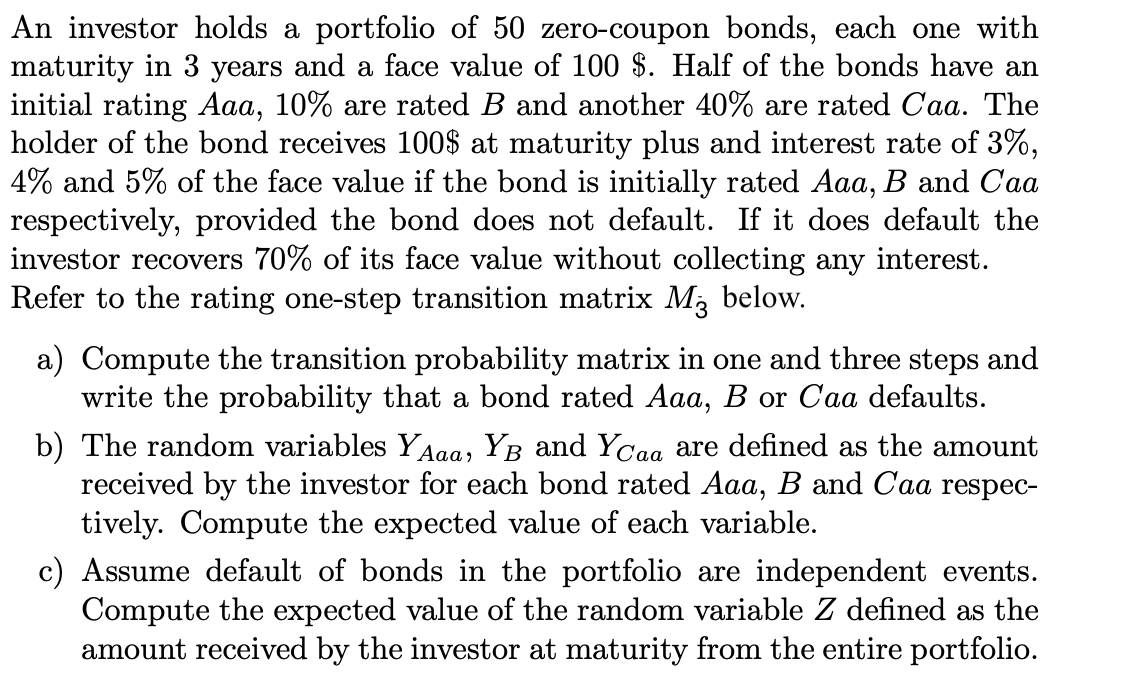

An investor holds a portfolio of 50 zero-coupon bonds, each one with maturity in 3 years and a face value of 100 $. Half of the bonds have an initial rating Aaa, 10% are rated B and another 40% are rated Caa. The holder of the bond receives 100$ at maturity plus and interest rate of 3%, 4% and 5% of the face value if the bond is initially rated Aaa, B and Caa respectively, provided the bond does not default. If it does default the investor recovers 70% of its face value without collecting any interest. Refer to the rating one-step transition matrix Mz below. a) Compute the transition probability matrix in one and three steps and write the probability that a bond rated Aaa, B or Caa defaults. b) The random variables Y Aaa, YB and Ycaa are defined as the amount received by the investor for each bond rated Aaa, B and Caa respec- tively. Compute the expected value of each variable. c) Assume default of bonds in the portfolio are independent events. Compute the expected value of the random variable Z defined as the amount received by the investor at maturity from the entire portfolio. M_3 = [ 0.3724 0.1303 0.0533 0.0723 0.1819 0.1058 0.0570 HOTEL 0.0677 0.2985 0.1298 0.0183 0.0375 0.0921 0.1023 0.0305 0.0487 0.2942 0.1914 0.1788 0.1149 0.1099 0.1260 0.0611 0.0535 0.2813 0.1115 0.1032 0.1741 0.0669 0.1310 0.0497 0.0561 0.2017 0.1045 0.0043 0.1322 0.0156 0.0879 0.1992 0.1439 0.2245 0.1066 0.0159 0.1647 0.1687 0.1454 0.1405 0.1096 0.2472 0.1883 0.1501 0.1631 0.0360 0.0042 0.1454 0.1986 1.0000 ] An investor holds a portfolio of 50 zero-coupon bonds, each one with maturity in 3 years and a face value of 100 $. Half of the bonds have an initial rating Aaa, 10% are rated B and another 40% are rated Caa. The holder of the bond receives 100$ at maturity plus and interest rate of 3%, 4% and 5% of the face value if the bond is initially rated Aaa, B and Caa respectively, provided the bond does not default. If it does default the investor recovers 70% of its face value without collecting any interest. Refer to the rating one-step transition matrix Mz below. a) Compute the transition probability matrix in one and three steps and write the probability that a bond rated Aaa, B or Caa defaults. b) The random variables Y Aaa, YB and Ycaa are defined as the amount received by the investor for each bond rated Aaa, B and Caa respec- tively. Compute the expected value of each variable. c) Assume default of bonds in the portfolio are independent events. Compute the expected value of the random variable Z defined as the amount received by the investor at maturity from the entire portfolio. M_3 = [ 0.3724 0.1303 0.0533 0.0723 0.1819 0.1058 0.0570 HOTEL 0.0677 0.2985 0.1298 0.0183 0.0375 0.0921 0.1023 0.0305 0.0487 0.2942 0.1914 0.1788 0.1149 0.1099 0.1260 0.0611 0.0535 0.2813 0.1115 0.1032 0.1741 0.0669 0.1310 0.0497 0.0561 0.2017 0.1045 0.0043 0.1322 0.0156 0.0879 0.1992 0.1439 0.2245 0.1066 0.0159 0.1647 0.1687 0.1454 0.1405 0.1096 0.2472 0.1883 0.1501 0.1631 0.0360 0.0042 0.1454 0.1986 1.0000 ]








