Please show and explain all hand written calculations. Show equations and steps.
Post the MatLab code
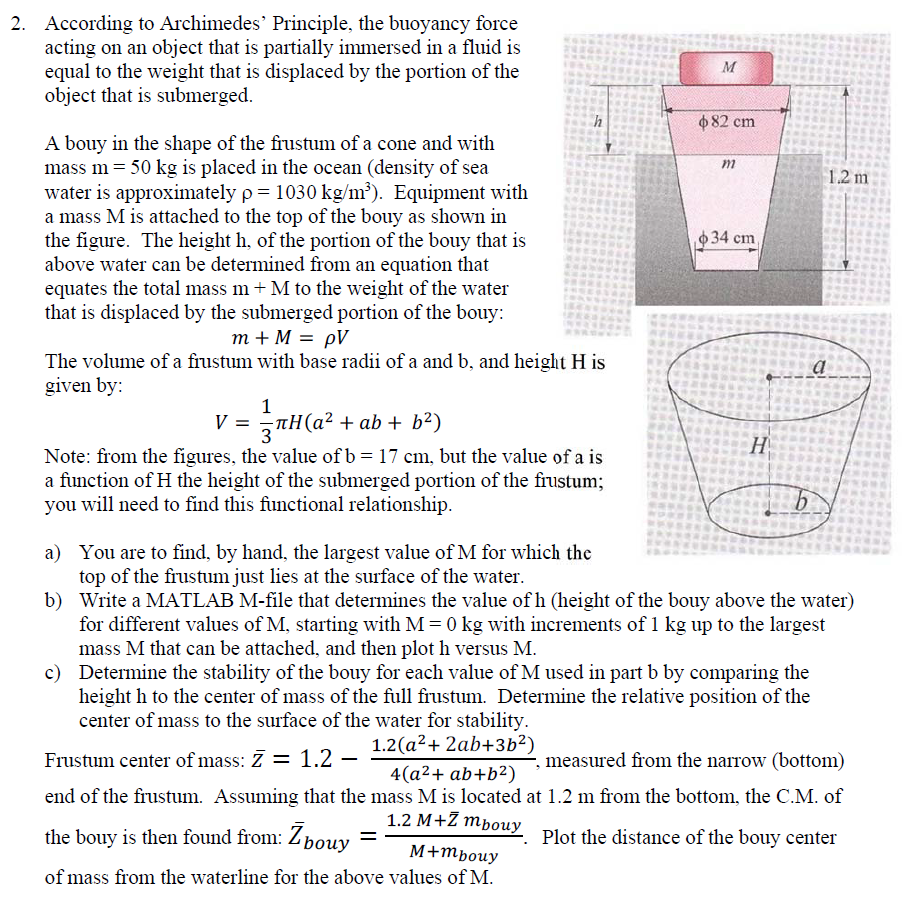
2. According to Archimedes' Principle, the buoyancy force acting on an object that is partially immersed in a fluid is equal to the weight that is displaced by the portion of the object that is submerged A bouy in the shape of the frustum of a cone and with mass m 50 kg is placed in the ocean (density of sea water is approximately 1030 kg/m3). Equipment with a mass M is attached to the top of the bouy as shown in the figure. The height h, of the portion of the bouy that is above water can be determined from an equation that equates the total mass m + M to the weight of the water that is displaced by the submerged portion of the bouy cm The volume of a frustum with base radii of a and b, and height H is ven tb Note: from the figures, the value of b = 17 cm, but the value of a is a function of H the height of the submerged portion of the frustum; you will need to find this functional relationship You are to find, by hand, the largest value of M for which the top of the frustum just lies at the surface of the water Write a MATLAB M-file that determines the value of h (height of the bouy above the water) for different values of M, starting with M- 0 kg with increments of 1 kg up to the largest mass M that can be attached, and then plot h versus M Determine the stability of the bouy for each value of M used in part b by comparing the height h to the center of mass of the full frustum. Determine the relative position of the ccntor ofmass to the suracc of the watox for stability a) b) c) 1.2(a2+ 2ab+3b2) Frustum center of mass: Z - 1.2 end of the frustum. Assuming that the mass M is located at 1.2 m from the bottom, the C.M. of the bouy is then found from: Zbou of mass from the waterlinc for the above values of M. (a2+ ab+b2) measured from the narrow (bottom) Plot the distance of the bouy center 2. According to Archimedes' Principle, the buoyancy force acting on an object that is partially immersed in a fluid is equal to the weight that is displaced by the portion of the object that is submerged A bouy in the shape of the frustum of a cone and with mass m 50 kg is placed in the ocean (density of sea water is approximately 1030 kg/m3). Equipment with a mass M is attached to the top of the bouy as shown in the figure. The height h, of the portion of the bouy that is above water can be determined from an equation that equates the total mass m + M to the weight of the water that is displaced by the submerged portion of the bouy cm The volume of a frustum with base radii of a and b, and height H is ven tb Note: from the figures, the value of b = 17 cm, but the value of a is a function of H the height of the submerged portion of the frustum; you will need to find this functional relationship You are to find, by hand, the largest value of M for which the top of the frustum just lies at the surface of the water Write a MATLAB M-file that determines the value of h (height of the bouy above the water) for different values of M, starting with M- 0 kg with increments of 1 kg up to the largest mass M that can be attached, and then plot h versus M Determine the stability of the bouy for each value of M used in part b by comparing the height h to the center of mass of the full frustum. Determine the relative position of the ccntor ofmass to the suracc of the watox for stability a) b) c) 1.2(a2+ 2ab+3b2) Frustum center of mass: Z - 1.2 end of the frustum. Assuming that the mass M is located at 1.2 m from the bottom, the C.M. of the bouy is then found from: Zbou of mass from the waterlinc for the above values of M. (a2+ ab+b2) measured from the narrow (bottom) Plot the distance of the bouy center







