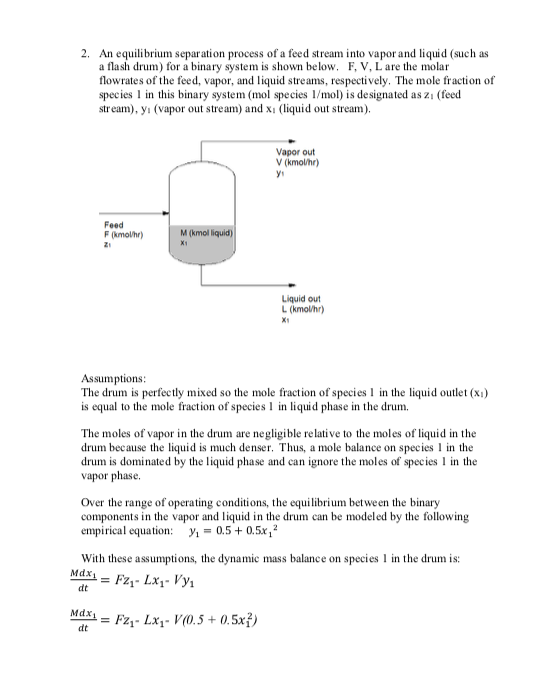
please show steps to answers provided.

2. An equilibrium separation process of a feed stream into vapor and liquid (such as a flash drum) for a binary system is shown below. F, V, L are the molar flowrates of the feed, vapor, and liquid streams, respectively. The mole fraction of species 1 in this binary system (mol species 1/mol) is designated as z1 (feed stream), y. (vapor out stream) and xi (liquid out stream), Vapor out V (kmol/hr) Feed Fikmothr) M (kmol liquid) Liquid out L (kmolhr) Assumptions: The drum is perfectly mixed so the mole fraction of species 1 in the liquid outlet (X1) is equal to the mole fraction of species 1 in liquid phase in the drum. The moles of vapor in the drum are negligible relative to the moles of liquid in the drum because the liquid is much denser. Thus, a mole balance on species 1 in the drum is dominated by the liquid phase and can ignore the moles of species 1 in the vapor phase, Over the range of operating conditions, the equilibrium between the binary components in the vapor and liquid in the drum can be modeled by the following empirical equation: y = 0.5 +0.5x, ? With these assumptions, the dynamic mass balance on species 1 in the drum is: Mdx = F2 - LX - Vy. dt Mdx dt = F2 - LX - V(0.5 +0.5x) a. Write the transfer function in standard form that describes the change in xi(t) if the inlet concentration, Zi(t), changes. Over the range of operating conditions F, L, and V are constant (therefore M is also constant). X' (s) Z' (s) b. The process is running at steady state, with L - 40 kmol/min and V-60 kmol/min. At steady state, z1 -0.4, X1-0.215 and y. -0.523. M-800 kmol. What are the the gain and time constant of the transfer function in part a (with units)? K-1.89 (no units) T-15.1 min 2/21/2022 Note: If you completed the problem with the initial values and just plugged them in your formula for K and tau, this still works for L-40 kmol/min and V-60 kmol/min. At steady state, 21 -0.4, XI-0.537 and y. -0.194. M-800 kmol. What are the the gain and time constant of the transfer function in part a (with units)? K - 1.38 (no units) - 11.1 min I updated the problem with new values because the original values did not satisfy the initial steady state balance. c. From steady state, the inlet concentration of zi is reduced to 0.3. Use the transfer function to estimate the new steady state concentration of species 1 in the liquid phase (x1). x 0.399 (for K-1.38 and x-0.537, the original problem statement) With the corrected value of K-1,89 and Xis. 0.215, new x: 0,026 2. An equilibrium separation process of a feed stream into vapor and liquid (such as a flash drum) for a binary system is shown below. F, V, L are the molar flowrates of the feed, vapor, and liquid streams, respectively. The mole fraction of species 1 in this binary system (mol species 1/mol) is designated as z1 (feed stream), y. (vapor out stream) and xi (liquid out stream), Vapor out V (kmol/hr) Feed Fikmothr) M (kmol liquid) Liquid out L (kmolhr) Assumptions: The drum is perfectly mixed so the mole fraction of species 1 in the liquid outlet (X1) is equal to the mole fraction of species 1 in liquid phase in the drum. The moles of vapor in the drum are negligible relative to the moles of liquid in the drum because the liquid is much denser. Thus, a mole balance on species 1 in the drum is dominated by the liquid phase and can ignore the moles of species 1 in the vapor phase, Over the range of operating conditions, the equilibrium between the binary components in the vapor and liquid in the drum can be modeled by the following empirical equation: y = 0.5 +0.5x, ? With these assumptions, the dynamic mass balance on species 1 in the drum is: Mdx = F2 - LX - Vy. dt Mdx dt = F2 - LX - V(0.5 +0.5x) a. Write the transfer function in standard form that describes the change in xi(t) if the inlet concentration, Zi(t), changes. Over the range of operating conditions F, L, and V are constant (therefore M is also constant). X' (s) Z' (s) b. The process is running at steady state, with L - 40 kmol/min and V-60 kmol/min. At steady state, z1 -0.4, X1-0.215 and y. -0.523. M-800 kmol. What are the the gain and time constant of the transfer function in part a (with units)? K-1.89 (no units) T-15.1 min 2/21/2022 Note: If you completed the problem with the initial values and just plugged them in your formula for K and tau, this still works for L-40 kmol/min and V-60 kmol/min. At steady state, 21 -0.4, XI-0.537 and y. -0.194. M-800 kmol. What are the the gain and time constant of the transfer function in part a (with units)? K - 1.38 (no units) - 11.1 min I updated the problem with new values because the original values did not satisfy the initial steady state balance. c. From steady state, the inlet concentration of zi is reduced to 0.3. Use the transfer function to estimate the new steady state concentration of species 1 in the liquid phase (x1). x 0.399 (for K-1.38 and x-0.537, the original problem statement) With the corrected value of K-1,89 and Xis. 0.215, new x: 0,026