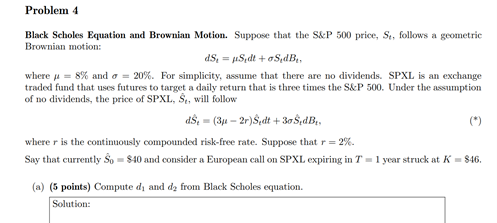
Please solve all subparts

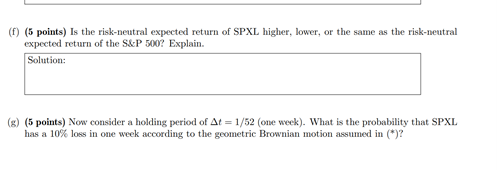
Problem 4 Black Scholes Equation and Brownian Motion. Suppose that the S&P 500 price, S., follows a geometric Brownian motion: dS, =Sdt + 5dB where y = 8% and o = 20%. For simplicity, assume that there are no dividends. SPXL is an exchange traded fund that uses futures to target a daily return that is three times the S&P 500. Under the assumption of no dividends, the price of SPXL, S, will follow ds - (3 - 2r)$dt +30$,dB, (8) where r is the continuously compounded risk-free rate. Suppose that r = 2%. Say that currently So = $40 and consider a European call on SPXL expiring in T = 1 year struck at K = $46. (a) (5 points) Computed, and dy from Black Scholes equation. Solution: (b) (5 points) Compute the Black Scholes price of the call option. Solution: (c) (5 points) What is the risk-neutral probability the call ends up in the money? You may use any approximation we have talked about in class. (d) (5 points) Compute the price of a derivative security that pays $1 if the call is in the money at T = 1. Will the expected return of such a derivative be higher or lower than the risk-free rate? Solution: () (5 points) Is e-TE($r-K)*] greater or less than the price of the call? Explain briefly. Solution: (f) (5 points) Is the risk-neutral expected return of SPXL higher, lower, or the same as the risk-neutral expected return of the S&P 500? Explain. Solution: (s) (5 points) Now consider a holding period of At = 1/52 (one week). What is the probability that SPXL has a 10% loss in one week according to the geometric Brownian motion assumed in (*)? a (h) (5 points) If the expected return of SPXL was instead just 3 instead of 34 - 2r, would there be an issue? Explain. Solution: Problem 4 Black Scholes Equation and Brownian Motion. Suppose that the S&P 500 price, S., follows a geometric Brownian motion: dS, =Sdt + 5dB where y = 8% and o = 20%. For simplicity, assume that there are no dividends. SPXL is an exchange traded fund that uses futures to target a daily return that is three times the S&P 500. Under the assumption of no dividends, the price of SPXL, S, will follow ds - (3 - 2r)$dt +30$,dB, (8) where r is the continuously compounded risk-free rate. Suppose that r = 2%. Say that currently So = $40 and consider a European call on SPXL expiring in T = 1 year struck at K = $46. (a) (5 points) Computed, and dy from Black Scholes equation. Solution: (b) (5 points) Compute the Black Scholes price of the call option. Solution: (c) (5 points) What is the risk-neutral probability the call ends up in the money? You may use any approximation we have talked about in class. (d) (5 points) Compute the price of a derivative security that pays $1 if the call is in the money at T = 1. Will the expected return of such a derivative be higher or lower than the risk-free rate? Solution: () (5 points) Is e-TE($r-K)*] greater or less than the price of the call? Explain briefly. Solution: (f) (5 points) Is the risk-neutral expected return of SPXL higher, lower, or the same as the risk-neutral expected return of the S&P 500? Explain. Solution: (s) (5 points) Now consider a holding period of At = 1/52 (one week). What is the probability that SPXL has a 10% loss in one week according to the geometric Brownian motion assumed in (*)? a (h) (5 points) If the expected return of SPXL was instead just 3 instead of 34 - 2r, would there be an issue? Explain. Solution