Please solve in matlab

The matrix

is called a rotation matrix since it can be used for rotating vectors (or points) in the xy-plane counter-clockwise through an angle about the origin. If (x, y) is a point in the plane, and is a vector containing the coordinates of this point, then the entries of the vector will contain the coordinates of the rotated point. Consider a polygon with vertices (0, 0), (0, 0.5), (-0.3, 0.5), (0.2, 1), (0.7, 0.5), (0.4, 0.5), (0.4, 0). Make a plot of this polygon in Matlab using the fill function. Rotate this polygon by an angle of 53 degrees using matrix-vector multiplication in Matlab with an appropriately chosen rotation matrix R. Make another plot of the rotated polygon using fill. Check whether your answer makes sense!

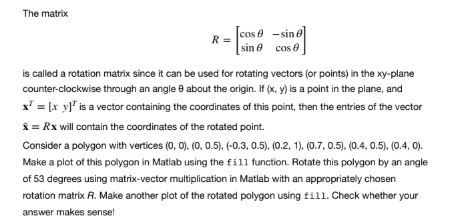
The matrix R ose -sin el sin cos is called a rotation matrix since it can be used for rotating vectors (or points) in the xy.plane counter-clockwise through an angle about the origin. If X. y) is a point in the plane, and X'=xy' is a vector containing the coordinates of this point, then the entries of the vector X=Rx will contain the coordinates of the rotated point. Consider a polygon with vertices (0,0), (0, 0.5).(-0.3, 0.5), (0.2, 1), (0.7.0.5), (0.4, 0.5), (0.4, 0). Make a plot of this polygon in Matlab using the fill function. Rotate this polygon by an angle of 53 degrees using matrix-vector multiplication in Matlab with an appropriately chosen rotation matrix R. Make another plot of the rotated polygon using fill. Check whether your answer makes sense! The matrix R ose -sin el sin cos is called a rotation matrix since it can be used for rotating vectors (or points) in the xy.plane counter-clockwise through an angle about the origin. If X. y) is a point in the plane, and X'=xy' is a vector containing the coordinates of this point, then the entries of the vector X=Rx will contain the coordinates of the rotated point. Consider a polygon with vertices (0,0), (0, 0.5).(-0.3, 0.5), (0.2, 1), (0.7.0.5), (0.4, 0.5), (0.4, 0). Make a plot of this polygon in Matlab using the fill function. Rotate this polygon by an angle of 53 degrees using matrix-vector multiplication in Matlab with an appropriately chosen rotation matrix R. Make another plot of the rotated polygon using fill. Check whether your answer makes sense