Please solve the following Continuous Time Markov Process Problem associated with Queueing Theory. Please spend some time reading the context and hint thoroughly, this should be an easy problem. Thank you for your patience!

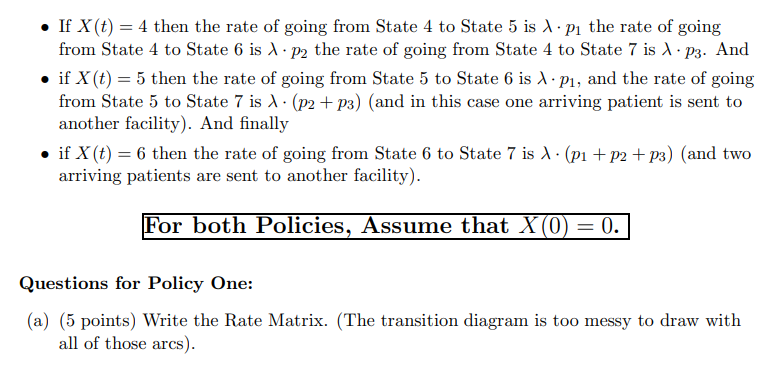
5. (35 points total) Consider a small temporary medical facility constructed to process incoming patients having probable COVID-IQ. The temporary structure is small and has four staffed triage rooms and three rooms to accommodate patients who are waiting for a triage room- Patient-arrival events follows a Poisson process with a rate of one arrival event per two hours. Each arrival event consists of the arrival of either a single patient, or two patients or three patients. Let p, denote the probability that a given arrival event consists of the arrival of a total of i patents. Assume that p1 2 BLT, p2 = (1.2, and p3 = [1.1. Upon arrival a patient is taken to an triage room if an triage room is available. If a triage room is not available then the patient is talcen to an available waiting room. The patients stay in the waiting room until a triage room becomes available, at which time the patient is transferred to an triage room. If no waiting room is available for a particular patient arriving to the facility then that patient is diverted to another facility. If the facility is deemed to be \"closed to accepting new patients\" [See Policy IUne and Policy Two below} then arriving ambulances are directed to take their patients to an alternate hospital. Patients' length of stay in a triage room follows a negative exponential distribution with a mean of 2.5 hours. This small temporary medical facility is considering two different policies of when to close down admittance due to capacity constraints. The facility is interested in studying the statistical performance of the facility with respect to closures caused by capacity limitation, starting from an epoch at which all rooms are empty. You will be asked to model the system two ways; 1} Policy One: the facility remains open at all times as long as there are rooms of some sort available [current policy); and 2} Policy Two: the facility closes to new patients (does not admit any new patients) as soon as least one waiting room is lled. Policy One Details: Here the facility remains open to admissions as long an there is at least one waiting room still available. Assmning that all 4 triage rooms are occupied then: 0 If U waiting rooms are occupied then the facility can accommodate arrival events consisting of l or 2 or 3 patients in the arrival event. 0 If the facility has just I waiting room occupied (and thus 2 waiting rooms available} then the facility will accommodate arrival events consisting of 1 or 2 or patients in the arrival event, and if there are 3 patients in the arrival event then the facility can receive 2 of the 3 patients and send one patient to an alternate facility. or If 2 waiting rooms are occupied [and thus 1 waiting room available) then the facility will accommodate arrival events consisting of 1 patient in the arrival event, and if there are 2 or 3 patients in the arrival event the the facility can receive 1 patient and send the other 1 or 2 patients to an alternate facility. 0 Finally if there U waiting rooms available then an arrival event consisting of the arrival of either 1 or 2 or 3 arriving patients then all of the arriving patients would be sent to an alternate facility, and there would not be a change the state in the model of the temporary medical facility at all. Notice: If the state of the system, X(t}, is the number of patients in the facility at time t (so the states would be U, 1, 2, + ' . , 7), then if XE) 4 then all three waiting rooms are available and, o If X62} 2 4 then the rate of going from State 4 to State 5 is A -p1 the rate of going from State 4 to State 5 is A + 312 the rate of going from State 4 to State 7" is A + 313. And In if X [t] = 5 then the rate of going from State 5 to State 5 is A p1, and the rate of going from State 5 to State 7 is A + [332 + p3} (and in this ease one arriving patient is sent to another facility}. And finallyr o ifX[t} = '6 then the rate of going from State 5 to State 7' is A + [301 +332 +p3} [and two arriving patients are sent to another facility}. I or both Policies Assume that X (9m Questions for Policy One: {a} (5 points} Write the Rate Matrix. [The transition diagram is too mess},F to draw with all of those ares}