Question: Let L{f()} be the Laplace Transform of a function f(t), defined as L{f)}= [ f(t)e* dt = F(s), say, in terms of a complex

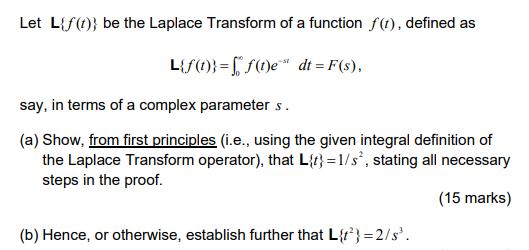
Let L{f()} be the Laplace Transform of a function f(t), defined as

Let L{f()} be the Laplace Transform of a function f(t), defined as L{f)}= [ f(t)e* dt = F(s), say, in terms of a complex parameter s. (a) Show, from first principles (i.e., using the given integral definition of the Laplace Transform operator), that L{f} = 1/s, stating all necessary steps in the proof. (15 marks) (b) Hence, or otherwise, establish further that L{t'} =2/s'.

Step by Step Solution

★★★★★

3.40 Rating (153 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Let Y(s) = L[y(t)] be the Laplace transform of the solution of a second-order differential equation representing a system with input x(t) and some initial conditions, (a) Find the zero-state response...

In two different ways, find the Laplace transform of G(t) = d/dt (te-t cost)

Let L{f(1)} be the Laplace Transform of a function f(t), defined as L{f(!)} = [ f(t)e*" dt = F(s), say, in terms of a complex parameter s. (a) Show, from first principles (i.e., using the given...

Problem 10.9. Consider a casual function, g(t), such that g(t) = 0 for t 0. Let 81(2)= dte "g(1) be the Laplace transform. Let F(w)=dt eg(t) be the Fourier transform. Show that and show that the...

3 ) consider the following Second order liveer ordinary differential equations y" (t ) - y'(6) - 24 (t) - 81-3 for t20 & #$3. where 8 ( t ) is the Dirac delta function and y (0)= 1 , y'(0)=2. ( 10...

Question 3 [10] Solve the Laplace equation "We = I], (U 1:: .1: at 00.0 a: y -=: no), given that u[,y} E I] for every y, Il is bounded as :1' 3- no, and on the positive 3: axis 143113} = g

Given the circuit in Fig. 18.41, with its excitation, determine the Fourier transform of i(t). i(t) 1 mH

1. A player throws a fair die and simultaneously flips a fair coin. If the coin lands heads, then she wins twice, and if tails, then one-half of the value that appears on the die. Determine her...

A region in space contains a total positive charge Q that is distributed spherically such that the volume charge density p (r} is given by Here a is a positive constant having units of C/m3. (a)...

Determine the convolution of the following pairs of signals by means of the z- transform. (a) x1(n) = (1/4)nu(n - 1), x2(n) = [1 + (1/2)n]u(n) (b) x1(n) = u(n), x2 (n) = (n) + (1/2)n u(n) (c) x1(n) =...

Prepare common-size comparative statements of financial position for years 2018 and 2017. (10) Use the following financial statements of Precise Company to answer the requirements. Precise Company...

Explain in detail. Question 1 (12 points): If we apply the BFS algorithm on the following graph, which of these visiting sequences are valid: abhfeg, abeghf, ghebfa, bhfaeg. b

The following information concerns production in the Forging Department for June. All direct materials are placed into the process at the beginning of production, and conversion costs are incurred...

A mural at the zoo is made up of 14 equal sections. An artist wants to paint four different animals using 11 of the 14 sections. Complete each fraction below to show one way that the artist can paint...

Suppose Neil Armstrong decided to throw a golf ball into the air while he was standing on the moon and that the height of the golf ball was modeled by the equation below, where s is measured in feet...

On January 1, 2025, Cullumber issued $740,000 of 9% serial bonds at par. Semiannual interest is payable on January 1 and July 1 and principal of $74,000 matures each January 1 starting in 2026. The...

mmunications Research Problem 3. Emerald Corporation is required to change its method of accounting for Federal income tax purposes. The change will require an adjustment to income to be made over...

Provide an example of an aggressive accounting practice. Why is this practice aggressive?

A passive RLC filter is represented by the ordinary differential equation where x(t) is the input and y(t) is the output. (a) Find the transfer function H(s) of the filter and indicate what type of...

Given that the Z-transform of a discrete-time cosine A cos(Ï 0 n) u[n] is (a) Use the given Z-transform to find a difference equation whose output y[n] is a discrete-time cosine A cos(Ï 0...

Use MATLAB to design a Butterworth second-order low-pass discrete filter H(Z) with half-power frequency hp = /2, and dc gain of 1. Consider this low-pass filter a prototype that can be used to...

D&G Industries operates three shifts every day of the week. Each shift includes full-time hourly workers, nonsupervisory salaried employees, and supervisors/ managers. D&G Industries would like to...

The following sample data were recently collected in the course of conducting a randomized block analysis of variance. Based on these sample data, what conclusions should be reached about blocking...

The Cordage Institute, based in Wayne, Pennsylvania, is an international association of manufacturers, producers, and resellers of cordage, rope, and twine. It is a not-for-profit corporation that...