A passive RLC filter is represented by the ordinary differential equation where x(t) is the input and
Question:
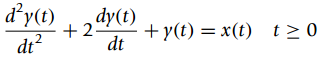
where x(t) is the input and y(t) is the output.
(a) Find the transfer function H(s) of the filter and indicate what type of filter it is.
(b) For an input x(t) = 2u(t), find the corresponding output y(t) and determine the steady-state response.
(c) If the input is x1(t) = 2[u(t) ˆ’ u(t ˆ’ 1)], express the corre-sponding output y1(t) in terms of the response y(t) obtained above.
(d) For the above two cases find the difference between the outputs and the inputs of the filter and indicate if this difference signals tend to zero as t increases. If so, what does this mean?
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





