Please solve using MATLAB

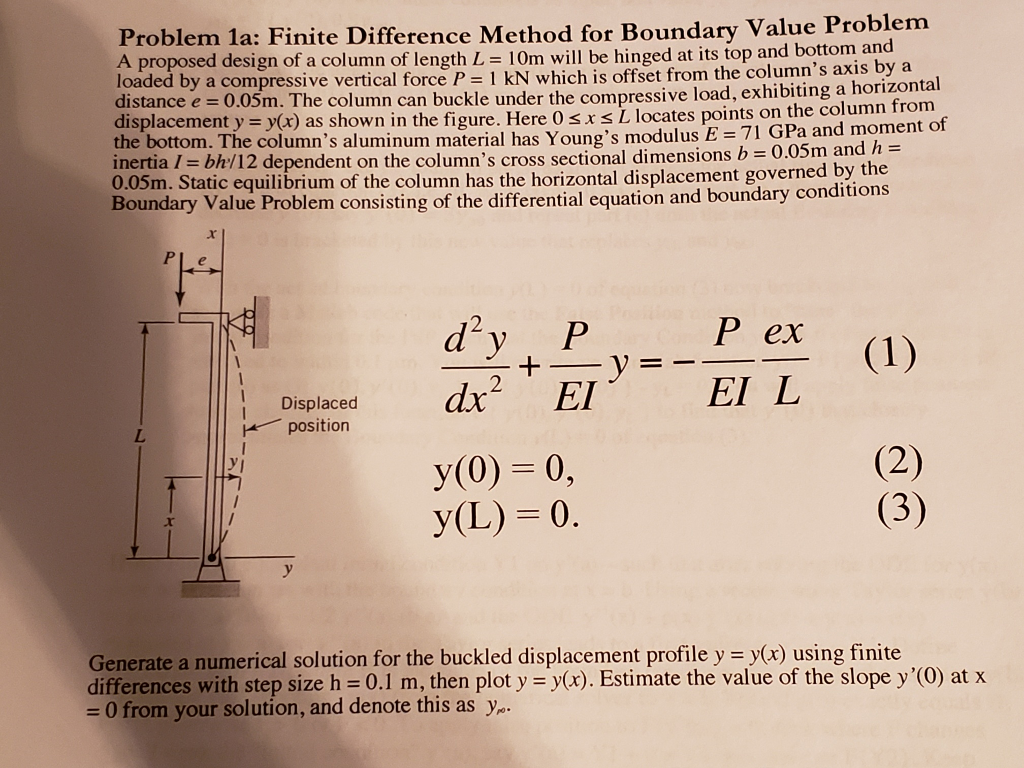
Problem 1a: Finite Difference Method for Boundary Value Problem A proposed design of a column of length L- 10m will be hinged at its top and bottom loaded by a compressive vertical force P 1 kN which is offset from the column's axis by a distance e 0.05m. The column can buckle under the com displacement y =y(x) as shown in the figure. Here oss L locates points on the column from the bottom. The column's aluminum material has Young's modulus E inertia bh/12 dependent on the column's 0.05m. Static equili Boundary Value Problem consisting of the differential equation and boundary conditions and pressive load, exhibiting a horizontal 71 GPa and moment cross sectional dimensions b = 0.05m and h = brium of the column has the horizontal displacement governed by the 2 Pex EI I ?+Elys y(0) = 0, y(L) 0. Displaceddx 2 L (1 position Generate a numerical solution for the buckled displacement profile y - y(x) using finite differences with step size h - 0.1 m, then plot y yx). Estimate the value of the slope y'(O) at x = 0 from your solution, and denote this as yo. alue of the slope y'(0) at x Problem 1a: Finite Difference Method for Boundary Value Problem A proposed design of a column of length L- 10m will be hinged at its top and bottom loaded by a compressive vertical force P 1 kN which is offset from the column's axis by a distance e 0.05m. The column can buckle under the com displacement y =y(x) as shown in the figure. Here oss L locates points on the column from the bottom. The column's aluminum material has Young's modulus E inertia bh/12 dependent on the column's 0.05m. Static equili Boundary Value Problem consisting of the differential equation and boundary conditions and pressive load, exhibiting a horizontal 71 GPa and moment cross sectional dimensions b = 0.05m and h = brium of the column has the horizontal displacement governed by the 2 Pex EI I ?+Elys y(0) = 0, y(L) 0. Displaceddx 2 L (1 position Generate a numerical solution for the buckled displacement profile y - y(x) using finite differences with step size h - 0.1 m, then plot y yx). Estimate the value of the slope y'(O) at x = 0 from your solution, and denote this as yo. alue of the slope y'(0) at x