PLEASE SOLVE USING R PROGRAMMING LANGUAGE

PLEASE SOLVE USING R PROGRAMMING LANGUAGE

PLEASE SOLVE USING R PROGRAMMING LANGUAGE

PLEASE SOLVE USING R PROGRAMMING LANGUAGE

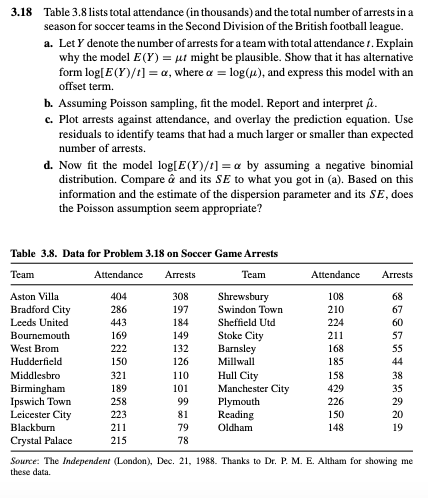
3.18 Table 3.8 lists total attendance (in thousands) and the total number of arrests in a season for soccer teams in the Second Division of the British football league. a. Let Y denote the number of arrests for a team with total attendance t. Explain why the model E(Y) t might be plausible. Show that it has alternative form log[E(Y)/1]-, where -log(), and express this model with an offset term. b. Assuming Poisson sampling, fit the model. Report and interpret c. Plot arrests against attendance, and overlay the prediction equation. Use residuals to identify teams that had a much larger or smaller than expected number of arrests. d. Now fit the model log[E(Y)/-a by assuming a negative binomial distribution. Compare and its SE to what you got in (a). Based on this information and the estimate of the dispersion parameter and its SE, does the Poisson assumption seem a ppropriate? Table 3.8. Data for Problem 3.18 on Soccer Game Arrests Team Attendance Arrests Team Attendance Arrests 08 Shrewsbury 197 184 Sheffield Utd 149 Soke City 132 Bamsley 126 110 Hull City 101 Manchester City 99 Plymouth 81 Reading Aston Villa Bradford City Leeds United 286 443 169 108 210 224 211 168 68 67 60 57 Swindon Town West Brom 150 321 Millwall 38 Birmingham Ipswich Town Leicester City Blackbun Crystal Palace 429 226 150 148 258 223 19 Source: The Independent London), Dec. 21. 1988. Thanks to Dr. P. M. E. Altham for showing me these data. 3.18 Table 3.8 lists total attendance (in thousands) and the total number of arrests in a season for soccer teams in the Second Division of the British football league. a. Let Y denote the number of arrests for a team with total attendance t. Explain why the model E(Y) t might be plausible. Show that it has alternative form log[E(Y)/1]-, where -log(), and express this model with an offset term. b. Assuming Poisson sampling, fit the model. Report and interpret c. Plot arrests against attendance, and overlay the prediction equation. Use residuals to identify teams that had a much larger or smaller than expected number of arrests. d. Now fit the model log[E(Y)/-a by assuming a negative binomial distribution. Compare and its SE to what you got in (a). Based on this information and the estimate of the dispersion parameter and its SE, does the Poisson assumption seem a ppropriate? Table 3.8. Data for Problem 3.18 on Soccer Game Arrests Team Attendance Arrests Team Attendance Arrests 08 Shrewsbury 197 184 Sheffield Utd 149 Soke City 132 Bamsley 126 110 Hull City 101 Manchester City 99 Plymouth 81 Reading Aston Villa Bradford City Leeds United 286 443 169 108 210 224 211 168 68 67 60 57 Swindon Town West Brom 150 321 Millwall 38 Birmingham Ipswich Town Leicester City Blackbun Crystal Palace 429 226 150 148 258 223 19 Source: The Independent London), Dec. 21. 1988. Thanks to Dr. P. M. E. Altham for showing me these data