please use C++ for the code

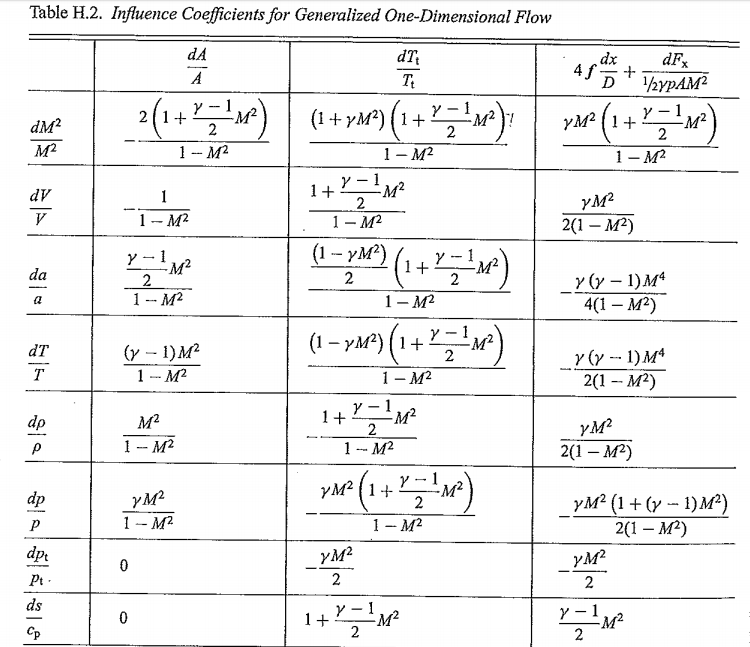
generalized one-dimensional flow method derived in many gas dynamics texts. It is a differential equation analysis from which influence coefficients are derived. These coefficients relate changes of one variable on another. You were provided with a handout showing a table of the influence coefficients. You will now use that table along with your lecture notes to solve a combined heat addition and friction problem for an aircraft engine combustion chamber Flow with an incoming Mach number of 0.4 and an inlet total pressure of 1.5 MPa enters a combustion chamber 0.75 m long with an inlet diameter of 0.4 m, which increases linearly to 0.6 m, and a friction factor of 0.045. The total temperature increases linearly from 700 K to 1700 K. What is the total pressure ratio across the burner if the specific heat ratio is 1.32? Also determine the burner ratios for static temperature and pressure, and the exit Mach number. On a single graph, plot the changes of these ratios and the Mach number as a function of axial position in the chamber (for incoming Mach number of 0.4 only) Now investigate the effects of these ratios with different incoming Mach numbers. Vary the Mach number from 0 to 0.9 in increments of 0.1. On a single graph, plot each variable ratio (total pressure, static pressure. static temperature) and the exit Mach number against the incoming Mach number. Do not plot non-physical results, but instead explain why these results are non-physical For both graphs, you will want to use a double-Y-axis plot, with the exit Mach number and pressure ratios on one axis, and the temperature ratio on the other axis To solve this problem, you will need to numerically integrate the equations you develop from the table of influence coefficients. This is relatively easy if you replace all the differential terms (dM, dl etc.) with delta terms (M. ??. etc.) where a delta term represents a change in the variable in space. Divide the chamber into 100 increments, and use a backwards difference for each delta term (i.e. ??_MPi-MPi-1) All terms on the right-hand side of the equations should be evaluated at the i - 1 node. In this fashion, you can numerically integrate your way across the burner generalized one-dimensional flow method derived in many gas dynamics texts. It is a differential equation analysis from which influence coefficients are derived. These coefficients relate changes of one variable on another. You were provided with a handout showing a table of the influence coefficients. You will now use that table along with your lecture notes to solve a combined heat addition and friction problem for an aircraft engine combustion chamber Flow with an incoming Mach number of 0.4 and an inlet total pressure of 1.5 MPa enters a combustion chamber 0.75 m long with an inlet diameter of 0.4 m, which increases linearly to 0.6 m, and a friction factor of 0.045. The total temperature increases linearly from 700 K to 1700 K. What is the total pressure ratio across the burner if the specific heat ratio is 1.32? Also determine the burner ratios for static temperature and pressure, and the exit Mach number. On a single graph, plot the changes of these ratios and the Mach number as a function of axial position in the chamber (for incoming Mach number of 0.4 only) Now investigate the effects of these ratios with different incoming Mach numbers. Vary the Mach number from 0 to 0.9 in increments of 0.1. On a single graph, plot each variable ratio (total pressure, static pressure. static temperature) and the exit Mach number against the incoming Mach number. Do not plot non-physical results, but instead explain why these results are non-physical For both graphs, you will want to use a double-Y-axis plot, with the exit Mach number and pressure ratios on one axis, and the temperature ratio on the other axis To solve this problem, you will need to numerically integrate the equations you develop from the table of influence coefficients. This is relatively easy if you replace all the differential terms (dM, dl etc.) with delta terms (M. ??. etc.) where a delta term represents a change in the variable in space. Divide the chamber into 100 increments, and use a backwards difference for each delta term (i.e. ??_MPi-MPi-1) All terms on the right-hand side of the equations should be evaluated at the i - 1 node. In this fashion, you can numerically integrate your way across the burner








