plz write neatly and circle u answer only answer to red!!! Don't just copy other Chegg answer!!!

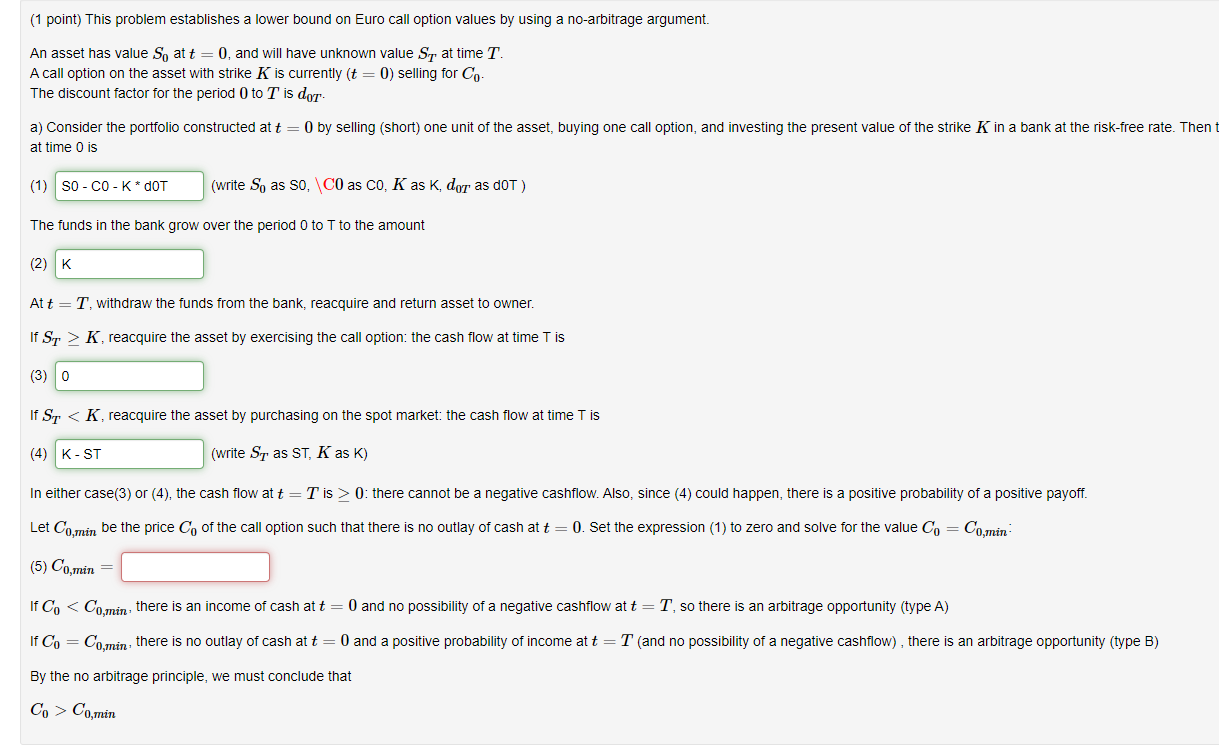
(1 point) This problem establishes a lower bound on Euro call option values by using a no-arbitrage argument. An asset has value So at t = 0, and will have unknown value St at time T. A call option on the asset with strike K is currently (t = 0) selling for Co The discount factor for the period 0 to T is dor a) Consider the portfolio constructed at t = 0 by selling (short) one unit of the asset, buying one call option, and investing the present value of the strike K in a bank at the risk-free rate. Thent at time 0 is (1) SO-CO-K * DOT (write So as so, \CO as Co, K as K, dor as dor) The funds in the bank grow over the period 0 to T to the amount (2) K Att T, withdraw the funds from the bank, reacquire and return asset to owner. If ST > K, reacquire the asset by exercising the call option: the cash flow at time T is (3) 0 If St 0: there cannot be a negative cashflow. Also, since (4) could happen, there is a positive probability of a positive payoff. Let Comin be the price Co of the call option such that there is no outlay of cash at t = 0. Set the expression (1) to zero and solve for the value Co = Comin (5) Comin If C, Comin (1 point) This problem establishes a lower bound on Euro call option values by using a no-arbitrage argument. An asset has value So at t = 0, and will have unknown value St at time T. A call option on the asset with strike K is currently (t = 0) selling for Co The discount factor for the period 0 to T is dor a) Consider the portfolio constructed at t = 0 by selling (short) one unit of the asset, buying one call option, and investing the present value of the strike K in a bank at the risk-free rate. Thent at time 0 is (1) SO-CO-K * DOT (write So as so, \CO as Co, K as K, dor as dor) The funds in the bank grow over the period 0 to T to the amount (2) K Att T, withdraw the funds from the bank, reacquire and return asset to owner. If ST > K, reacquire the asset by exercising the call option: the cash flow at time T is (3) 0 If St 0: there cannot be a negative cashflow. Also, since (4) could happen, there is a positive probability of a positive payoff. Let Comin be the price Co of the call option such that there is no outlay of cash at t = 0. Set the expression (1) to zero and solve for the value Co = Comin (5) Comin If C, Comin