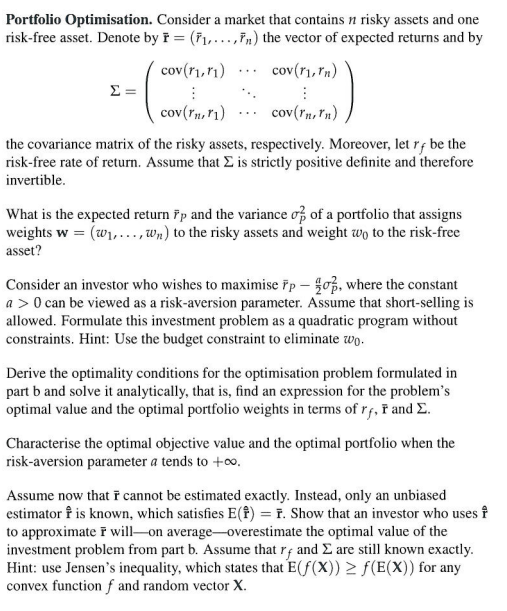
Portfolio Optimisation. Consider a market that contains n risky assets and one risk-free asset. Denote by i = (1, ..,in the vector of expected returns and by cov(r1,11) ... cov(r1, in) cov(Inn) ... cov(Inern) the covariance matrix of the risky assets, respectively. Moreover, letr, be the risk-free rate of return. Assume that is strictly positive definite and therefore invertible. What is the expected return ip and the variance of a portfolio that assigns weights W = (W1,...,wn) to the risky assets and weight wo to the risk-free asset? Consider an investor who wishes to maximise ip - 90, where the constant a> 0 can be viewed as a risk-aversion parameter. Assume that short-selling is allowed. Formulate this investment problem as a quadratic program without constraints. Hint: Use the budget constraint to eliminate wo. Derive the optimality conditions for the optimisation problem formulated in part b and solve it analytically, that is, find an expression for the problem's optimal value and the optimal portfolio weights in terms of rf, P and Characterise the optimal objective value and the optimal portfolio when the risk-aversion parameter a tends to +0. Assume now that cannot be estimated exactly. Instead, only an unbiased estimator P is known, which satisfies E(f) = 1. Show that an investor who uses f to approximate I will on average-overestimate the optimal value of the investment problem from part b. Assume that rf and are still known exactly Hint: use Jensen's inequality, which states that E(F(X)) = f(E(X)) for any convex function f and random vector X. Portfolio Optimisation. Consider a market that contains n risky assets and one risk-free asset. Denote by i = (1, ..,in the vector of expected returns and by cov(r1,11) ... cov(r1, in) cov(Inn) ... cov(Inern) the covariance matrix of the risky assets, respectively. Moreover, letr, be the risk-free rate of return. Assume that is strictly positive definite and therefore invertible. What is the expected return ip and the variance of a portfolio that assigns weights W = (W1,...,wn) to the risky assets and weight wo to the risk-free asset? Consider an investor who wishes to maximise ip - 90, where the constant a> 0 can be viewed as a risk-aversion parameter. Assume that short-selling is allowed. Formulate this investment problem as a quadratic program without constraints. Hint: Use the budget constraint to eliminate wo. Derive the optimality conditions for the optimisation problem formulated in part b and solve it analytically, that is, find an expression for the problem's optimal value and the optimal portfolio weights in terms of rf, P and Characterise the optimal objective value and the optimal portfolio when the risk-aversion parameter a tends to +0. Assume now that cannot be estimated exactly. Instead, only an unbiased estimator P is known, which satisfies E(f) = 1. Show that an investor who uses f to approximate I will on average-overestimate the optimal value of the investment problem from part b. Assume that rf and are still known exactly Hint: use Jensen's inequality, which states that E(F(X)) = f(E(X)) for any convex function f and random vector X







