Power Method and Inverse Iteration

(a) Implement the Power Method for an arbitrary matrix A ? Rⁿⁿ and an initial vector x₀ ? Rⁿ .

(b) Use your code to find an eigenvector of

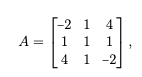
starting with x₀ = (1, 2, −1)^T and x₀ = (1, 2, 1)^T . Report the first 5 iterates for each of the two initial vectors. Then use MATLAB's eig(A) to examine the eigenvalues and eigenvectors of A. Where do the sequences converge to? Why do the limits not seem to be the same?

(c) Implement the Inverse Power Method for an arbitrary matrix A ∈ R^n×n, an initial vector x₀ ∈ Rⁿ and an initial eigenvalue guess θ ∈ R. 

(d) Use your code from (c) to calculate all eigenvectors of A. You may pick appropriate values for θ and the initial vector as you wish (obviously not the eigenvectors themselves). Always report the first 5 iterates and explain where the sequence converges to and why. 

Please also hand in your code.

A = -2 1 4 1 1 1 4 1 -2]