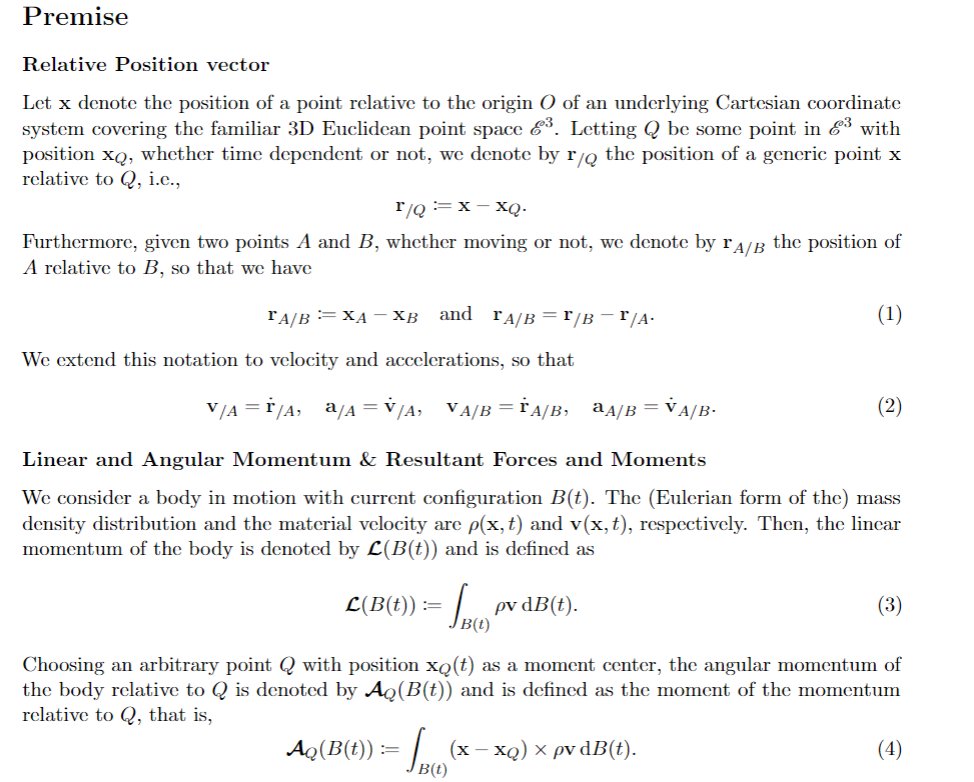
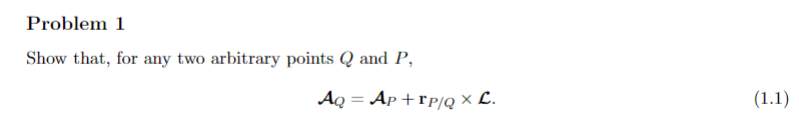
Premise Relative Position vector Let x denote the position of a point relative to the origin O of an underlying Cartesian coordinate system covering the familiar 3D Euclidean point space &. Letting Q be some point in with position xq, whether time dependent or not, we denote by r/o the position of a generic point x relative to Q, i.c., r/Q == x-xQ. Furthermore, given two points A and B, whether moving or not, we denote by F/B the position of A relative to B, so that we have TA/B=XA-XB and rA/B=1/B /A- We extend this notation to velocity and accelerations, so that V/A=/A_/A=V/A, VA/B=A/B,_A/B=A/B- c(B(t)) = p(1) B(t) Linear and Angular Momentum & Resultant Forces and Moments We consider a body in motion with current configuration B(t). The (Eulerian form of the) mass density distribution and the material velocity are p(x, t) and v(x, t), respectively. Then, the linear momentum of the body is denoted by L(B(t)) and is defined as pv dB(t). Aq(B(t)) = (x (1) (2) (x xq) pv dB(t). Choosing an arbitrary point Q with position xo(t) as a moment center, the angular momentum of the body relative to Q is denoted by Ao(B(t)) and is defined as the moment of the momentum relative to Q, that is, (3) Note that, using the notation introduced above, A(B(t)) can be written as Aq = B(0 (5) We assume that B(t) moves under the action of a surface traction distribution s(x, t) and an external body force field bo(x, t). Then, the resultant of the external forces acting on B(t) is denoted by F(B(t)) and is defined as T/QXp r/Q x pv dB(t). F(B(t)) = bodB(t) + (6) B(t) Using the notation introduced above, and choosing a point Q with position xo(t) as moment center, the resultant moment of all the external forces computed relative to Q is denoted by Mo (B(t)) and is defined as MQ(B(t)) = r/Q x bo dB(t) + B(t) -JAR xG(t) = = 1 B(0) B Aspin (t) = B(t) Center of Mass and Spin Angular Momentum The center of mass of B(t) is the point G whose position relative to the origin O is Love sd(@B(t)). r/qxsd(@B(t)). px dB(t). Given the above definition, one can show that the linear momentum of the body can be expressed as L(B(t)) = mvg(t) with vo(t) = xg(t), (9) (7) where and vg(t) is the velocity of G. We denote the spin angular momentum by Aspin (t) and we define it as the moment relative to G of the momentum relative to G,* i.e., (8) r/GX pv/G dB(t). (10) Problem 1 Show that, for any two arbitrary points Q and P, Aq = Ap+rP/Q X L. (1.1)