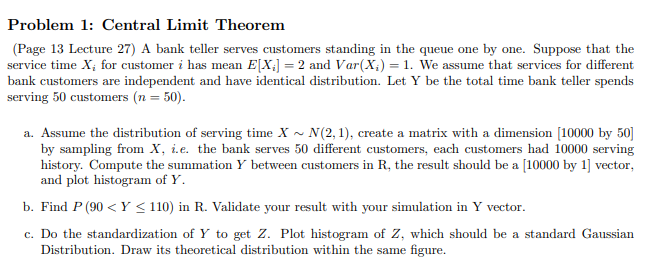
Problem 1: Central Limit Theorem (Page 13 Lecture 27) A bank teller serves customers standing in the queue one by one. Suppose that the service time X; for customer i has mean E[X] = 2 and Var(X;) = 1. We assume that services for different bank customers are independent and have identical distribution. Let Y be the total time bank teller spends serving 50 customers (n = 50). a. Assume the distribution of serving time X ~N(2, 1), create a matrix with a dimension [10000 by 50] by sampling from X, i.e. the bank serves 50 different customers, each customers had 10000 serving history. Compute the summation Y between customers in R, the result should be a [10000 by 1] vector, and plot histogram of Y. b. Find P (90