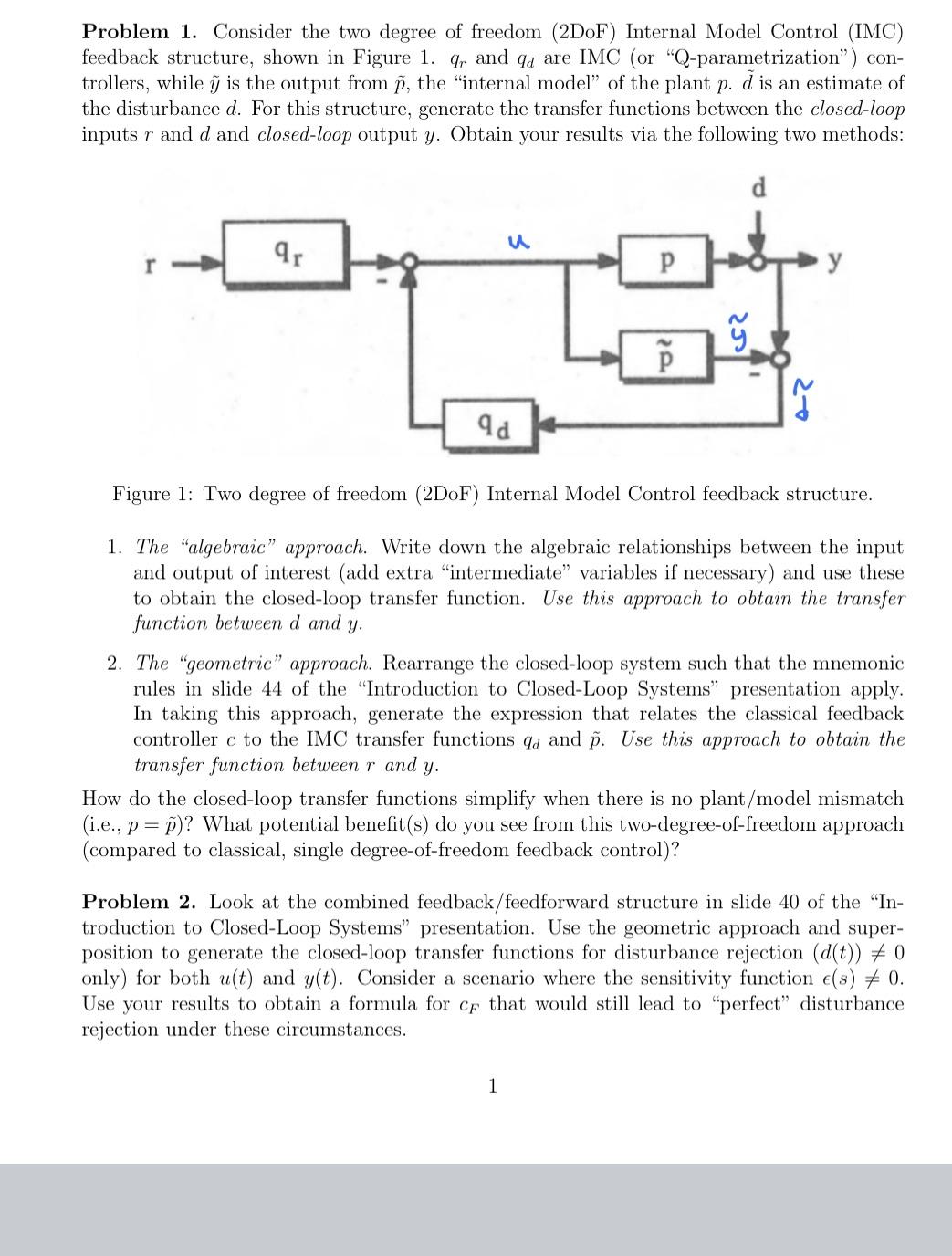
Problem 1. Consider the two degree of freedom (2DOF) Internal Model Control (IMC) feedback structure, shown in Figure 1. qr and qd are IMC (or "Q-parametrization") con- trollers, while y is the output from p, the "internal model" of the plant p. d is an estimate of the disturbance d. For this structure, generate the transfer functions between the closed-loop inputs r and d and closed-loop output y. Obtain your results via the following two methods: qr r y. d ad Figure 1: Two degree of freedom (2DOF) Internal Model Control feedback structure. 1. The "algebraic" approach. Write down the algebraic relationships between the input and output of interest (add extra "intermediate" variables if necessary) and use these to obtain the closed-loop transfer function. Use this approach to obtain the transfer function between d and y. 2. The "geometric" approach. Rearrange the closed-loop system such that the mnemonic rules in slide 44 of the "Introduction to Closed-Loop Systems" presentation apply. In taking this approach, generate the expression that relates the classical feedback controller c to the IMC transfer functions qa and p. Use this approach to obtain the transfer function between r and y. How do the closed-loop transfer functions simplify when there is no plant/model mismatch (i.e., pp)? What potential benefit(s) do you see from this two-degree-of-freedom approach (compared to classical, single degree-of-freedom feedback control)? Problem 2. Look at the combined feedback/feedforward structure in slide 40 of the "In- troduction to Closed-Loop Systems" presentation. Use the geometric approach and super- position to generate the closed-loop transfer functions for disturbance rejection (d(t)) # 0 only) for both u(t) and y(t). Consider a scenario where the sensitivity function e(s) 0. Use your results to obtain a formula for cp that would still lead to "perfect" disturbance rejection under these circumstances. 1